Question Number 9675 by ridwan balatif last updated on 23/Dec/16

Commented by ridwan balatif last updated on 23/Dec/16
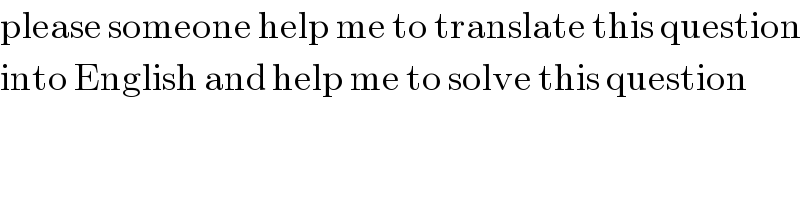
$$\mathrm{please}\:\mathrm{someone}\:\mathrm{help}\:\mathrm{me}\:\mathrm{to}\:\mathrm{translate}\:\mathrm{this}\:\mathrm{question} \\ $$$$\mathrm{into}\:\mathrm{English}\:\mathrm{and}\:\mathrm{help}\:\mathrm{me}\:\mathrm{to}\:\mathrm{solve}\:\mathrm{this}\:\mathrm{question} \\ $$
Commented by sandy_suhendra last updated on 23/Dec/16
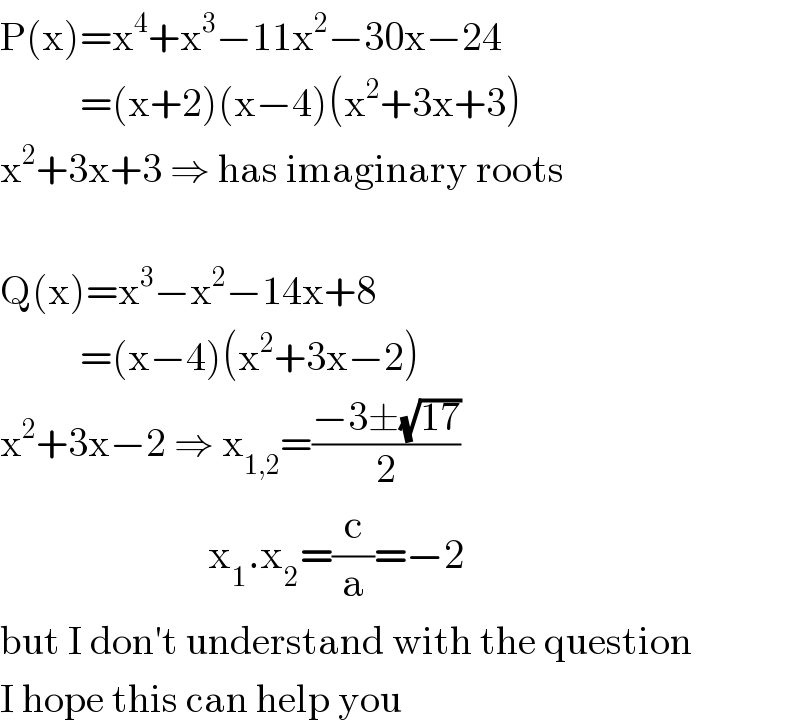
$$\mathrm{P}\left(\mathrm{x}\right)=\mathrm{x}^{\mathrm{4}} +\mathrm{x}^{\mathrm{3}} −\mathrm{11x}^{\mathrm{2}} −\mathrm{30x}−\mathrm{24} \\ $$$$\:\:\:\:\:\:\:\:\:\:=\left(\mathrm{x}+\mathrm{2}\right)\left(\mathrm{x}−\mathrm{4}\right)\left(\mathrm{x}^{\mathrm{2}} +\mathrm{3x}+\mathrm{3}\right) \\ $$$$\mathrm{x}^{\mathrm{2}} +\mathrm{3x}+\mathrm{3}\:\Rightarrow\:\mathrm{has}\:\mathrm{imaginary}\:\mathrm{roots} \\ $$$$ \\ $$$$\mathrm{Q}\left(\mathrm{x}\right)=\mathrm{x}^{\mathrm{3}} −\mathrm{x}^{\mathrm{2}} −\mathrm{14x}+\mathrm{8} \\ $$$$\:\:\:\:\:\:\:\:\:\:=\left(\mathrm{x}−\mathrm{4}\right)\left(\mathrm{x}^{\mathrm{2}} +\mathrm{3x}−\mathrm{2}\right) \\ $$$$\mathrm{x}^{\mathrm{2}} +\mathrm{3x}−\mathrm{2}\:\Rightarrow\:\mathrm{x}_{\mathrm{1},\mathrm{2}} =\frac{−\mathrm{3}\pm\sqrt{\mathrm{17}}}{\mathrm{2}}\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{x}_{\mathrm{1}} .\mathrm{x}_{\mathrm{2}} =\frac{\mathrm{c}}{\mathrm{a}}=−\mathrm{2} \\ $$$$\mathrm{but}\:\mathrm{I}\:\mathrm{don}'\mathrm{t}\:\mathrm{understand}\:\mathrm{with}\:\mathrm{the}\:\mathrm{question} \\ $$$$\mathrm{I}\:\mathrm{hope}\:\mathrm{this}\:\mathrm{can}\:\mathrm{help}\:\mathrm{you} \\ $$
