Question Number 11146 by suci last updated on 14/Mar/17
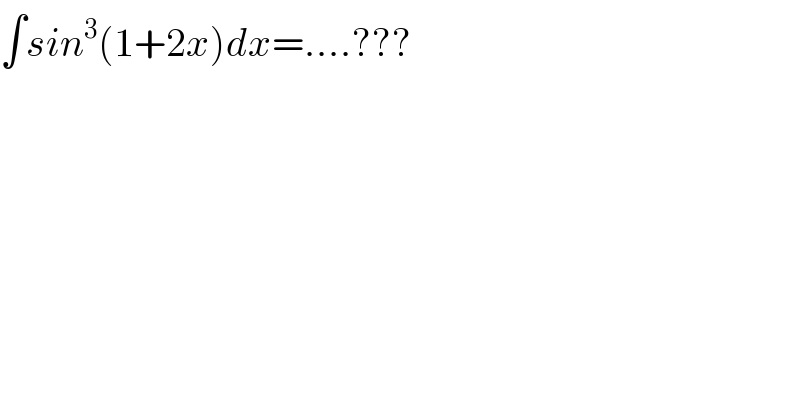
$$\int{sin}^{\mathrm{3}} \left(\mathrm{1}+\mathrm{2}{x}\right){dx}=….??? \\ $$
Answered by ajfour last updated on 14/Mar/17
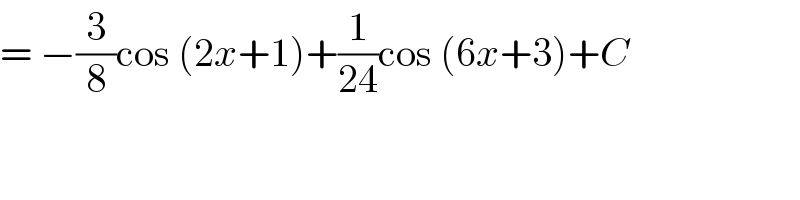
$$=\:−\frac{\mathrm{3}}{\mathrm{8}}\mathrm{cos}\:\left(\mathrm{2}{x}+\mathrm{1}\right)+\frac{\mathrm{1}}{\mathrm{24}}\mathrm{cos}\:\left(\mathrm{6}{x}+\mathrm{3}\right)+{C} \\ $$
Commented by ajfour last updated on 14/Mar/17
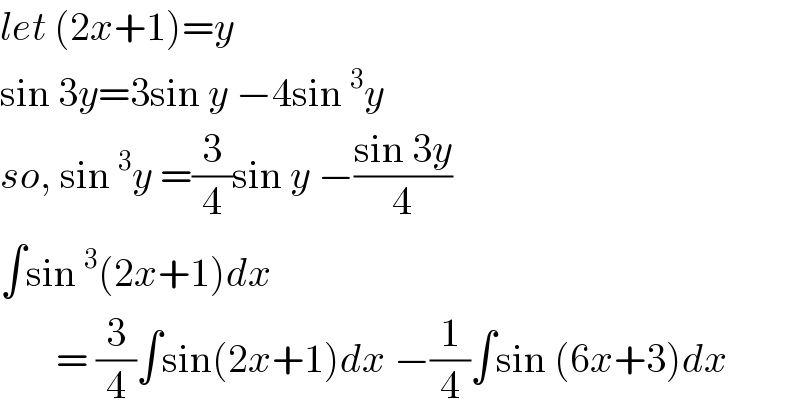
$${let}\:\left(\mathrm{2}{x}+\mathrm{1}\right)={y} \\ $$$$\mathrm{sin}\:\mathrm{3}{y}=\mathrm{3sin}\:{y}\:−\mathrm{4sin}\:^{\mathrm{3}} {y} \\ $$$${so},\:\mathrm{sin}\:^{\mathrm{3}} {y}\:=\frac{\mathrm{3}}{\mathrm{4}}\mathrm{sin}\:{y}\:−\frac{\mathrm{sin}\:\mathrm{3}{y}}{\mathrm{4}} \\ $$$$\int\mathrm{sin}\:^{\mathrm{3}} \left(\mathrm{2}{x}+\mathrm{1}\right){dx} \\ $$$$\:\:\:\:\:\:\:=\:\frac{\mathrm{3}}{\mathrm{4}}\int\mathrm{sin}\left(\mathrm{2}{x}+\mathrm{1}\right){dx}\:−\frac{\mathrm{1}}{\mathrm{4}}\int\mathrm{sin}\:\left(\mathrm{6}{x}+\mathrm{3}\right){dx} \\ $$
Answered by Mechas88 last updated on 17/Mar/17
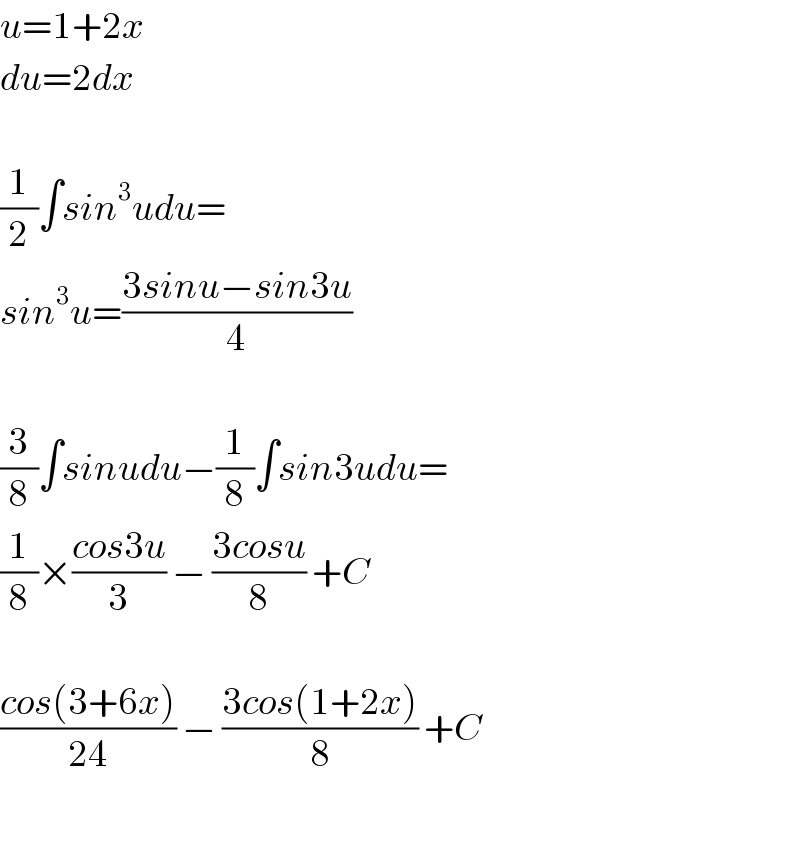
$${u}=\mathrm{1}+\mathrm{2}{x} \\ $$$${du}=\mathrm{2}{dx} \\ $$$$ \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\int{sin}^{\mathrm{3}} {udu}= \\ $$$${sin}^{\mathrm{3}} {u}=\frac{\mathrm{3}{sinu}−{sin}\mathrm{3}{u}}{\mathrm{4}} \\ $$$$ \\ $$$$\frac{\mathrm{3}}{\mathrm{8}}\int{sinudu}−\frac{\mathrm{1}}{\mathrm{8}}\int{sin}\mathrm{3}{udu}= \\ $$$$\frac{\mathrm{1}}{\mathrm{8}}×\frac{{cos}\mathrm{3}{u}}{\mathrm{3}}\:−\:\frac{\mathrm{3}{cosu}}{\mathrm{8}}\:+{C} \\ $$$$ \\ $$$$\frac{{cos}\left(\mathrm{3}+\mathrm{6}{x}\right)}{\mathrm{24}}\:−\:\frac{\mathrm{3}{cos}\left(\mathrm{1}+\mathrm{2}{x}\right)}{\mathrm{8}}\:+{C} \\ $$$$ \\ $$
