sinh-ln-x-1-x-2-A-2x-B-1-x-C-x-2-D-x-

Question Number 71235 by Rio Michael last updated on 13/Oct/19

s i n h [l n (x + \sqrt{1 + x^{2}})] \equiv

A . 2 x

B . \frac{1}{x}

C . x^{2}

D . x

Commented by mathmax by abdo last updated on 13/Oct/19

s h {l n (x + \sqrt{1 + x^{2}})} = \frac{e^{l n (x + \sqrt{1 + x^{2}})} - e^{- l n (x + \sqrt{1 + x^{2})}}}{2}

= \frac{x + \sqrt{1 + x^{2}} - \frac{1}{x + \sqrt{1 + x^{2}}}}{2} = \frac{{(x + \sqrt{1 + x^{2}})}^{2} - 1}{2 (x + \sqrt{1 + x^{2}})}

= \frac{x^{2} + 2 x \sqrt{1 + x^{2}} + 1 + x^{2} - 1}{2 (x + \sqrt{1 + x^{2})}} = \frac{2 x^{2} + 2 x \sqrt{1 + x^{2}}}{2 (x + \sqrt{1 + x^{2}})}

= \frac{x (x + \sqrt{1 + x^{2}}}{x + \sqrt{1 + x^{2}}} = x

a l s o w e c a n u s e t h a t l n (x + \sqrt{1 + x^{2}}) = a r g s h (x) = {s h}^{- 1} (x)

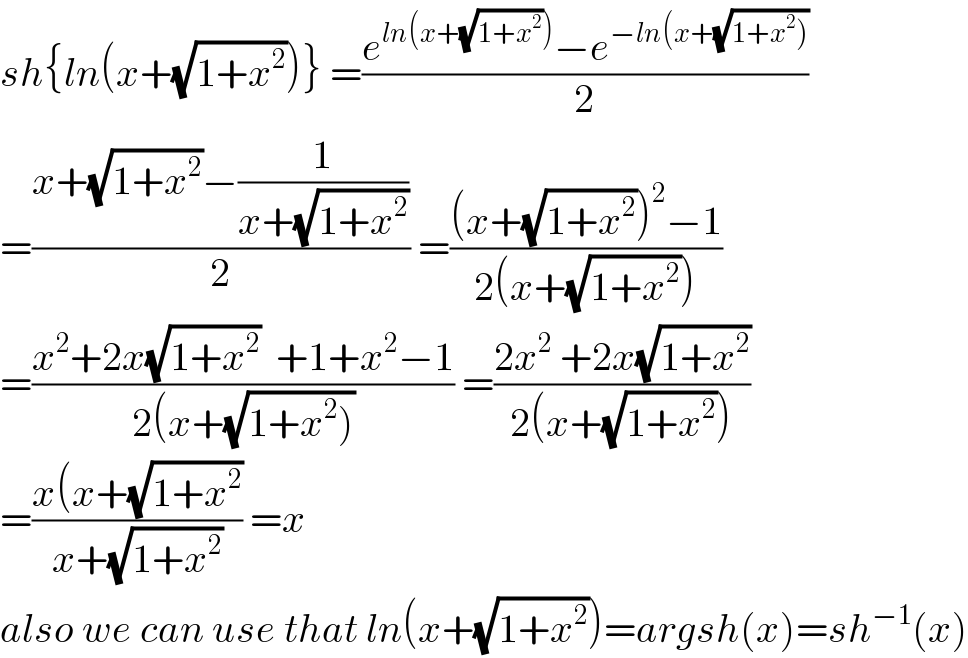
Answered by MJS last updated on 13/Oct/19

\sinh α = \frac{1}{2} (e^{α} - e^{- α})

\Rightarrow answer is D . x

Commented by Rio Michael last updated on 13/Oct/19

i {d o n}^{'} t u n d e r s t a n d t h e w o r k i n g s i r

Commented by MJS last updated on 13/Oct/19

first, sorry I made a typo in the formula

\sinh \ln (x + \sqrt{x^{2} + 1}) = \frac{1}{2} (x + \sqrt{x^{2} + 1} - \frac{1}{x + \sqrt{x^{2} + 1}}) =

= \frac{1}{2} \times \frac{{(x + \sqrt{x^{2} + 1})}^{2} - 1}{x + \sqrt{x^{2} + 1}} = \frac{1}{2} \times \frac{2 x^{2} + 2 x \sqrt{x^{2} + 1}}{x + \sqrt{x^{2} + 1}} = x

Commented by Rio Michael last updated on 13/Oct/19

t h a n k s s i r

Answered by mr W last updated on 13/Oct/19

l e t t = \ln (x + \sqrt{1 + x^{2}})

e^{t} = x + \sqrt{1 + x^{2}} \dots (i)

e^{- t} = \frac{1}{x + \sqrt{1 + x^{2}}} = - x + \sqrt{1 + x^{2}}

- e^{- t} = x - \sqrt{1 + x^{2}} \dots (i i)

e^{t} - e^{- t} = 2 x

\frac{e^{t} - e^{- t}}{2} = x

\Rightarrow \sinh t = x

i . e . \sinh [\ln (x + \sqrt{1 + x^{2}})] = x

\Rightarrow a n s w e r D

Commented by Rio Michael last updated on 13/Oct/19

t h a n k s s i r s