Question Number 4222 by Rasheed Soomro last updated on 02/Jan/16

Commented by prakash jain last updated on 03/Jan/16
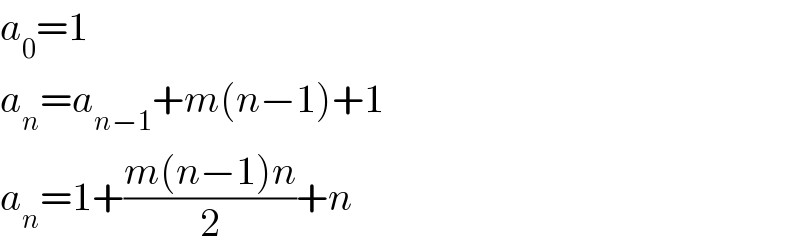
Commented by prakash jain last updated on 03/Jan/16
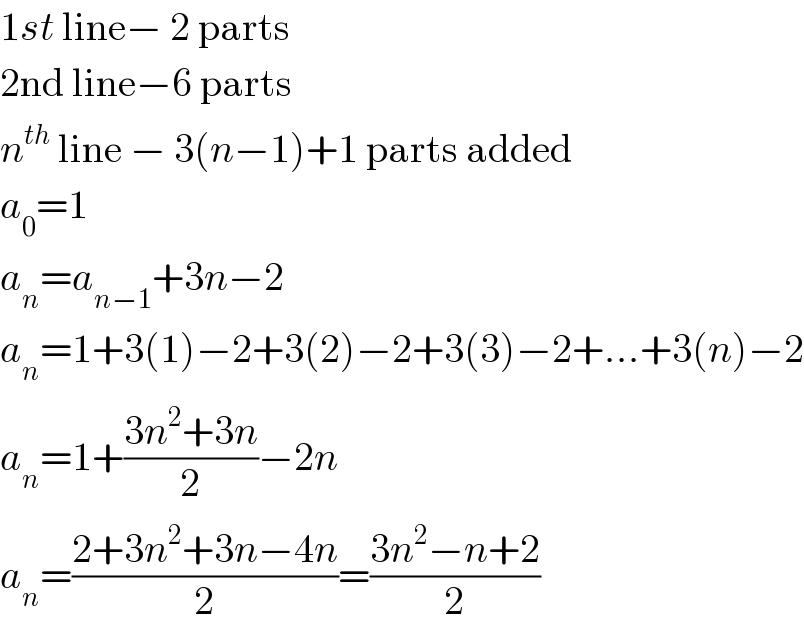
Commented by Rasheed Soomro last updated on 03/Jan/16
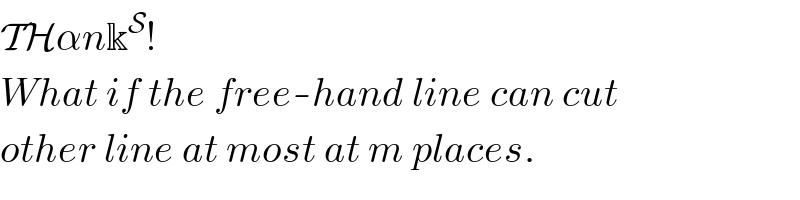
Commented by Rasheed Soomro last updated on 03/Jan/16

