Solve-2x-x-7-2-x-3-2-0-

Question Number 8071 by marcusvsoliveira last updated on 29/Sep/16

S o l v e - 2 x (x + \frac{7}{2}) - {(x - 3)}^{2} ⩽ 0

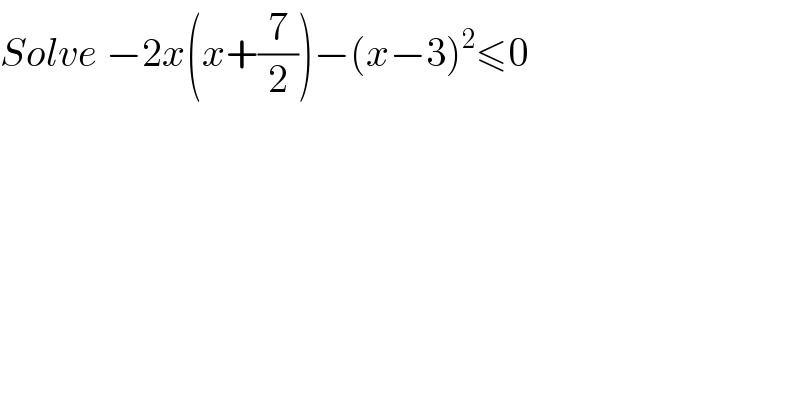
Commented by FilupSmith last updated on 29/Sep/16

- 2 x (x + \frac{7}{2}) - {(x - 3)}^{2} = - (2 x^{2} + 7 x) - (x^{2} - 6 x + 9)

= - 2 x^{2} - x^{2} - 7 x - 6 x - 9

= - 3 x^{2} - 13 x - 9 ⩽ 0

∴ 3 x^{2} + 13 x + 9 ⩾ 0

x = \frac{- 13 \pm \sqrt{13^{2} - 4 (3) (9)}}{6} = \frac{- 13 \pm \sqrt{61}}{6}

w o r k i n g

Commented by marcusvsoliveira last updated on 29/Sep/16

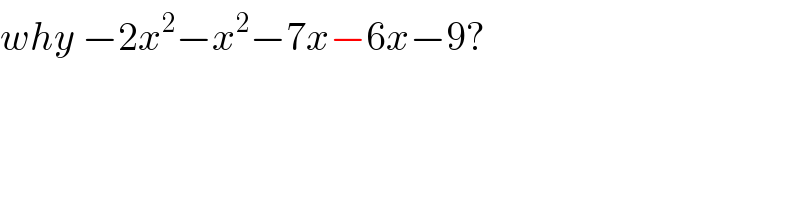
w h y - 2 x^{2} - x^{2} - 7 x - 6 x - 9 ?

Answered by prakash jain last updated on 29/Sep/16

- 2 x (x + \frac{7}{2}) - {(x - 3)}^{2} ⩽ 0

- 2 x^{2} - 7 x - x^{2} + 6 x - 9 ⩽ 0

- 3 x^{2} - x - 9 ⩽ 0

- 3 x^{2} - x - 9 ⩽ 0

r o o t a r e \frac{1 \pm \sqrt{1 - 27 \times 4}}{- 6} \Rightarrow n o r e a l r o o t

as the quadratic has no real roots it

takes the same as coefficient of x^{2} for x \in R .

- 3 x^{2} - x - 9 ⩽ 0 for x \in R

Solution set R

Commented by FilupSmith last updated on 30/Sep/16

I made a silly error . no wonder i couldnt

figure it out