Menu Close

solve-for-x-y-z-N-35x-21y-60z-665-

Tinku Tara 0 Comments
Pin




Question Number 77965 by mr W last updated on 12/Jan/20
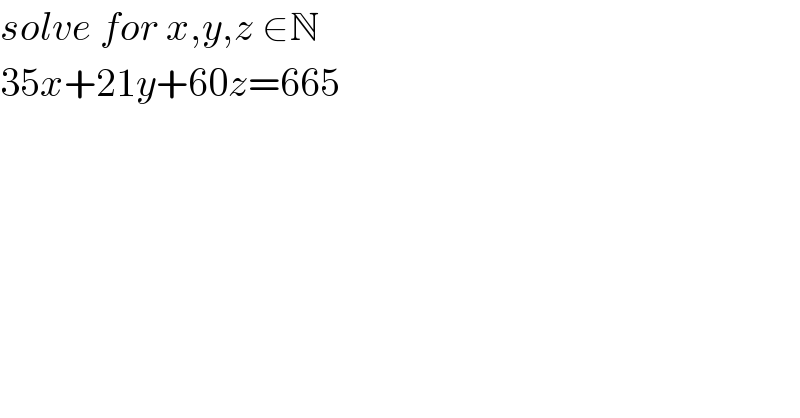
solve for x,y,z ∈N 35x+21y+60z=665
$${solve}\:{for}\:{x},{y},{z}\:\in\mathbb{N} \\ $$$$\mathrm{35}{x}+\mathrm{21}{y}+\mathrm{60}{z}=\mathrm{665} \\ $$
Commented by john santu last updated on 12/Jan/20
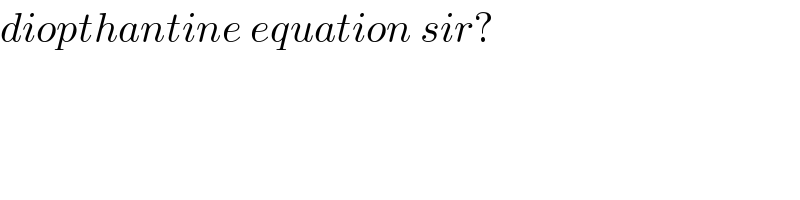
diopthantine equation sir?
$${diopthantine}\:{equation}\:{sir}? \\ $$
Commented by mr W last updated on 12/Jan/20
yes
$${yes} \\ $$
Commented by TawaTawa last updated on 12/Jan/20
35x + 3(7y + 20z) = 665 Let u = 7y + 20z 35x + 3u = 665 ...... (ii) Particular solution. x_1 = 1, u_1 = 210 General solution for (ii) ∴ x = 1 + 3m 35(1 + 3m) + 3u = 665 ..... (ii) ∴ 35 + 105m + 3u = 665 ∴ 3u = 665 − 35 − 105m ∴ 3u = 630 − 105m ∴ u = 210 − 35m (with m be any integer) Particular solution for 7y + 20z = 1 (y_1 = 3, z_1 = − 1) ∴ y = 3 + 20k ∴ 7(3 + 20k) + 20z = 1, 21 + 140k + 20z = 1 ∴ 20z = 1 − 21 − 140k ∴ 20z = − 20 − 140k, z = − 1 − 7k General solution of 35x + 21y + 60z = 665 y = (3 + 20k)u = 3u + 20uk = 3u + 20n y = 3(210 − 35m) + 20n = 630 − 105m + 20n z = (− 1 − 7k)u = − u − 7uk = − u − 7n z = − (210 − 35m) − 7n = − 210 + 35m − 7n Therefore, x = 1 + 3m y = 630 − 105m + 20n z = − 210 + 35m − 7n m, n be any integer
$$\mathrm{35x}\:+\:\mathrm{3}\left(\mathrm{7y}\:+\:\mathrm{20z}\right)\:\:=\:\:\mathrm{665} \\ $$$$\mathrm{Let}\:\:\mathrm{u}\:\:=\:\:\mathrm{7y}\:+\:\mathrm{20z} \\ $$$$\mathrm{35x}\:+\:\mathrm{3u}\:\:=\:\:\mathrm{665}\:\:\:\:\:\:……\:\left(\mathrm{ii}\right) \\ $$$$\mathrm{Particular}\:\mathrm{solution}.\:\:\:\:\mathrm{x}_{\mathrm{1}} \:\:=\:\:\mathrm{1},\:\:\:\:\mathrm{u}_{\mathrm{1}} \:=\:\mathrm{210} \\ $$$$\mathrm{General}\:\mathrm{solution}\:\mathrm{for}\:\:\left(\mathrm{ii}\right) \\ $$$$\therefore\:\:\:\:\:\:\:\:\:\mathrm{x}\:\:=\:\:\mathrm{1}\:+\:\mathrm{3m} \\ $$$$\mathrm{35}\left(\mathrm{1}\:+\:\mathrm{3m}\right)\:+\:\mathrm{3u}\:\:=\:\:\mathrm{665}\:\:\:\:\:\:…..\:\left(\mathrm{ii}\right) \\ $$$$\therefore\:\:\:\:\:\:\:\mathrm{35}\:+\:\mathrm{105m}\:+\:\mathrm{3u}\:\:=\:\:\mathrm{665} \\ $$$$\therefore\:\:\:\:\:\:\:\mathrm{3u}\:\:=\:\:\mathrm{665}\:\:−\:\mathrm{35}\:−\:\mathrm{105m} \\ $$$$\therefore\:\:\:\:\:\:\:\mathrm{3u}\:\:=\:\:\mathrm{630}\:\:−\:\mathrm{105m} \\ $$$$\therefore\:\:\:\:\:\:\:\:\:\:\:\mathrm{u}\:\:=\:\:\mathrm{210}\:\:−\:\mathrm{35m}\:\:\:\:\left(\mathrm{with}\:\:\mathrm{m}\:\:\mathrm{be}\:\mathrm{any}\:\mathrm{integer}\right) \\ $$$$\mathrm{Particular}\:\mathrm{solution}\:\mathrm{for} \\ $$$$\:\:\:\:\:\:\:\mathrm{7y}\:+\:\mathrm{20z}\:\:=\:\:\mathrm{1}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{y}_{\mathrm{1}} \:\:=\:\:\:\mathrm{3},\:\:\:\:\:\mathrm{z}_{\mathrm{1}} \:\:=\:\:−\:\mathrm{1}\right) \\ $$$$\therefore\:\:\:\:\:\:\:\mathrm{y}\:\:\:=\:\:\:\mathrm{3}\:+\:\mathrm{20k} \\ $$$$\therefore\:\:\:\:\mathrm{7}\left(\mathrm{3}\:+\:\mathrm{20k}\right)\:+\:\mathrm{20z}\:\:=\:\:\mathrm{1},\:\:\:\:\:\:\:\:\:\:\mathrm{21}\:+\:\mathrm{140k}\:+\:\mathrm{20z}\:\:=\:\:\mathrm{1} \\ $$$$\therefore\:\:\:\:\:\mathrm{20z}\:\:=\:\:\mathrm{1}\:−\:\mathrm{21}\:−\:\mathrm{140k} \\ $$$$\therefore\:\:\:\:\:\mathrm{20z}\:\:=\:\:−\:\mathrm{20}\:−\:\mathrm{140k},\:\:\:\:\:\:\:\:\:\:\:\mathrm{z}\:\:\:=\:\:−\:\mathrm{1}\:−\:\mathrm{7k} \\ $$$$ \\ $$$$\mathrm{General}\:\mathrm{solution}\:\mathrm{of} \\ $$$$\mathrm{35x}\:+\:\mathrm{21y}\:+\:\mathrm{60z}\:\:=\:\mathrm{665} \\ $$$$\:\:\:\:\:\:\mathrm{y}\:\:=\:\:\left(\mathrm{3}\:+\:\mathrm{20k}\right)\mathrm{u}\:\:\:\:\:\:=\:\:\:\mathrm{3u}\:+\:\mathrm{20uk}\:\:\:\:=\:\:\:\mathrm{3u}\:+\:\mathrm{20n} \\ $$$$\:\:\:\:\:\:\mathrm{y}\:\:\:=\:\:\mathrm{3}\left(\mathrm{210}\:−\:\mathrm{35m}\right)\:+\:\mathrm{20n}\:\:\:=\:\:\:\mathrm{630}\:−\:\mathrm{105m}\:+\:\mathrm{20n} \\ $$$$ \\ $$$$\:\:\:\:\:\:\:\mathrm{z}\:\:=\:\:\left(−\:\mathrm{1}\:−\:\mathrm{7k}\right)\mathrm{u}\:\:\:=\:\:−\:\mathrm{u}\:−\:\mathrm{7uk}\:\:\:=\:\:−\:\mathrm{u}\:−\:\mathrm{7n} \\ $$$$\:\:\:\:\:\:\:\mathrm{z}\:\:=\:\:−\:\left(\mathrm{210}\:−\:\mathrm{35m}\right)\:−\:\mathrm{7n}\:\:\:=\:\:−\:\mathrm{210}\:+\:\mathrm{35m}\:−\:\mathrm{7n} \\ $$$$\mathrm{Therefore}, \\ $$$$\:\:\:\:\:\:\mathrm{x}\:\:=\:\:\mathrm{1}\:+\:\mathrm{3m} \\ $$$$\:\:\:\:\:\:\mathrm{y}\:\:=\:\:\mathrm{630}\:−\:\mathrm{105m}\:+\:\mathrm{20n} \\ $$$$\:\:\:\:\:\:\mathrm{z}\:\:=\:\:−\:\mathrm{210}\:+\:\mathrm{35m}\:−\:\mathrm{7n} \\ $$$$\mathrm{m},\:\mathrm{n}\:\:\mathrm{be}\:\mathrm{any}\:\mathrm{integer} \\ $$
Commented by TawaTawa last updated on 12/Jan/20
Sir mrW, i used the method you thought me sometime ago. Help me check.
$$\mathrm{Sir}\:\mathrm{mrW},\:\:\mathrm{i}\:\mathrm{used}\:\mathrm{the}\:\mathrm{method}\:\mathrm{you}\:\mathrm{thought}\:\mathrm{me}\:\mathrm{sometime}\:\mathrm{ago}. \\ $$$$\mathrm{Help}\:\mathrm{me}\:\mathrm{check}. \\ $$
Commented by mr W last updated on 12/Jan/20
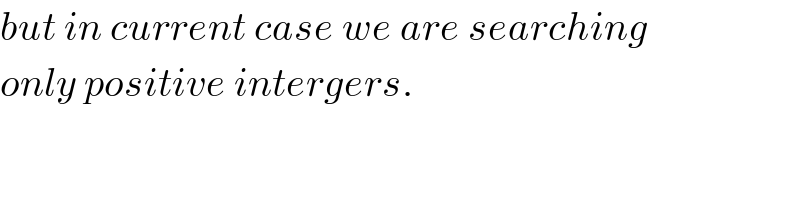
but in current case we are searching only positive intergers.
$${but}\:{in}\:{current}\:{case}\:{we}\:{are}\:{searching} \\ $$$${only}\:{positive}\:{intergers}. \\ $$
Commented by TawaTawa last updated on 12/Jan/20
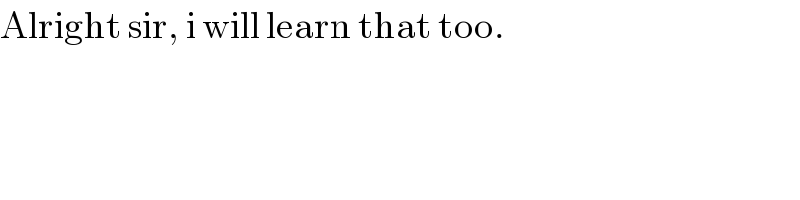
Alright sir, i will learn that too.
$$\mathrm{Alright}\:\mathrm{sir},\:\mathrm{i}\:\mathrm{will}\:\mathrm{learn}\:\mathrm{that}\:\mathrm{too}. \\ $$
Commented by mr W last updated on 12/Jan/20
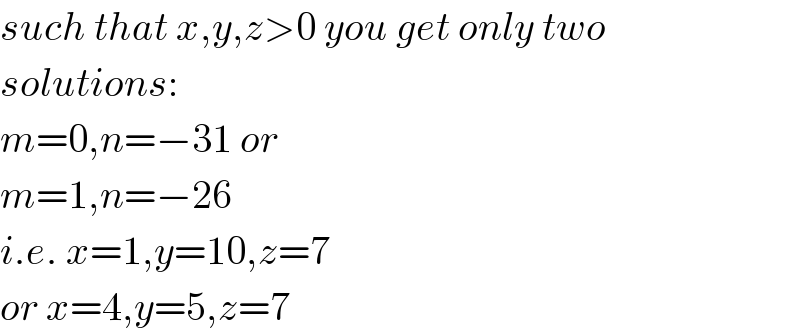
such that x,y,z>0 you get only two solutions: m=0,n=−31 or m=1,n=−26 i.e. x=1,y=10,z=7 or x=4,y=5,z=7
$${such}\:{that}\:{x},{y},{z}>\mathrm{0}\:{you}\:{get}\:{only}\:{two} \\ $$$${solutions}: \\ $$$${m}=\mathrm{0},{n}=−\mathrm{31}\:{or} \\ $$$${m}=\mathrm{1},{n}=−\mathrm{26} \\ $$$${i}.{e}.\:{x}=\mathrm{1},{y}=\mathrm{10},{z}=\mathrm{7} \\ $$$${or}\:{x}=\mathrm{4},{y}=\mathrm{5},{z}=\mathrm{7} \\ $$
Commented by TawaTawa last updated on 12/Jan/20
God bless you sir. I appreciate
$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}.\:\mathrm{I}\:\mathrm{appreciate} \\ $$
Commented by TawaTawa last updated on 12/Jan/20
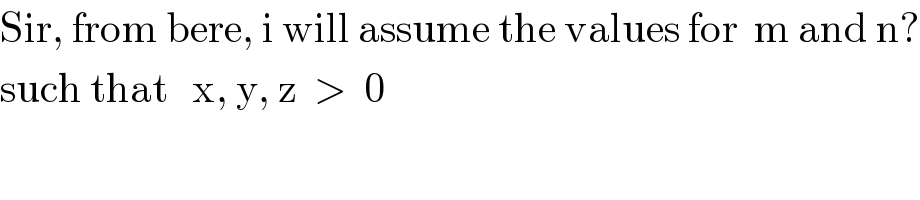
Sir, from bere, i will assume the values for m and n? such that x, y, z > 0
$$\mathrm{Sir},\:\mathrm{from}\:\mathrm{bere},\:\mathrm{i}\:\mathrm{will}\:\mathrm{assume}\:\mathrm{the}\:\mathrm{values}\:\mathrm{for}\:\:\mathrm{m}\:\mathrm{and}\:\mathrm{n}? \\ $$$$\mathrm{such}\:\mathrm{that}\:\:\:\mathrm{x},\:\mathrm{y},\:\mathrm{z}\:\:>\:\:\mathrm{0} \\ $$
Answered by key of knowledge last updated on 12/Jan/20
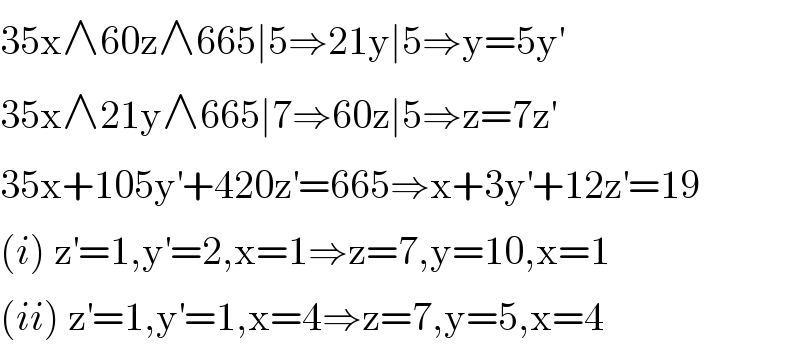
35x∧60z∧665∣5⇒21y∣5⇒y=5y^′ 35x∧21y∧665∣7⇒60z∣5⇒z=7z^′ 35x+105y^′ +420z^′ =665⇒x+3y^′ +12z^′ =19 (i) z^′ =1,y^′ =2,x=1⇒z=7,y=10,x=1 (ii) z^′ =1,y^′ =1,x=4⇒z=7,y=5,x=4
$$\mathrm{35x}\wedge\mathrm{60z}\wedge\mathrm{665}\mid\mathrm{5}\Rightarrow\mathrm{21y}\mid\mathrm{5}\Rightarrow\mathrm{y}=\mathrm{5y}^{'} \\ $$$$\mathrm{35x}\wedge\mathrm{21y}\wedge\mathrm{665}\mid\mathrm{7}\Rightarrow\mathrm{60z}\mid\mathrm{5}\Rightarrow\mathrm{z}=\mathrm{7z}^{'} \\ $$$$\mathrm{35x}+\mathrm{105y}^{'} +\mathrm{420z}^{'} =\mathrm{665}\Rightarrow\mathrm{x}+\mathrm{3y}^{'} +\mathrm{12z}^{'} =\mathrm{19} \\ $$$$\left({i}\right)\:\mathrm{z}^{'} =\mathrm{1},\mathrm{y}^{'} =\mathrm{2},\mathrm{x}=\mathrm{1}\Rightarrow\mathrm{z}=\mathrm{7},\mathrm{y}=\mathrm{10},\mathrm{x}=\mathrm{1} \\ $$$$\left({ii}\right)\:\mathrm{z}^{'} =\mathrm{1},\mathrm{y}^{'} =\mathrm{1},\mathrm{x}=\mathrm{4}\Rightarrow\mathrm{z}=\mathrm{7},\mathrm{y}=\mathrm{5},\mathrm{x}=\mathrm{4} \\ $$
Commented by mr W last updated on 12/Jan/20
very nice! thanks!
$${very}\:{nice}!\:{thanks}! \\ $$
Commented by jagoll last updated on 13/Jan/20
what the meaning 60z∣5 ? divided by 5 ? or mod 5 ?
$${what}\:{the}\:{meaning}\:\mathrm{60}{z}\mid\mathrm{5}\:?\: \\ $$$${divided}\:{by}\:\mathrm{5}\:?\:{or}\:{mod}\:\mathrm{5}\:? \\ $$
Commented by mr W last updated on 13/Jan/20
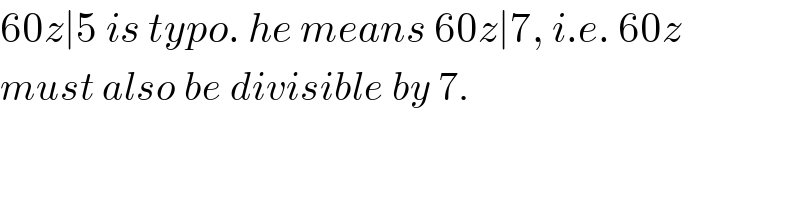
60z∣5 is typo. he means 60z∣7, i.e. 60z must also be divisible by 7.
$$\mathrm{60}{z}\mid\mathrm{5}\:{is}\:{typo}.\:{he}\:{means}\:\mathrm{60}{z}\mid\mathrm{7},\:{i}.{e}.\:\mathrm{60}{z} \\ $$$${must}\:{also}\:{be}\:{divisible}\:{by}\:\mathrm{7}. \\ $$
Commented by jagoll last updated on 13/Jan/20
oo typo sir. all right thanks sir
$${oo}\:{typo}\:{sir}.\:{all}\:{right}\:{thanks}\:{sir} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *