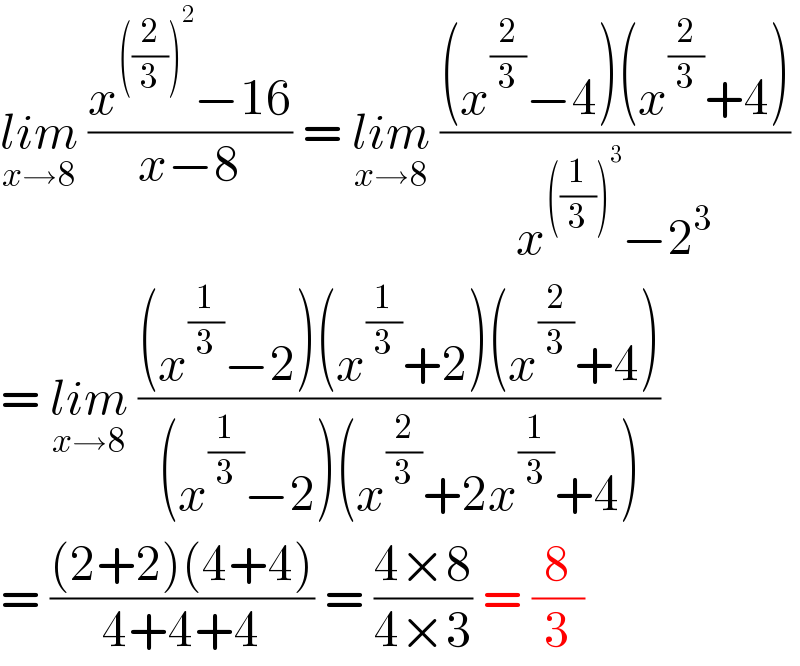solve-without-using-l-hopital-and-series-lim-x-8-x-x-1-3-16-x-8-

Question Number 133494 by Eric002 last updated on 22/Feb/21

s o l v e w i t h o u t u s i n g l^{'} h o p i t a l a n d s e r i e s

\lim_{x \to 8} \frac{x \sqrt[3]{x} - 16}{x - 8}

Answered by Olaf last updated on 22/Feb/21

X = \frac{x \sqrt[3]{x} - 16}{x - 8}

X = \frac{x^{\frac{4}{3}} - 2^{4}}{x^{\frac{3}{3}} - 2^{3}}

Let u = x^{\frac{1}{3}}

X = \frac{u^{4} - 2^{4}}{u^{3} - 2^{3}}

X = \frac{(u - 2) (u + 2) (u^{2} + 2^{2})}{(u - 2) (u^{2} + 2 u + 2^{2})}

X = \frac{(u + 2) (u^{2} + 2^{2})}{u^{2} + 2 u + 2^{2}}

\lim_{x \to 8} \frac{x \sqrt[3]{x} - 16}{x - 8} = \lim_{u \to 2} \frac{(u + 2) (u^{2} + 2^{2})}{u^{2} + 2 u + 2^{2}}

= \frac{4 \times 8}{12} = \frac{8}{3}

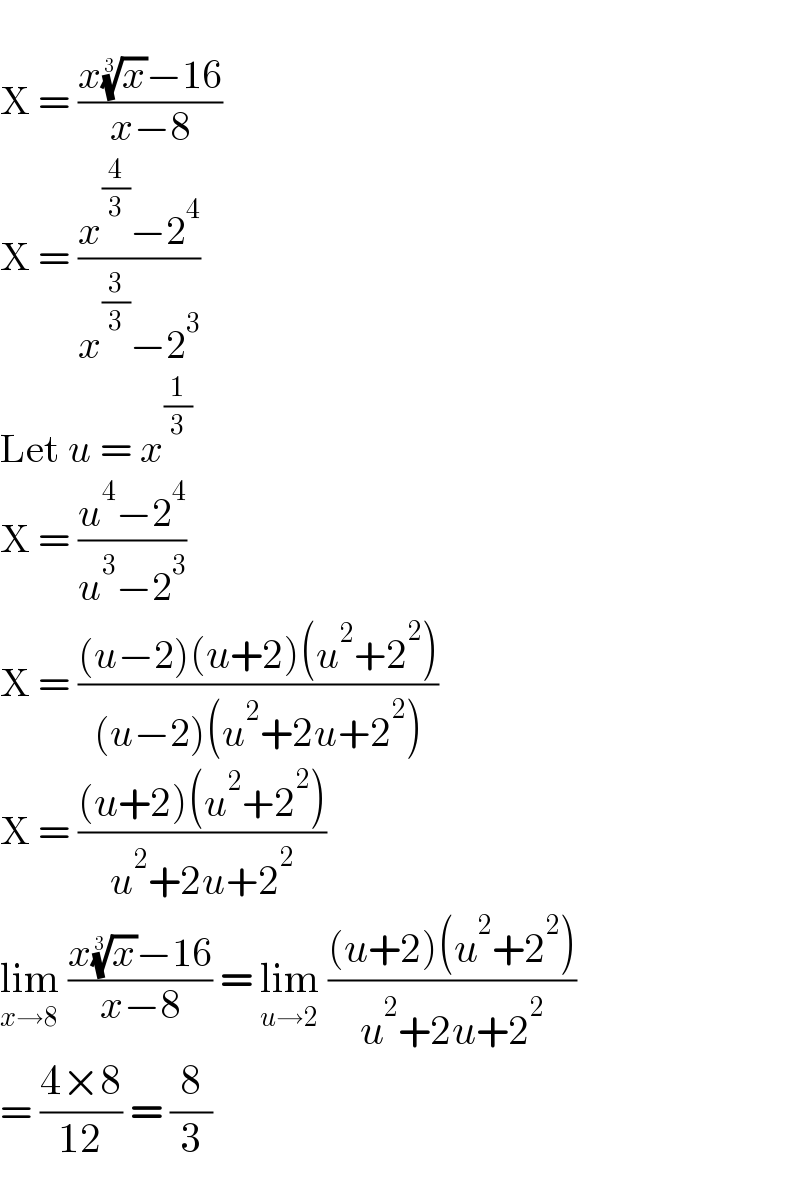
Commented by Eric002 last updated on 22/Feb/21

w e l l d o n e

Commented by otchereabdullai@gmail.com last updated on 14/Mar/21

nice one

Answered by Dwaipayan Shikari last updated on 22/Feb/21

x = t^{3}

\lim_{t \to 2} \frac{t^{4} - 16}{t^{3} - 8} = \frac{(t - 2) (t + 2) (t^{2} + 4)}{(t - 2) (t^{2} + 2 t + 4)} = \frac{4.8}{12} = \frac{8}{3}

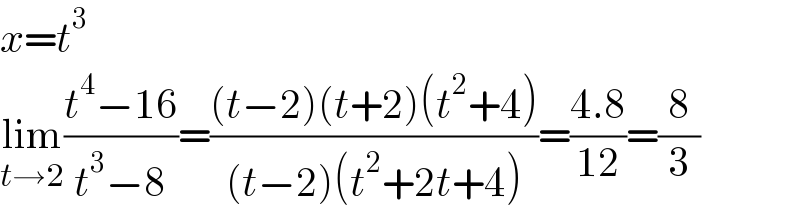
Answered by malwan last updated on 22/Feb/21

\underset{x \to 8}{l i m} \frac{x^{{(\frac{2}{3})}^{2}} - 16}{x - 8} = \underset{x \to 8}{l i m} \frac{(x^{\frac{2}{3}} - 4) (x^{\frac{2}{3}} + 4)}{x^{{(\frac{1}{3})}^{3}} - 2^{3}}

= \underset{x \to 8}{l i m} \frac{(x^{\frac{1}{3}} - 2) (x^{\frac{1}{3}} + 2) (x^{\frac{2}{3}} + 4)}{(x^{\frac{1}{3}} - 2) (x^{\frac{2}{3}} + 2 x^{\frac{1}{3}} + 4)}

= \frac{(2 + 2) (4 + 4)}{4 + 4 + 4} = \frac{4 \times 8}{4 \times 3} = \frac{8}{3}

Facebook Tweet Pin