Question Number 77483 by aliesam last updated on 06/Jan/20

$${solve}\: \\ $$$${y}'\:{cos}\left({x}\right)\:+\frac{\mathrm{1}}{\mathrm{2}}\:{y}\:{sin}\left({x}\right)\:=\:{e}^{{x}} \:\sqrt{{sin}\left({x}\right)}\:\: \\ $$
Commented by mathmax by abdo last updated on 06/Jan/20
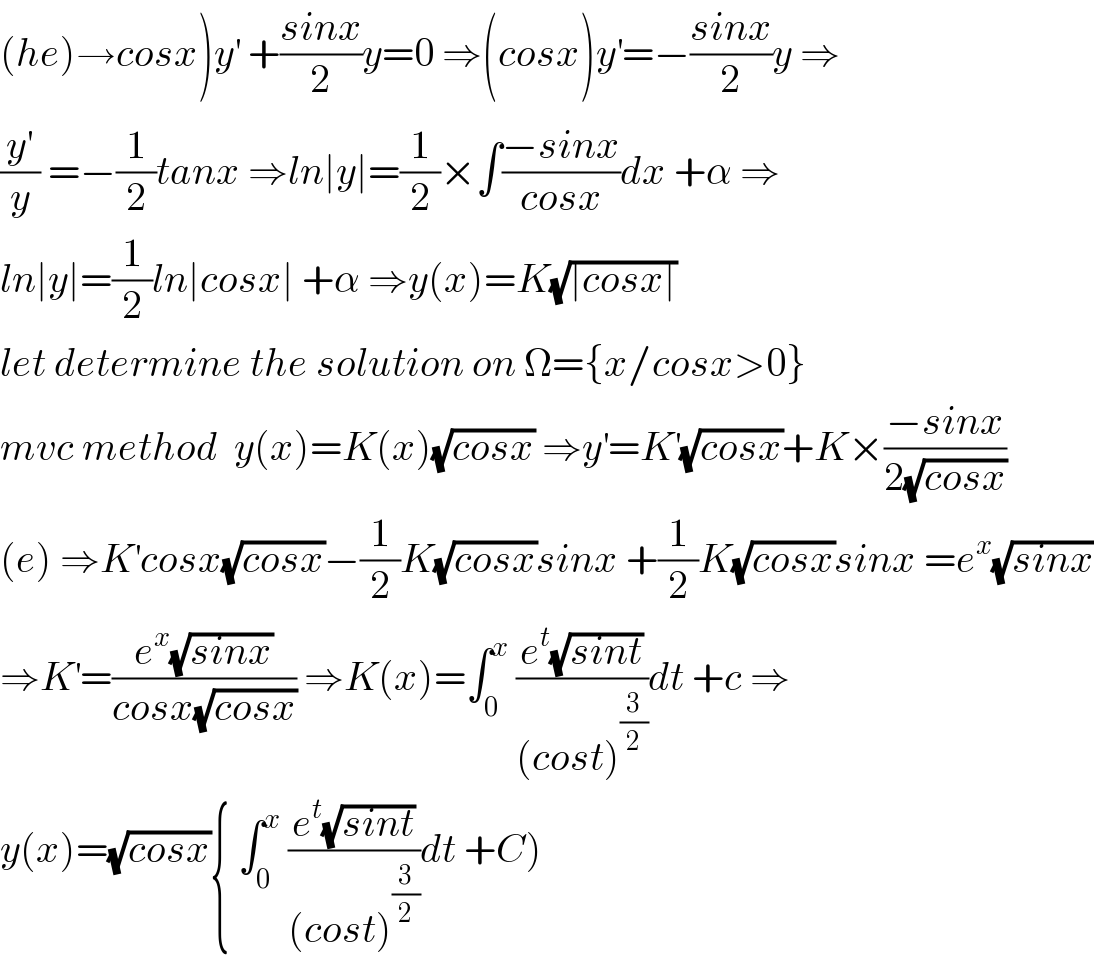
$$\left.\left({he}\right)\rightarrow{cosx}\right){y}^{'} \:+\frac{{sinx}}{\mathrm{2}}{y}=\mathrm{0}\:\Rightarrow\left({cosx}\right){y}^{'} =−\frac{{sinx}}{\mathrm{2}}{y}\:\Rightarrow \\ $$$$\frac{{y}^{'} }{{y}}\:=−\frac{\mathrm{1}}{\mathrm{2}}{tanx}\:\Rightarrow{ln}\mid{y}\mid=\frac{\mathrm{1}}{\mathrm{2}}×\int\frac{−{sinx}}{{cosx}}{dx}\:+\alpha\:\Rightarrow \\ $$$${ln}\mid{y}\mid=\frac{\mathrm{1}}{\mathrm{2}}{ln}\mid{cosx}\mid\:+\alpha\:\Rightarrow{y}\left({x}\right)={K}\sqrt{\mid{cosx}\mid} \\ $$$${let}\:{determine}\:{the}\:{solution}\:{on}\:\Omega=\left\{{x}/{cosx}>\mathrm{0}\right\} \\ $$$${mvc}\:{method}\:\:{y}\left({x}\right)={K}\left({x}\right)\sqrt{{cosx}}\:\Rightarrow{y}^{'} ={K}^{'} \sqrt{{cosx}}+{K}×\frac{−{sinx}}{\mathrm{2}\sqrt{{cosx}}} \\ $$$$\left({e}\right)\:\Rightarrow{K}^{'} {cosx}\sqrt{{cosx}}−\frac{\mathrm{1}}{\mathrm{2}}{K}\sqrt{{cosx}}{sinx}\:+\frac{\mathrm{1}}{\mathrm{2}}{K}\sqrt{{cosx}}{sinx}\:={e}^{{x}} \sqrt{{sinx}} \\ $$$$\Rightarrow{K}^{'} =\frac{{e}^{{x}} \sqrt{{sinx}}}{{cosx}\sqrt{{cosx}}}\:\Rightarrow{K}\left({x}\right)=\int_{\mathrm{0}} ^{{x}} \:\frac{{e}^{{t}} \sqrt{{sint}}}{\left({cost}\right)^{\frac{\mathrm{3}}{\mathrm{2}}} }{dt}\:+{c}\:\Rightarrow \\ $$$${y}\left({x}\right)=\sqrt{{cosx}}\left\{\:\int_{\mathrm{0}} ^{{x}} \:\frac{{e}^{{t}} \sqrt{{sint}}}{\left({cost}\right)^{\frac{\mathrm{3}}{\mathrm{2}}} }{dt}\:+{C}\right) \\ $$
