Question Number 1809 by alib last updated on 04/Oct/15

$$\mathrm{tan}\:{a}+\mathrm{cot}\:{a}+\mathrm{tan}\:\mathrm{3}{a}+\mathrm{cot}\:\mathrm{3}{a}=\frac{\mathrm{8cos}\:^{\mathrm{2}} \mathrm{2}{a}}{\mathrm{sin}\:\mathrm{6}{a}} \\ $$$$ \\ $$
Commented by alib last updated on 04/Oct/15

$$\boldsymbol{{prove}} \\ $$
Answered by 112358 last updated on 05/Oct/15
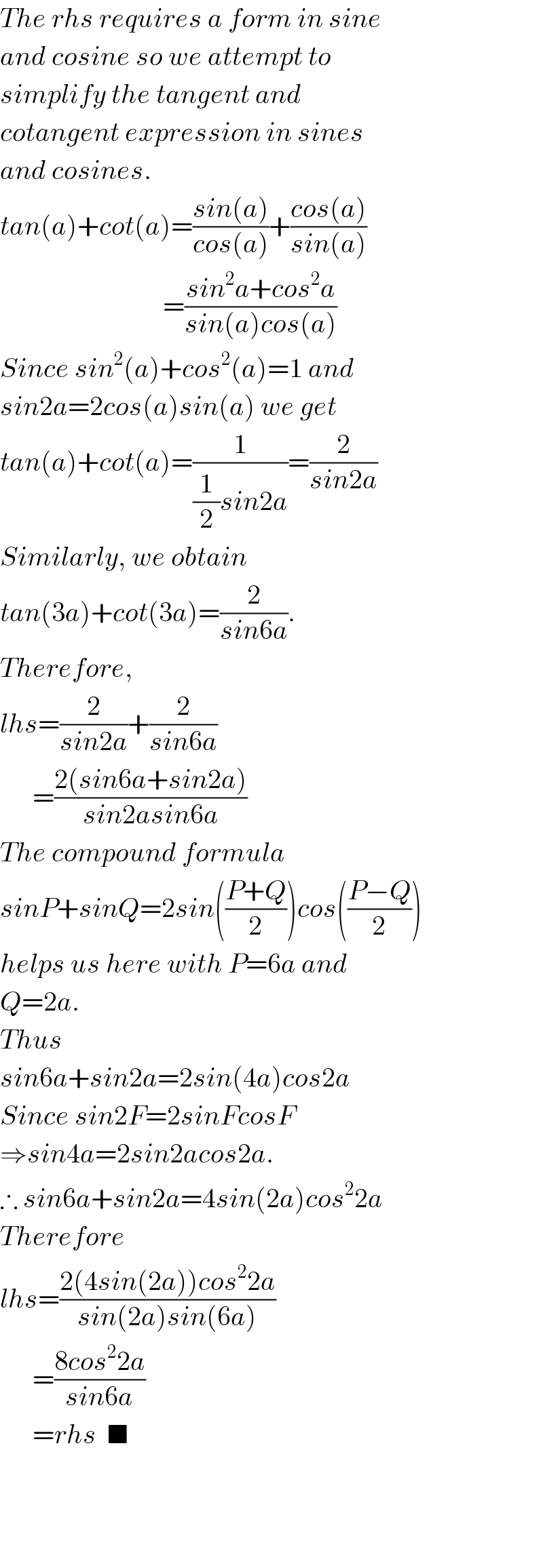
$${The}\:{rhs}\:{requires}\:{a}\:{form}\:{in}\:{sine} \\ $$$${and}\:{cosine}\:{so}\:{we}\:{attempt}\:{to}\: \\ $$$${simplify}\:{the}\:{tangent}\:{and}\: \\ $$$${cotangent}\:{expression}\:{in}\:{sines} \\ $$$${and}\:{cosines}. \\ $$$${tan}\left({a}\right)+{cot}\left({a}\right)=\frac{{sin}\left({a}\right)}{{cos}\left({a}\right)}+\frac{{cos}\left({a}\right)}{{sin}\left({a}\right)} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{{sin}^{\mathrm{2}} {a}+{cos}^{\mathrm{2}} {a}}{{sin}\left({a}\right){cos}\left({a}\right)} \\ $$$${Since}\:{sin}^{\mathrm{2}} \left({a}\right)+{cos}^{\mathrm{2}} \left({a}\right)=\mathrm{1}\:{and}\: \\ $$$${sin}\mathrm{2}{a}=\mathrm{2}{cos}\left({a}\right){sin}\left({a}\right)\:{we}\:{get} \\ $$$${tan}\left({a}\right)+{cot}\left({a}\right)=\frac{\mathrm{1}}{\frac{\mathrm{1}}{\mathrm{2}}{sin}\mathrm{2}{a}}=\frac{\mathrm{2}}{{sin}\mathrm{2}{a}} \\ $$$${Similarly},\:{we}\:{obtain} \\ $$$${tan}\left(\mathrm{3}{a}\right)+{cot}\left(\mathrm{3}{a}\right)=\frac{\mathrm{2}}{{sin}\mathrm{6}{a}}. \\ $$$${Therefore},\: \\ $$$${lhs}=\frac{\mathrm{2}}{{sin}\mathrm{2}{a}}+\frac{\mathrm{2}}{{sin}\mathrm{6}{a}} \\ $$$$\:\:\:\:\:\:=\frac{\mathrm{2}\left({sin}\mathrm{6}{a}+{sin}\mathrm{2}{a}\right)}{{sin}\mathrm{2}{asin}\mathrm{6}{a}} \\ $$$${The}\:{compound}\:{formula} \\ $$$${sinP}+{sinQ}=\mathrm{2}{sin}\left(\frac{{P}+{Q}}{\mathrm{2}}\right){cos}\left(\frac{{P}−{Q}}{\mathrm{2}}\right) \\ $$$${helps}\:{us}\:{here}\:{with}\:{P}=\mathrm{6}{a}\:{and} \\ $$$${Q}=\mathrm{2}{a}.\: \\ $$$${Thus}\: \\ $$$${sin}\mathrm{6}{a}+{sin}\mathrm{2}{a}=\mathrm{2}{sin}\left(\mathrm{4}{a}\right){cos}\mathrm{2}{a} \\ $$$${Since}\:{sin}\mathrm{2}{F}=\mathrm{2}{sinFcosF}\: \\ $$$$\Rightarrow{sin}\mathrm{4}{a}=\mathrm{2}{sin}\mathrm{2}{acos}\mathrm{2}{a}.\: \\ $$$$\therefore\:{sin}\mathrm{6}{a}+{sin}\mathrm{2}{a}=\mathrm{4}{sin}\left(\mathrm{2}{a}\right){cos}^{\mathrm{2}} \mathrm{2}{a} \\ $$$${Therefore}\: \\ $$$${lhs}=\frac{\mathrm{2}\left(\mathrm{4}{sin}\left(\mathrm{2}{a}\right)\right){cos}^{\mathrm{2}} \mathrm{2}{a}}{{sin}\left(\mathrm{2}{a}\right){sin}\left(\mathrm{6}{a}\right)} \\ $$$$\:\:\:\:\:\:=\frac{\mathrm{8}{cos}^{\mathrm{2}} \mathrm{2}{a}}{{sin}\mathrm{6}{a}} \\ $$$$\:\:\:\:\:\:={rhs}\:\:\blacksquare \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
