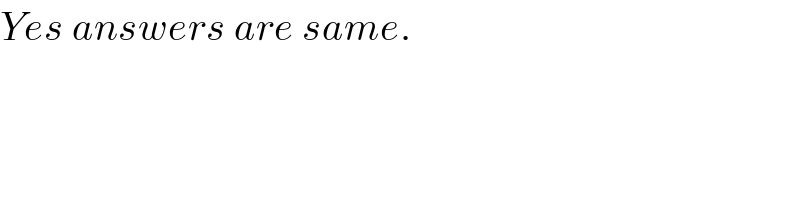Two-tractor-working-together-have-plowed-the-field-for-48-hours-If-half-of-the-field-is-plowed-by-one-of-them-and-then-the-remaining-half-to-the-other-the-work-would-be-carried-out-100-hours-Ho

FacebookTweetPin

Question Number 4539 by love math last updated on 05/Feb/16

$$Twotractor,workingtogether,$$$$haveplowedthefieldfor48hours.$$$$Ifhalfofthefieldisplowedby$$$$oneofthemandthentheremaining$$$$halftotheother,theworkwould$$$$becarriedout100hours.Howmany$$$$hourstoplowthefieldifeverytractor$$$$workingseparately?$$

Commented by Yozzii last updated on 07/Feb/16

$$Letp\left(1\right)=areaplowedperhourby$$$$tractor1andp\left(2\right)theareaplowed$$$$perhourbytractor2.Ingeneral,$$$$definep=\frac{totalareaplowed}{totaltimetaken}=\frac{a}{t}(a,t>0)$$$$\Rightarrow t=\frac{a}{p}.Forfixeda\Rightarrow t\propto \frac{1}{p}.$$$$\Rightarrow fortwotractors,tfallssince$$$$p=p\left(1\right)+p\left(2\right).$$$$\therefore 48(p\left(1\right)+p\left(2\right))=a\dots \dots ..\left(1\right)$$$$Sincet=\frac{a}{p},forthesecondcase,$$$$100=\frac{0.5a}{p\left(1\right)}+\frac{0.5a}{p\left(2\right)}$$$$\frac{200}{a}=\frac{1}{p\left(1\right)}+\frac{1}{p\left(2\right)}=\frac{p\left(1\right)+p\left(2\right)}{p\left(1\right)p\left(2\right)}=\frac{a/48}{p\left(1\right)p\left(2\right)}$$$$p\left(1\right)p\left(2\right)=\frac{a^2}{48\times 200}=\frac{a^2}{9600}$$$$\Rightarrow p\left(1\right)=\frac{a^2}{9600p\left(2\right)}\dots \dots \dots \dots .\left(2\right)$$$$Substitutingp\left(1\right)from\left(2\right)into\left(1\right)$$$$\Rightarrow 48(\frac{a^2}{9600p\left(2\right)}+p\left(2\right))=a$$$$\frac{a^2}{200p\left(2\right)}+48p\left(2\right)=a$$$$a^2+9600p{\left(2\right)}^2=200ap\left(2\right)$$$$9600p{\left(2\right)}^2-200ap\left(2\right)+a^2=0$$$$\Rightarrow p\left(2\right)=\frac{200a\pm \sqrt{40000a^2-4\times 9600\times a^2}}{2\times 9600}$$$$p\left(2\right)=\frac{200a\pm 40a}{2\times 10\times 2\times 480}=\frac{5a\pm a}{480}=\frac{6}{480}a,\frac{4}{480}a$$$$p\left(2\right)=\frac{a}{80},\frac{a}{120}$$$$\therefore p\left(1\right)=\frac{a^2}{9600}\times \frac{80}{a},\frac{a^2}{9600}\times \frac{120}{a}$$$$p\left(1\right)=\frac{a}{120},\frac{a}{80}.$$$$So\{p\left(1\right),p\left(2\right)\}=\{\frac{a}{80},\frac{a}{120}\}\vee \{\frac{a}{120},\frac{a}{80}\}$$$$Iftractor1worksalone,$$$$t_1=\frac{a}{a/80}=80hours$$$$Iftractor2worksalone,$$$$t_2=\frac{a}{a/120}=120hours.$$$$Thesymbolsforeachtractorare$$$$assignedarbitrarilysothatinall,$$$$oneofthetractors\left(1or2\right)takes80$$$$hoursbythemselvestoplowthefield$$$$whiletheother\left(2or1\right)takes120hours.$$$$$$$$$$$$$$

Answered by Rasheed Soomro last updated on 07/Feb/16

$$LettractorAcanploughwholefield$$$$inxhoursAND$$$$tractorBinyhours.$$$$Perhour:$$$$Aploughs\frac{1}{x}offieldperhour$$$$Bploughs\frac{1}{y}offieldperhour$$$$In48hours:$$$$Aploughs\frac{48}{x}offield$$$$Bploughs\frac{48}{y}offield$$$$\frac{48}{x}+\frac{48}{y}=1\left(Wholefield\right)\dots \dots \dots \left(i\right)$$$$Halffield:$$$$Aploughshalffieldin\frac{x}{2}hours$$$$Bploughshalffieldin\frac{y}{2}hours$$$$\frac{x}{2}+\frac{y}{2}=100\dots \dots \dots \dots \dots \dots \dots \left(ii\right)$$$$x+y=200$$$$y=200-x$$$$Substitutingin\left(i\right)$$$$\frac{48}{x}+\frac{48}{200-x}=1$$$$48(200-x)+48x=x(200-x)$$$$9600-48x+48x=200x-x^2$$$$x^2-200x+9600=0$$$$x=\frac{-(-200)\pm \sqrt{{(-200)}^2-4\left(1\right)\left(9600\right)}}{2\left(1\right)}$$$$=\frac{200\pm 40}{2}=120,80$$$$$$$$IfAploughsthefieldin120hours,Bploughs$$$$200-120=80hours.$$$$IfAploughsthefieldin80hours,Bploughs$$$$200-80=120hours.$$

Commented by Yozzii last updated on 07/Feb/16

$$Myanswersatisfiesyourequations.$$$$(x,y)=(80,120)$$

Commented by Rasheed Soomro last updated on 07/Feb/16

$$Yesanswersaresame.$$

Terms of Service
Privacy Policy
Contact: info@tinkutara.com

FacebookTweetPin