What-are-the-dimensions-of-the-rectangle-of-maximum-area-that-can-be-inscribed-in-the-portion-of-the-parabola-x-2-8y-intercepted-by-the-line-y-2-

Question Number 136006 by liberty last updated on 17/Mar/21

What are the dimensions of the rectangle of maximum area that can be inscribed in the portion of the parabola x^2=8y intercepted by the line y=2?

Answered by mr W last updated on 18/Mar/21

A = 2 p (2 - \frac{p^{2}}{8})

\frac{d A}{d p} = 4 - \frac{3 p^{2}}{4} = 0

\Rightarrow p = \frac{4}{\sqrt{3}} = \frac{4 \sqrt{3}}{3}

B = 2 p = \frac{8 \sqrt{3}}{3}

H = 2 - \frac{16 \times 3}{8 \times 9} = 2 - \frac{2}{3} = \frac{4}{3}

A_{m a x} = \frac{8 \sqrt{3}}{3} \times \frac{4}{3} = \frac{32 \sqrt{3}}{9}

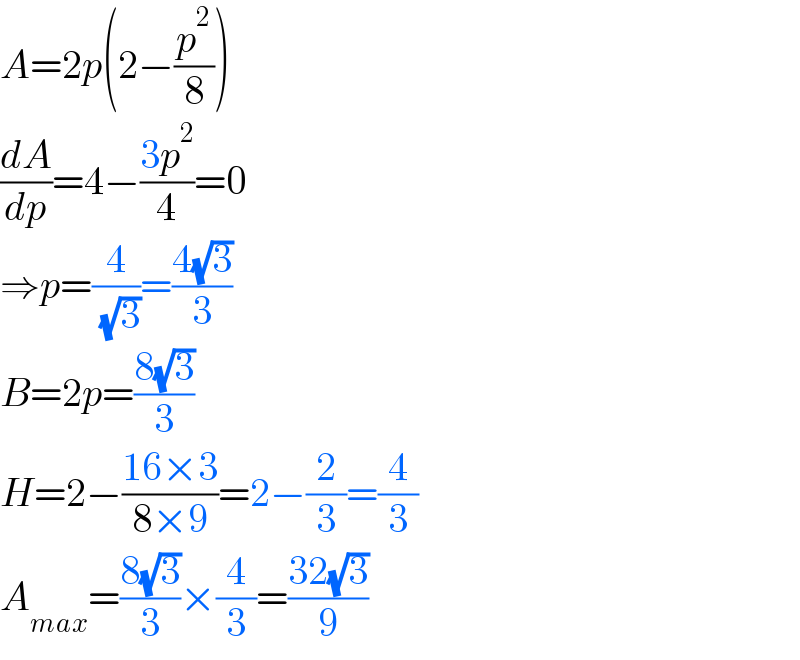
Commented by otchereabdullai@gmail.com last updated on 17/Mar/21

nice!

Commented by liberty last updated on 17/Mar/21

i g o t \frac{32}{3 \sqrt{3}} s i r

Commented by liberty last updated on 18/Mar/21

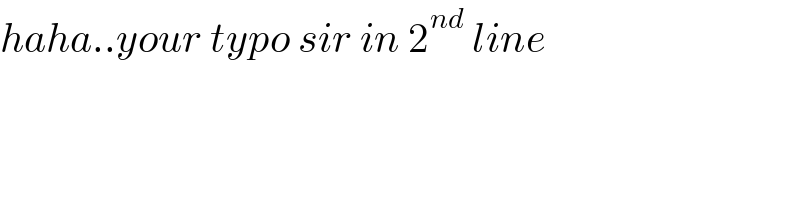
h a h a . . y o u r t y p o s i r i n 2^{n d} l i n e

Commented by mr W last updated on 18/Mar/21

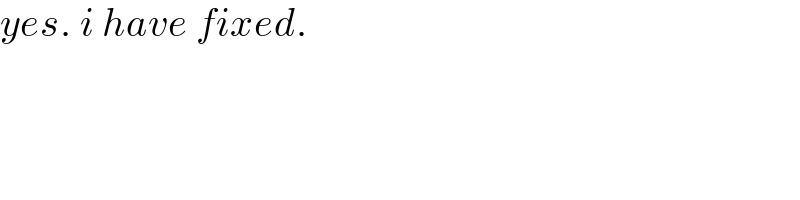
y e s . i h a v e f i x e d .

Answered by liberty last updated on 17/Mar/21

l e t A (- x, y) a n d B (x, y) t w o

p o i n t a t p a r a b o l a

t h e a r e a o f r e c t a n g l e i s A = 2 x (2 - y)

A (x) = 2 x (2 - \frac{x^{2}}{8}) = 4 x - \frac{x^{3}}{4}

A^{'} (x) = 4 - \frac{3}{4} x^{2} = 0 \Rightarrow x = \frac{4}{\sqrt{3}}

A {(x)}_{m a x} = \frac{8}{\sqrt{3}} (2 - \frac{16}{24}) = \frac{8}{\sqrt{3}} (2 - \frac{2}{3})

= \frac{32}{3 \sqrt{3}}

Facebook Tweet Pin