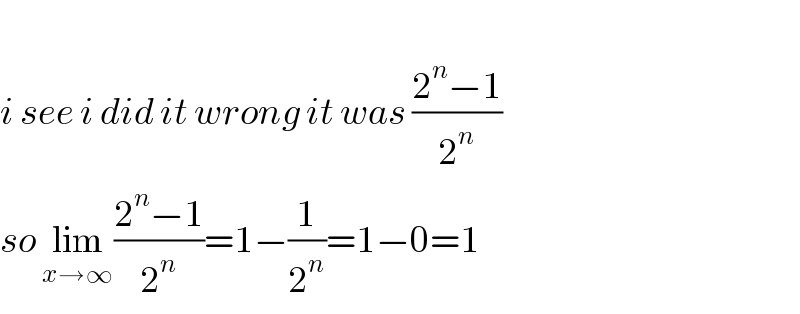why-1-1-2-1-4-1-8-2-

Question Number 5158 by 1771727373 last updated on 24/Apr/16

w h y

1 + \frac{1}{2} + \frac{1}{4} + \frac{1}{8} + \dots \dots \dots . . = 2

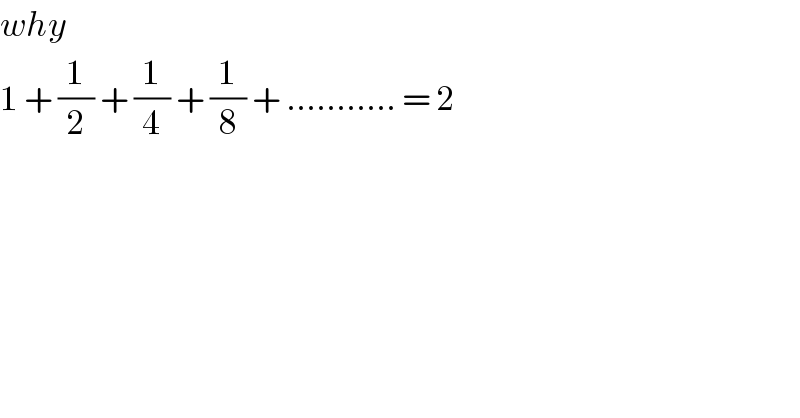
Answered by FilupSmith last updated on 24/Apr/16

1 + \frac{1}{2} + \frac{1}{4} + \dots = S

∴ 2 S = 2 + 1 + \frac{1}{2} + \dots

∴ 2 S = 2 + (1 + \frac{1}{2} + \dots)

2 S = 2 + S

∴ S = 2

∴ 1 + \frac{1}{2} + \frac{1}{4} + \dots = 2

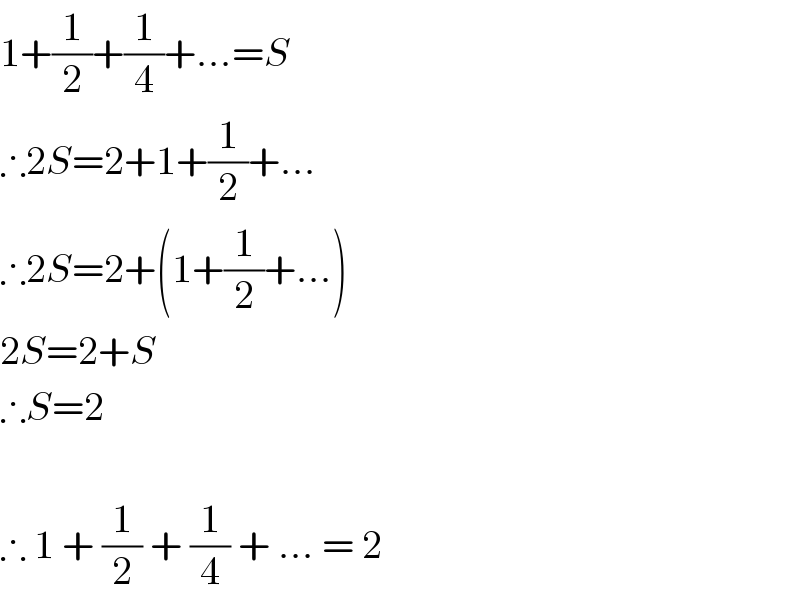
Commented by 1771727373 last updated on 24/Apr/16

o r

\frac{1}{2} + \frac{1}{4} + \frac{1}{8} = \frac{7}{8}

\frac{1}{2} + \frac{1}{4} = \frac{3}{4}

\frac{1}{2} = \frac{1}{2}

∵ \frac{n^{2} - 1}{n^{2}} = Z

\lim_{x \to \infty} (1 + Z) = 1 + 1 + \frac{1}{\infty} = 2

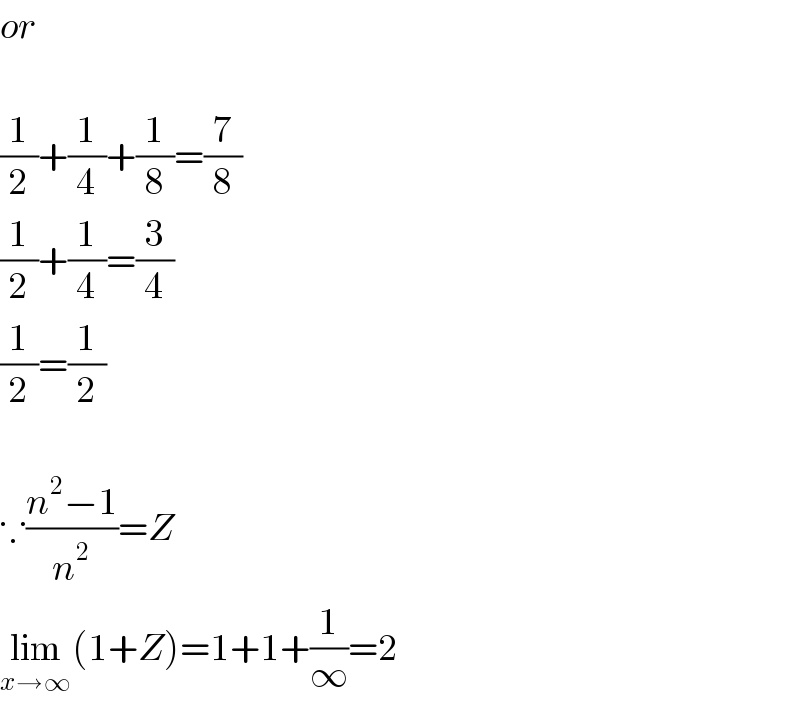
Commented by FilupSmith last updated on 25/Apr/16

I {don}^{'} t think this is correct .

The limit only shows its divergance .

Not its sum

Commented by prakash jain last updated on 26/Apr/16

\frac{1}{2} + \frac{1}{4} + \frac{1}{8} = \frac{7}{8} \neq \frac{n^{2} - 1}{n^{2}}

There is no n for which \frac{n^{2} - 1}{n^{2}} = \frac{7}{8}

8 n^{2} - 8 = 7 n^{2} \Rightarrow n^{2} = 8 (n o t p o s s i b l e)

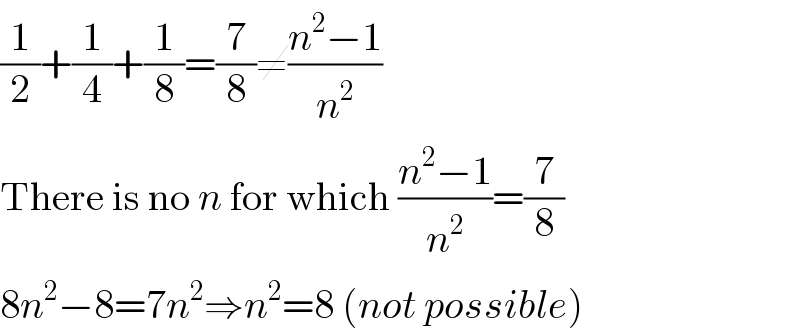
Commented by 1771727373 last updated on 27/Apr/16

i s e e i d i d i t w r o n g i t w a s \frac{2^{n} - 1}{2^{n}}

s o \lim_{x \to \infty} \frac{2^{n} - 1}{2^{n}} = 1 - \frac{1}{2^{n}} = 1 - 0 = 1