Question Number 11827 by uni last updated on 01/Apr/17
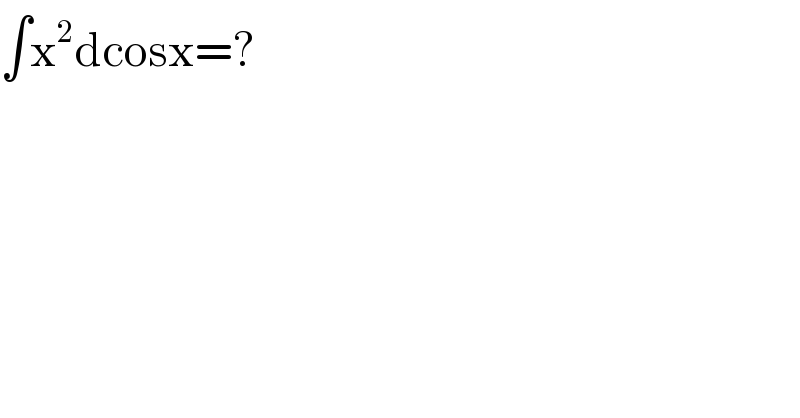
$$\int\mathrm{x}^{\mathrm{2}} \mathrm{dcosx}=? \\ $$
Answered by mrW1 last updated on 01/Apr/17
![=x^2 cos x−∫cos xd(x^2 ) =x^2 cos x−2∫xcos xdx =x^2 cos x−2∫xdsin x =x^2 cos x−2[xsin x−∫sin xdx] =x^2 cos x−2[xsin x+cos x]+C =(x^2 −2)cos x−2xsin x+C](https://www.tinkutara.com/question/Q11829.png)
$$={x}^{\mathrm{2}} \mathrm{cos}\:{x}−\int\mathrm{cos}\:{xd}\left({x}^{\mathrm{2}} \right) \\ $$$$={x}^{\mathrm{2}} \mathrm{cos}\:{x}−\mathrm{2}\int{x}\mathrm{cos}\:{xdx} \\ $$$$={x}^{\mathrm{2}} \mathrm{cos}\:{x}−\mathrm{2}\int{xd}\mathrm{sin}\:{x} \\ $$$$={x}^{\mathrm{2}} \mathrm{cos}\:{x}−\mathrm{2}\left[{x}\mathrm{sin}\:{x}−\int\mathrm{sin}\:{xdx}\right] \\ $$$$={x}^{\mathrm{2}} \mathrm{cos}\:{x}−\mathrm{2}\left[{x}\mathrm{sin}\:{x}+\mathrm{cos}\:{x}\right]+{C} \\ $$$$=\left({x}^{\mathrm{2}} −\mathrm{2}\right)\mathrm{cos}\:{x}−\mathrm{2}{x}\mathrm{sin}\:{x}+{C} \\ $$
