x-8-x-2-4x-16-dx-

Question Number 7872 by tawakalitu last updated on 22/Sep/16

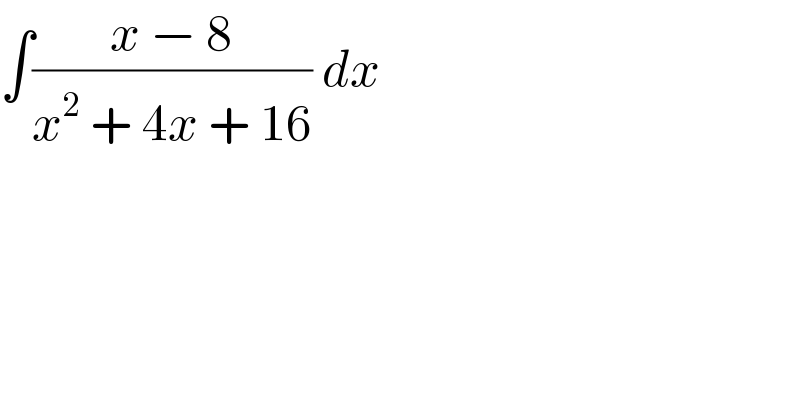
\int \frac{x - 8}{x^{2} + 4 x + 16} d x

Commented by prakash jain last updated on 23/Sep/16

\frac{x - 8}{x^{2} + 4 x + 16} = \frac{x - 8}{x^{2} + 4 x + 4 + 12}

= \frac{x - 8}{{(x + 2)}^{2} - {(i \sqrt{12})}^{2}}

= \frac{x - 8}{(x + 2 + i \sqrt{12}) (x + 2 - i \sqrt{12})}

c o n v e r t t o p a r t i a l f r a c t i o n

(x - 8) (\frac{1}{x + 2 - i \sqrt{12}} - \frac{1}{x + 2 + i \sqrt{12}}) \frac{1}{2 i \sqrt{12}}

(\frac{x - 8}{x - 2 - i \sqrt{12}} - \frac{x - 8}{x - 2 + i \sqrt{12}}) \frac{1}{2 i \sqrt{12}}

(\frac{x + 2 - i \sqrt{12} - (10 - i \sqrt{12})}{x + 2 - i \sqrt{12}} - \frac{x + 2 + i \sqrt{12} - (10 + i \sqrt{12})}{x + 2 + i \sqrt{12}}) \frac{1}{2 i \sqrt{12}}

(\frac{(10 - i \sqrt{12})}{x - 2 - i \sqrt{12}} - \frac{(10 + i \sqrt{12})}{x - 2 + i \sqrt{12}}) \frac{1}{2 i \sqrt{12}}

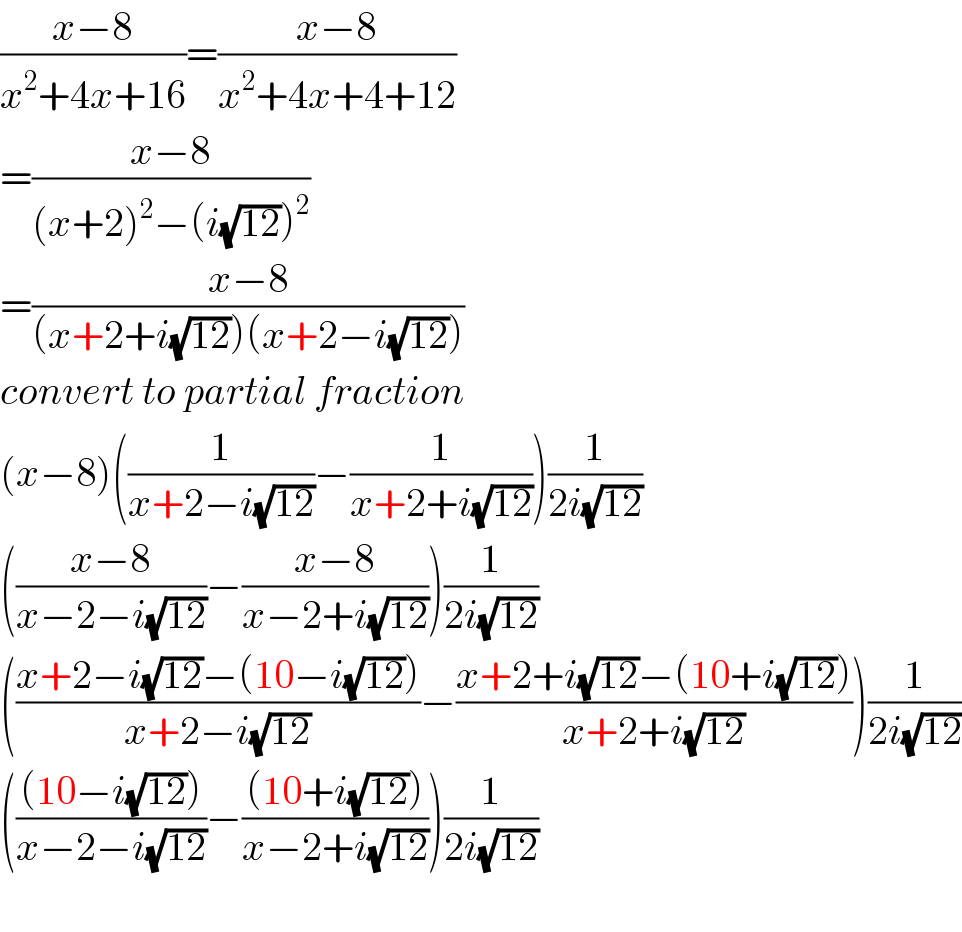
Commented by tawakalitu last updated on 22/Sep/16

T h a n k s s o m u c h

Commented by sandy_suhendra last updated on 23/Sep/16

I t h i n k {(x + 2)}^{2} - {(i \sqrt{12})}^{2} = (x + 2 + i \sqrt{12}) (x + 2 - i \sqrt{12})

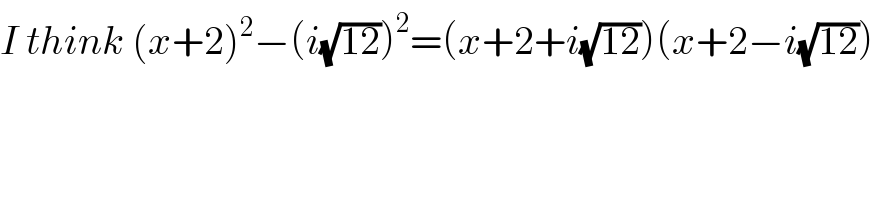
Commented by prakash jain last updated on 23/Sep/16

Thanks

Answered by prakash jain last updated on 02/Oct/16

see comments