Question Number 1016 by 123456 last updated on 14/May/15
![[(x^→ ×a^→ )×b^→ ]×x^→ =c^→](https://www.tinkutara.com/question/Q1016.png)
Answered by rpatle69@gmail.com last updated on 14/May/15
![[−b^→ ×(x^→ ×a^→ )]×x^→ =c^→ x^→ ×[b^→ ×(x^→ ×a^→ )]=c^→ x^→ ×[(b^→ .a^→ )x^→ −(b^→ .x^→ )a^→ ]=c^→](https://www.tinkutara.com/question/Q1017.png)
Commented by prakash jain last updated on 17/May/15

Commented by 123456 last updated on 17/May/15
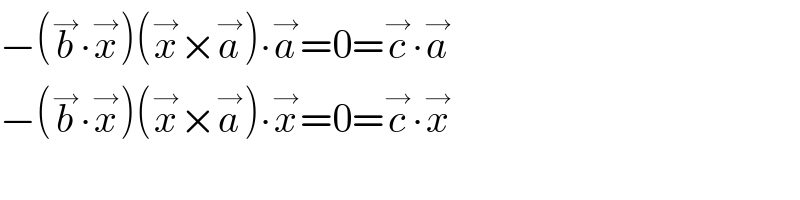
Commented by 123456 last updated on 17/May/15

