Question Number 131697 by mathlove last updated on 07/Feb/21

Answered by mr W last updated on 07/Feb/21
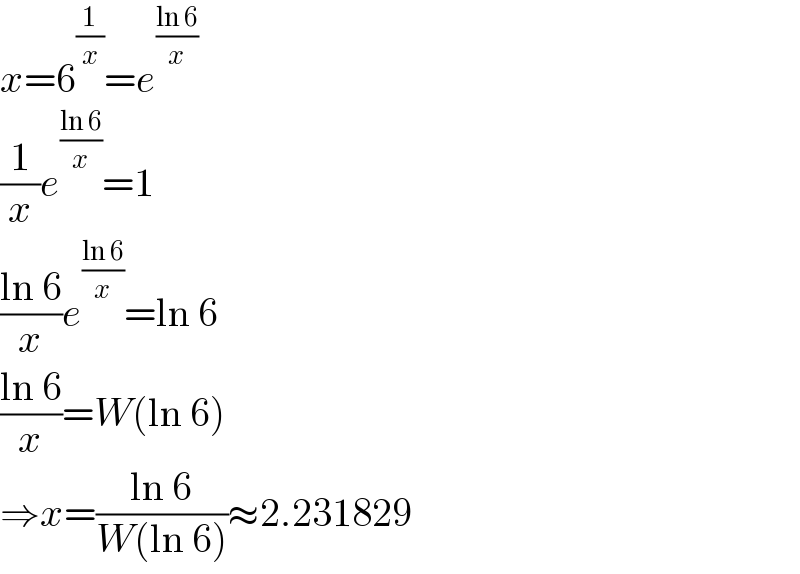
Commented by mathlove last updated on 07/Feb/21

Commented by mathlove last updated on 07/Feb/21

Commented by mr W last updated on 07/Feb/21

Answered by Dwaipayan Shikari last updated on 07/Feb/21

Commented by I want to learn more last updated on 07/Feb/21
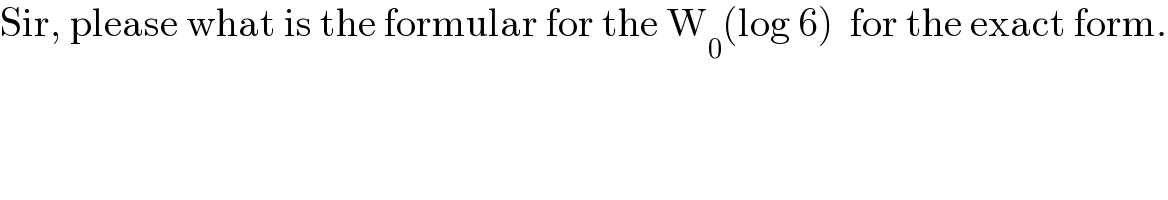
Commented by Dwaipayan Shikari last updated on 07/Feb/21

Commented by I want to learn more last updated on 07/Feb/21