Menu Close

x-y-z-Z-find-all-solutions-of-xy-x-y-z-

Tinku Tara 0 Comments
Pin




Question Number 69939 by mr W last updated on 29/Sep/19
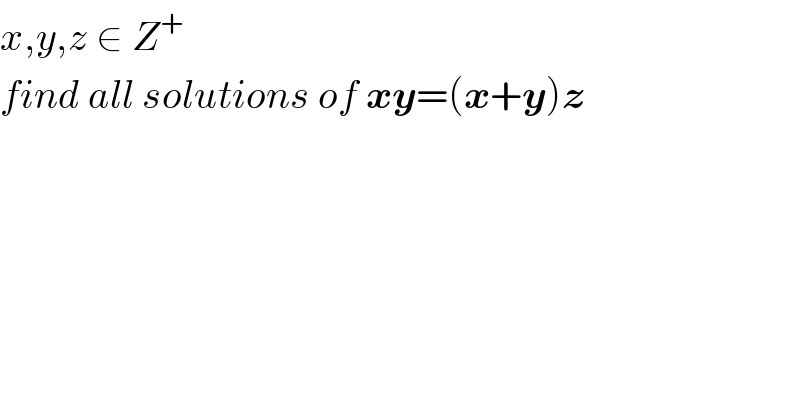
x,y,z ∈ Z^+ find all solutions of xy=(x+y)z
$${x},{y},{z}\:\in\:{Z}^{+} \\ $$$${find}\:{all}\:{solutions}\:{of}\:\boldsymbol{{xy}}=\left(\boldsymbol{{x}}+\boldsymbol{{y}}\right)\boldsymbol{{z}} \\ $$
Commented by Rasheed.Sindhi last updated on 29/Sep/19
k∈Z^+ in the following. (x,y,z)=(2k,2k,k), (5k,20k,4k),(20k,5k,4k), (3k,6k,2k),(6k,3k,2k),(7k,42k,6k), (4,12,3) ^(• ) If (p,q,r) is a solution then (q,p,r) is also solution. ^• If (p,q,r) is a solution then (pk,qk,rk) is also solution.k∈Z^+ ....
$${k}\in\mathbb{Z}^{+} \:{in}\:{the}\:{following}. \\ $$$$\left({x},{y},{z}\right)=\left(\mathrm{2}{k},\mathrm{2}{k},{k}\right), \\ $$$$\left(\mathrm{5}{k},\mathrm{20}{k},\mathrm{4}{k}\right),\left(\mathrm{20}{k},\mathrm{5}{k},\mathrm{4}{k}\right), \\ $$$$\left(\mathrm{3}{k},\mathrm{6}{k},\mathrm{2}{k}\right),\left(\mathrm{6}{k},\mathrm{3}{k},\mathrm{2}{k}\right),\left(\mathrm{7}{k},\mathrm{42}{k},\mathrm{6}{k}\right), \\ $$$$\left(\mathrm{4},\mathrm{12},\mathrm{3}\right) \\ $$$$\:^{\bullet\:} {If}\:\left({p},{q},{r}\right)\:{is}\:{a}\:{solution}\:{then}\:\left({q},{p},{r}\right) \\ $$$$\:\:{is}\:{also}\:{solution}. \\ $$$$\:^{\bullet} \:{If}\:\left({p},{q},{r}\right)\:{is}\:{a}\:{solution}\:{then}\:\left({pk},{qk},{rk}\right) \\ $$$$\:\:\:\:{is}\:{also}\:{solution}.{k}\in\mathbb{Z}^{+} \\ $$$$…. \\ $$
Commented by mr W last updated on 29/Sep/19
thanks sir! these are solutions. but how can we express ALL possible solutions?
$${thanks}\:{sir}! \\ $$$${these}\:{are}\:{solutions}.\:{but}\:{how}\:{can}\:{we}\: \\ $$$${express}\:{ALL}\:{possible}\:{solutions}? \\ $$
Commented by Rasheed.Sindhi last updated on 29/Sep/19
Sir at the moment I have no idea of expressing all the possible solutions! However I am thinking about it...
$${Sir}\:{at}\:{the}\:{moment}\:{I}\:{have}\:{no}\:{idea}\:{of} \\ $$$${expressing}\:{all}\:{the}\:{possible}\:{solutions}! \\ $$$${However}\:{I}\:{am}\:{thinking}\:{about}\:{it}… \\ $$
Commented by mr W last updated on 29/Sep/19
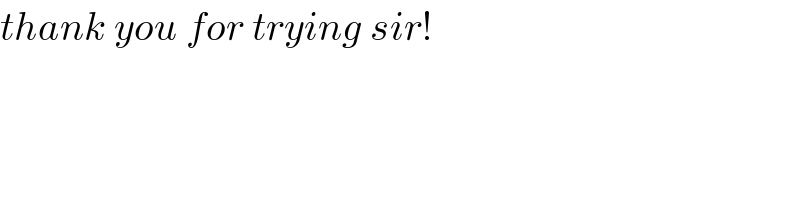
thank you for trying sir!
$${thank}\:{you}\:{for}\:{trying}\:{sir}! \\ $$
Commented by Rasheed.Sindhi last updated on 29/Sep/19
Sir I think infinite solutions! I′ll try to write some observations about such triplets which satisfy your equation.
$${Sir}\:{I}\:{think}\:{infinite}\:{solutions}! \\ $$$${I}'{ll}\:{try}\:{to}\:{write}\:{some}\:{observations} \\ $$$${about}\:{such}\:{triplets}\:{which}\:{satisfy} \\ $$$${your}\:{equation}. \\ $$
Commented by mind is power last updated on 29/Sep/19
suppose x and y coprime ⇒x+y and xy are coprim ⇒xy∣z⇒z=xy and x+y=1 now assume x and y are note coprim let d=gcd(x,y)⇒x=dx′,y=dy′ x′,y′ coprime ⇒d^2 x′y′=d(x′+y′)z ⇒dx′y′=(x′+y′)z x′y′∣z cause x′+y′ and x′y′ are coprime and x′y′∣dx′y′=(x′+y′)z z=x′y′.k ⇒d=k(x′+y′) let S={n∈N n∣d} for all m∈N^∗ we can Whrite m=p+q withe p,q coprim m=1+(m−1) k=m withe m∈S x′+y′=(d/m)∈S (x′,y′)∈{(a,b)∣a+b=(d/m),and gcd(a,b)=1} x=dx′=da y=dx′=db z=kx′y′=mab
$${suppose}\:{x}\:{and}\:{y}\:{coprime} \\ $$$$\Rightarrow{x}+{y}\:{and}\:{xy}\:{are}\:{coprim}\: \\ $$$$\Rightarrow{xy}\mid{z}\Rightarrow{z}={xy}\:{and}\:{x}+{y}=\mathrm{1} \\ $$$${now}\:{assume}\:{x}\:{and}\:{y}\:{are}\:{note}\:{coprim} \\ $$$${let}\:{d}={gcd}\left({x},{y}\right)\Rightarrow{x}={dx}',{y}={dy}' \\ $$$${x}',{y}'\:{coprime} \\ $$$$\Rightarrow{d}^{\mathrm{2}} {x}'{y}'={d}\left({x}'+{y}'\right){z} \\ $$$$\Rightarrow{dx}'{y}'=\left({x}'+{y}'\right){z} \\ $$$${x}'{y}'\mid{z}\:\:{cause}\:{x}'+{y}'\:{and}\:{x}'{y}'\:{are}\:{coprime}\: \\ $$$${and}\:{x}'{y}'\mid{dx}'{y}'=\left({x}'+{y}'\right){z} \\ $$$${z}={x}'{y}'.{k} \\ $$$$\Rightarrow{d}={k}\left({x}'+{y}'\right) \\ $$$${let}\:{S}=\left\{{n}\in\mathbb{N}\:\:\:{n}\mid{d}\right\} \\ $$$${for}\:{all}\:{m}\in\mathbb{N}^{\ast} \:{we}\:{can}\:{Whrite}\:{m}={p}+{q}\:{withe}\:{p},{q}\:{coprim}\:{m}=\mathrm{1}+\left({m}−\mathrm{1}\right) \\ $$$${k}={m}\:{withe}\:{m}\in{S} \\ $$$${x}'+{y}'=\frac{{d}}{{m}}\in{S} \\ $$$$\left({x}',{y}'\right)\in\left\{\left({a},{b}\right)\mid{a}+{b}=\frac{{d}}{{m}},{and}\:{gcd}\left({a},{b}\right)=\mathrm{1}\right\} \\ $$$${x}={dx}'={da} \\ $$$${y}={dx}'={db} \\ $$$${z}={kx}'{y}'={mab} \\ $$$$ \\ $$
Commented by Prithwish sen last updated on 30/Sep/19
Given xy=xz+yz ⇒ 2xy−2xz−2yz = 0 ......(i) and 2xy+2xz+2yz=(x+y+z)^2 −(x^2 +y^2 +z^2 ).....(ii) Adding (i) and (ii) ⇒xy=(((x+y+z)^2 −(x^2 +y^2 +z^2 ))/4) .....(iii) Now there are three possibilities x y z Case I even odd even Case II even even odd CaseIII even even even Now here I am considering only Case I Let x= 2m y= 2n+1 z = 2p ⇒ p = ((m(2n+1))/(2(m+n)+1)) ⇒ 2mp+2np+p = 2mn +m ........(iv) Now from (iii) xy = (((2m+2n+2p+1)^2 −{4m^2 +(2n+1)^2 +4p^2 })/4) = ((8mn+8mp+8np+4m+4p)/4) =( 2mp+2np+p)+2mn+m = 4mn +2m Now ∵ p is an integer ∴ p = n+ ((m−2n^2 −n)/(2m+2n+1)) ⇒m−2n^2 −n=0 ⇒ n = (((√(1+8m))−1)/4) = (((1+4q)−1)/4) = q putting m = 2q^2 +q p =(((2q^2 +q)(2q+1))/(2{(2q^2 +q)+q}+1)) = ((q(2q+1)^2 )/(2q(2q+1)+2q+1)) =q ∴ x = 2q(2q+1) , y =2q+1 and z = 2q q x y z 1 6 3 2 2 20 5 4 3 42 7 6 4 72 9 8 and so on ......... m,n,p ,q are integers Mr. W Sir and Rasheed Sir please give your valuable suggestions.Sir I make the whole thing only dependent on q. By putting q=1,2,... and you get the result.
$$ \\ $$$$\mathrm{Given} \\ $$$$\mathrm{xy}=\mathrm{xz}+\mathrm{yz} \\ $$$$\Rightarrow\:\mathrm{2xy}−\mathrm{2xz}−\mathrm{2yz}\:=\:\mathrm{0}\:……\left(\mathrm{i}\right) \\ $$$$\mathrm{and}\:\mathrm{2xy}+\mathrm{2xz}+\mathrm{2yz}=\left(\mathrm{x}+\mathrm{y}+\mathrm{z}\right)^{\mathrm{2}} −\left(\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} +\mathrm{z}^{\mathrm{2}} \right)…..\left(\mathrm{ii}\right) \\ $$$$\mathrm{Adding}\:\left(\mathrm{i}\right)\:\mathrm{and}\:\left(\mathrm{ii}\right) \\ $$$$\Rightarrow\mathrm{xy}=\frac{\left(\mathrm{x}+\mathrm{y}+\mathrm{z}\right)^{\mathrm{2}} −\left(\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} +\mathrm{z}^{\mathrm{2}} \right)}{\mathrm{4}}\:…..\left(\mathrm{iii}\right) \\ $$$$\boldsymbol{\mathrm{Now}}\:\boldsymbol{\mathrm{there}}\:\boldsymbol{\mathrm{are}}\:\boldsymbol{\mathrm{three}}\:\boldsymbol{\mathrm{possibilities}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\boldsymbol{\mathrm{x}}\:\:\:\:\:\:\:\:\:\:\:\:\boldsymbol{\mathrm{y}}\:\:\:\:\:\:\:\:\:\:\:\boldsymbol{\mathrm{z}} \\ $$$$\boldsymbol{\mathrm{Case}}\:\boldsymbol{\mathrm{I}}\:\:\:\:\:\boldsymbol{\mathrm{even}}\:\:\:\:\:\:\boldsymbol{\mathrm{odd}}\:\:\:\:\:\boldsymbol{\mathrm{even}} \\ $$$$\boldsymbol{\mathrm{Case}}\:\boldsymbol{\mathrm{I}}\mathrm{I}\:\:\:\boldsymbol{\mathrm{even}}\:\:\:\:\:\:\:\:\boldsymbol{\mathrm{even}}\:\:\:\boldsymbol{\mathrm{odd}} \\ $$$$\boldsymbol{\mathrm{CaseIII}}\:\:\:\boldsymbol{\mathrm{even}}\:\:\:\:\:\boldsymbol{\mathrm{even}}\:\:\:\:\boldsymbol{\mathrm{even}} \\ $$$$\boldsymbol{\mathrm{Now}}\:\boldsymbol{\mathrm{here}}\:\boldsymbol{\mathrm{I}}\:\boldsymbol{\mathrm{am}}\:\boldsymbol{\mathrm{considering}}\:\boldsymbol{\mathrm{only}}\:\boldsymbol{\mathrm{Case}}\:\boldsymbol{\mathrm{I}}\: \\ $$$$\mathrm{Let}\:\mathrm{x}=\:\mathrm{2m}\:\:\mathrm{y}=\:\mathrm{2n}+\mathrm{1}\:\:\mathrm{z}\:=\:\mathrm{2p} \\ $$$$\:\:\:\:\:\Rightarrow\:\:\mathrm{p}\:=\:\frac{\mathrm{m}\left(\mathrm{2n}+\mathrm{1}\right)}{\mathrm{2}\left(\mathrm{m}+\mathrm{n}\right)+\mathrm{1}} \\ $$$$\Rightarrow\:\mathrm{2}\boldsymbol{\mathrm{mp}}+\mathrm{2}\boldsymbol{\mathrm{np}}+\boldsymbol{\mathrm{p}}\:=\:\mathrm{2}\boldsymbol{\mathrm{mn}}\:+\boldsymbol{\mathrm{m}}\:……..\left(\boldsymbol{\mathrm{iv}}\right) \\ $$$$\mathrm{Now}\:\mathrm{from}\:\left(\mathrm{iii}\right) \\ $$$$\:\:\boldsymbol{\mathrm{xy}}\:=\:\frac{\left(\mathrm{2}\boldsymbol{\mathrm{m}}+\mathrm{2}\boldsymbol{\mathrm{n}}+\mathrm{2}\boldsymbol{\mathrm{p}}+\mathrm{1}\right)^{\mathrm{2}} −\left\{\mathrm{4}\boldsymbol{\mathrm{m}}^{\mathrm{2}} +\left(\mathrm{2}\boldsymbol{\mathrm{n}}+\mathrm{1}\right)^{\mathrm{2}} +\mathrm{4}\boldsymbol{\mathrm{p}}^{\mathrm{2}} \right\}}{\mathrm{4}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:=\:\frac{\mathrm{8}\boldsymbol{\mathrm{mn}}+\mathrm{8}\boldsymbol{\mathrm{mp}}+\mathrm{8}\boldsymbol{\mathrm{np}}+\mathrm{4}\boldsymbol{\mathrm{m}}+\mathrm{4}\boldsymbol{\mathrm{p}}}{\mathrm{4}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:=\left(\:\mathrm{2}\boldsymbol{\mathrm{mp}}+\mathrm{2}\boldsymbol{\mathrm{np}}+\boldsymbol{\mathrm{p}}\right)+\mathrm{2}\boldsymbol{\mathrm{mn}}+\boldsymbol{\mathrm{m}}\:=\:\mathrm{4}\boldsymbol{\mathrm{mn}}\:+\mathrm{2}\boldsymbol{\mathrm{m}} \\ $$$$\:\:\:\mathrm{Now}\:\because\:\boldsymbol{\mathrm{p}}\:\boldsymbol{\mathrm{is}}\:\boldsymbol{\mathrm{an}}\:\boldsymbol{\mathrm{integer}} \\ $$$$\therefore\:\mathrm{p}\:=\:\mathrm{n}+\:\frac{\mathrm{m}−\mathrm{2n}^{\mathrm{2}} −\mathrm{n}}{\mathrm{2m}+\mathrm{2n}+\mathrm{1}} \\ $$$$\Rightarrow\mathrm{m}−\mathrm{2n}^{\mathrm{2}} −\mathrm{n}=\mathrm{0} \\ $$$$\Rightarrow\:\boldsymbol{\mathrm{n}}\:=\:\frac{\sqrt{\mathrm{1}+\mathrm{8}\boldsymbol{\mathrm{m}}}−\mathrm{1}}{\mathrm{4}}\:\:=\:\frac{\left(\mathrm{1}+\mathrm{4}\boldsymbol{\mathrm{q}}\right)−\mathrm{1}}{\mathrm{4}}\:=\:\boldsymbol{\mathrm{q}}\:\:\boldsymbol{\mathrm{putting}}\:\:\boldsymbol{\mathrm{m}}\:=\:\mathrm{2}\boldsymbol{\mathrm{q}}^{\mathrm{2}} +\boldsymbol{\mathrm{q}} \\ $$$$\mathrm{p}\:=\frac{\left(\mathrm{2}\boldsymbol{\mathrm{q}}^{\mathrm{2}} +\boldsymbol{\mathrm{q}}\right)\left(\mathrm{2}\boldsymbol{\mathrm{q}}+\mathrm{1}\right)}{\mathrm{2}\left\{\left(\mathrm{2}\boldsymbol{\mathrm{q}}^{\mathrm{2}} +\boldsymbol{\mathrm{q}}\right)+\boldsymbol{\mathrm{q}}\right\}+\mathrm{1}}\:=\:\frac{\boldsymbol{\mathrm{q}}\left(\mathrm{2}\boldsymbol{\mathrm{q}}+\mathrm{1}\right)^{\mathrm{2}} }{\mathrm{2}\boldsymbol{\mathrm{q}}\left(\mathrm{2}\boldsymbol{\mathrm{q}}+\mathrm{1}\right)+\mathrm{2}\boldsymbol{\mathrm{q}}+\mathrm{1}} \\ $$$$\:\:=\boldsymbol{\mathrm{q}} \\ $$$$\therefore\:\boldsymbol{\mathrm{x}}\:=\:\mathrm{2}\boldsymbol{\mathrm{q}}\left(\mathrm{2}\boldsymbol{\mathrm{q}}+\mathrm{1}\right)\:,\:\boldsymbol{\mathrm{y}}\:=\mathrm{2}\boldsymbol{\mathrm{q}}+\mathrm{1}\:\boldsymbol{\mathrm{and}}\:\boldsymbol{\mathrm{z}}\:=\:\mathrm{2}\boldsymbol{\mathrm{q}} \\ $$$$\:\:\:\:\:\:\:\boldsymbol{\mathrm{q}}\:\:\:\:\:\:\:\:\boldsymbol{\mathrm{x}}\:\:\:\:\:\:\:\:\:\:\:\boldsymbol{\mathrm{y}}\:\:\:\:\:\:\:\boldsymbol{\mathrm{z}} \\ $$$$\:\:\:\:\:\:\:\:\:\mathrm{1}\:\:\:\:\:\:\:\mathrm{6}\:\:\:\:\:\:\:\:\:\:\mathrm{3}\:\:\:\:\:\:\:\mathrm{2} \\ $$$$\:\:\:\:\:\:\:\:\:\mathrm{2}\:\:\:\:\:\:\mathrm{20}\:\:\:\:\:\:\:\:\:\mathrm{5}\:\:\:\:\:\:\:\:\mathrm{4} \\ $$$$\:\:\:\:\:\:\:\:\:\mathrm{3}\:\:\:\:\:\:\mathrm{42}\:\:\:\:\:\:\:\:\:\mathrm{7}\:\:\:\:\:\:\:\:\mathrm{6} \\ $$$$\:\:\:\:\:\:\:\:\:\:\mathrm{4}\:\:\:\:\:\:\mathrm{72}\:\:\:\:\:\:\:\:\mathrm{9}\:\:\:\:\:\:\:\mathrm{8} \\ $$$$\:\:\:\:\:\:\:\boldsymbol{\mathrm{and}}\:\boldsymbol{\mathrm{so}}\:\boldsymbol{\mathrm{on}}\:……… \\ $$$$\boldsymbol{\mathrm{m}},\boldsymbol{\mathrm{n}},\boldsymbol{\mathrm{p}}\:,\boldsymbol{\mathrm{q}}\:\boldsymbol{\mathrm{are}}\:\boldsymbol{\mathrm{integers}} \\ $$$$\boldsymbol{\mathrm{Mr}}.\:\boldsymbol{\mathrm{W}}\:\boldsymbol{\mathrm{Sir}}\:\boldsymbol{\mathrm{and}}\:\boldsymbol{\mathrm{Rasheed}}\:\boldsymbol{\mathrm{Sir}}\:\boldsymbol{\mathrm{please}}\:\boldsymbol{\mathrm{give}}\:\boldsymbol{\mathrm{your}} \\ $$$$\boldsymbol{\mathrm{valuable}}\:\boldsymbol{\mathrm{suggestions}}.\boldsymbol{\mathrm{Sir}}\:\boldsymbol{\mathrm{I}}\:\:\boldsymbol{\mathrm{make}}\:\boldsymbol{\mathrm{the}}\:\boldsymbol{\mathrm{whole}}\:\boldsymbol{\mathrm{thing}}\: \\ $$$$\boldsymbol{\mathrm{only}}\:\boldsymbol{\mathrm{dependent}}\:\boldsymbol{\mathrm{on}}\:\boldsymbol{\mathrm{q}}.\:\boldsymbol{\mathrm{By}}\:\boldsymbol{\mathrm{putting}}\:\boldsymbol{\mathrm{q}}=\mathrm{1},\mathrm{2},… \\ $$$$\boldsymbol{\mathrm{and}}\:\boldsymbol{\mathrm{you}}\:\boldsymbol{\mathrm{get}}\:\boldsymbol{\mathrm{the}}\:\boldsymbol{\mathrm{result}}. \\ $$
Commented by mr W last updated on 29/Sep/19
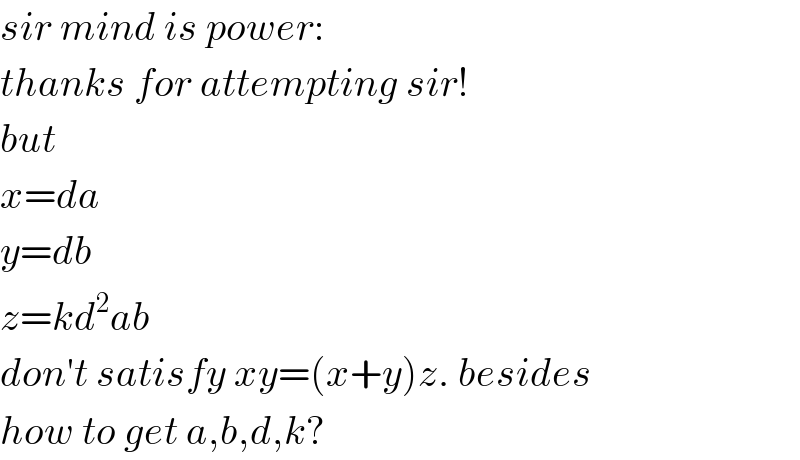
sir mind is power: thanks for attempting sir! but x=da y=db z=kd^2 ab don′t satisfy xy=(x+y)z. besides how to get a,b,d,k?
$${sir}\:{mind}\:{is}\:{power}: \\ $$$${thanks}\:{for}\:{attempting}\:{sir}! \\ $$$${but} \\ $$$${x}={da} \\ $$$${y}={db} \\ $$$${z}={kd}^{\mathrm{2}} {ab} \\ $$$${don}'{t}\:{satisfy}\:{xy}=\left({x}+{y}\right){z}.\:{besides} \\ $$$${how}\:{to}\:{get}\:{a},{b},{d},{k}? \\ $$
Commented by mind is power last updated on 29/Sep/19
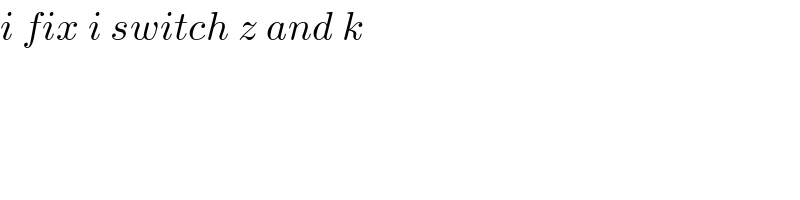
i fix i switch z and k
$${i}\:{fix}\:{i}\:{switch}\:{z}\:{and}\:{k}\: \\ $$
Commented by mr W last updated on 29/Sep/19
prithwish sir: thanks for trying! with x=2m, y=(((√(1+8m))+1)/2),z=2p if you take m=2, y≠integer!
$${prithwish}\:{sir}: \\ $$$${thanks}\:{for}\:{trying}! \\ $$$${with}\:{x}=\mathrm{2}{m},\:{y}=\frac{\sqrt{\mathrm{1}+\mathrm{8}{m}}+\mathrm{1}}{\mathrm{2}},{z}=\mathrm{2}{p} \\ $$$${if}\:{you}\:{take}\:{m}=\mathrm{2},\:{y}\neq{integer}! \\ $$
Commented by mr W last updated on 29/Sep/19
there are three unknowns x,y,z. a general solution must have two parameters in the expression. this is like to find the general integer solution for x+2y+z=7.
$${there}\:{are}\:{three}\:{unknowns}\:{x},{y},{z}. \\ $$$${a}\:{general}\:{solution}\:{must}\:{have}\:{two} \\ $$$${parameters}\:{in}\:{the}\:{expression}.\:{this} \\ $$$${is}\:{like}\:{to}\:{find}\:{the}\:{general}\:{integer} \\ $$$${solution}\:{for}\:{x}+\mathrm{2}{y}+{z}=\mathrm{7}. \\ $$
Commented by mind is power last updated on 29/Sep/19
d given parameter z=dab m divusor of d a and b are such a+b=(d/m) and gcd(a,b)=1 a=(d/m)−b gcd(a,b)=1⇒gcd((d/m),b) soit b is coprime withe (d/(m )) withe b≤(d/m) same withe a coprime withe (d/m) (a,b)∗describe all integer withe a+b=(d/m) withe a and b coprime withe (d/m) exampl a+b=6 (a,b)∈{(1,5) (5,1)} Sorry my english not Good
$${d}\:{given}\:{parameter} \\ $$$${z}={dab} \\ $$$${m}\:{divusor}\:{of}\:{d}\: \\ $$$${a}\:{and}\:{b}\:{are}\:{such}\:{a}+{b}=\frac{{d}}{{m}} \\ $$$${and}\:{gcd}\left({a},{b}\right)=\mathrm{1} \\ $$$${a}=\frac{{d}}{{m}}−{b} \\ $$$${gcd}\left({a},{b}\right)=\mathrm{1}\Rightarrow{gcd}\left(\frac{{d}}{{m}},{b}\right) \\ $$$${soit}\:{b}\:{is}\:{coprime}\:{withe}\:\frac{{d}}{{m}\:}\:{withe}\:{b}\leqslant\frac{{d}}{{m}} \\ $$$${same}\:{withe}\:{a}\:{coprime}\:{withe}\:\frac{{d}}{{m}} \\ $$$$\left({a},{b}\right)\ast{describe}\:{all}\:{integer}\:{withe}\:{a}+{b}=\frac{{d}}{{m}}\:{withe}\:{a}\:{and}\:{b}\:{coprime}\:{withe}\:\frac{{d}}{{m}} \\ $$$${exampl}\:{a}+{b}=\mathrm{6} \\ $$$$\left({a},{b}\right)\in\left\{\left(\mathrm{1},\mathrm{5}\right)\:\left(\mathrm{5},\mathrm{1}\right)\right\} \\ $$$${Sorry}\:{my}\:{english}\:{not}\:{Good} \\ $$$$ \\ $$$$ \\ $$
Answered by Rasheed.Sindhi last updated on 02/Oct/19
I have made a formula which produce a triplet satisfying the given equation for every pair of positive integers: Let p,q∈Z^+ x=p(p+q) y=q(p+q) z=pq We can algebraically prove that (x,y,z)=( p(p+q) , q(p+q) , pq ) is solution of xy=(x+y)z This proves that the solutions are infinite. Claim: ^• If p & q be coprime then (x,y,z) will be primitive triple satisfying xy=(x+y)z ^• The above formulas can produce all the possible primitive solutions ^• Non-primitive solution are merely integral multiples of primitive solutions. Your offered example( (8,24,6)) is is non-primitive solution and its corresponding primitive solution is (4,12,3) and this can be produced by my formulas (put p=1,q=3). How do you see this claim.please give a counter example if you don′t agree.
$${I}\:{have}\:{made}\:{a}\:{formula}\:{which}\:{produce} \\ $$$${a}\:{triplet}\:{satisfying}\:{the}\:{given}\:{equation} \\ $$$${for}\:{every}\:{pair}\:{of}\:{positive}\:{integers}: \\ $$$$\:{Let}\:{p},{q}\in\mathbb{Z}^{+} \\ $$$${x}={p}\left({p}+{q}\right) \\ $$$${y}={q}\left({p}+{q}\right) \\ $$$${z}={pq} \\ $$$${We}\:{can}\:{algebraically}\:{prove}\:{that} \\ $$$$\left({x},{y},{z}\right)=\left(\:{p}\left({p}+{q}\right)\:,\:{q}\left({p}+{q}\right)\:,\:{pq}\:\right)\:{is}\:{solution} \\ $$$${of}\:\:\boldsymbol{{xy}}=\left(\boldsymbol{{x}}+\boldsymbol{{y}}\right)\boldsymbol{{z}} \\ $$$${This}\:{proves}\:{that}\:{the}\:{solutions}\:{are} \\ $$$${infinite}. \\ $$$$\boldsymbol{{Claim}}: \\ $$$$\:^{\bullet} {If}\:\:{p}\:\&\:{q}\:{be}\:{coprime}\:{then} \\ $$$$\left({x},{y},{z}\right)\:{will}\:{be}\:{primitive}\:{triple}\:{satisfying} \\ $$$${xy}=\left({x}+{y}\right){z} \\ $$$$\:^{\bullet} {The}\:{above}\:{formulas}\:{can}\:{produce} \\ $$$$\:\:{all}\:{the}\:{possible}\:{primitive}\:{solutions} \\ $$$$\:^{\bullet} {Non}-{primitive}\:{solution}\:{are}\:{merely} \\ $$$$\:\:{integral}\:{multiples}\:{of}\:{primitive}\:{solutions}. \\ $$$$ \\ $$$$\:\:\:{Your}\:{offered}\:{example}\left(\:\left(\mathrm{8},\mathrm{24},\mathrm{6}\right)\right)\:{is} \\ $$$$\:\:\:\:{is}\:{non}-{primitive}\:{solution}\:{and}\:{its} \\ $$$$\:\:\:\:{corresponding}\:{primitive}\:{solution} \\ $$$$\:\:\:{is}\:\left(\mathrm{4},\mathrm{12},\mathrm{3}\right)\:{and}\:{this}\:{can}\:{be}\:{produced} \\ $$$$\:\:\:{by}\:{my}\:{formulas}\:\left({put}\:{p}=\mathrm{1},{q}=\mathrm{3}\right). \\ $$$${How}\:{do}\:{you}\:{see}\:{this}\:{claim}.{please} \\ $$$${give}\:{a}\:{counter}\:{example}\:{if}\:{you}\:{don}'{t} \\ $$$${agree}. \\ $$
Commented by Prithwish sen last updated on 30/Sep/19
As a whole for the expression xy=(x+y)z x,y,z ∈Z^+ we have Case I x=2q(2q+1) y = (2q+1) z=2q Case II x = 2q y = 2q(2q−1) z = 2q−1 and for Case III no such expression found. x y z q=1 6 3 2 −Case I 2 2 1−Case II q=2 20 5 4 − Case I 4 12 3 − Case II (value of q=3 42 7 6− Case I x and y 6 30 5 − Case II can be q=4 72 9 8 − Case I altered) 8 56 7 − Case II and so on...... It confirms that the expression has infinite number of solutions .
$$\mathrm{As}\:\mathrm{a}\:\mathrm{whole} \\ $$$$\mathrm{for}\:\mathrm{the}\:\mathrm{expression}\: \\ $$$$\boldsymbol{\mathrm{xy}}=\left(\boldsymbol{\mathrm{x}}+\boldsymbol{\mathrm{y}}\right)\boldsymbol{\mathrm{z}}\:\:\:\:\boldsymbol{\mathrm{x}},\boldsymbol{\mathrm{y}},\boldsymbol{\mathrm{z}}\:\in\mathbb{Z}^{+} \\ $$$$\mathrm{we}\:\mathrm{have} \\ $$$$\boldsymbol{\mathrm{Case}}\:\boldsymbol{\mathrm{I}}\:\boldsymbol{\mathrm{x}}=\mathrm{2}\boldsymbol{\mathrm{q}}\left(\mathrm{2}\boldsymbol{\mathrm{q}}+\mathrm{1}\right)\:\:\boldsymbol{\mathrm{y}}\:=\:\left(\mathrm{2}\boldsymbol{\mathrm{q}}+\mathrm{1}\right)\:\:\boldsymbol{\mathrm{z}}=\mathrm{2}\boldsymbol{\mathrm{q}} \\ $$$$\boldsymbol{\mathrm{Case}}\:\boldsymbol{\mathrm{II}}\:\boldsymbol{\mathrm{x}}\:=\:\mathrm{2}\boldsymbol{\mathrm{q}}\:\:\:\:\:\boldsymbol{\mathrm{y}}\:=\:\mathrm{2}\boldsymbol{\mathrm{q}}\left(\mathrm{2}\boldsymbol{\mathrm{q}}−\mathrm{1}\right)\:\:\boldsymbol{\mathrm{z}}\:=\:\mathrm{2}\boldsymbol{\mathrm{q}}−\mathrm{1} \\ $$$$\boldsymbol{\mathrm{and}}\:\boldsymbol{\mathrm{for}}\:\boldsymbol{\mathrm{Case}}\:\boldsymbol{\mathrm{III}}\:\boldsymbol{\mathrm{no}}\:\boldsymbol{\mathrm{such}}\:\boldsymbol{\mathrm{expression}}\:\boldsymbol{\mathrm{found}}. \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\boldsymbol{\mathrm{x}}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\boldsymbol{\mathrm{y}}\:\:\:\:\:\:\:\:\:\:\:\:\boldsymbol{\mathrm{z}} \\ $$$$\boldsymbol{\mathrm{q}}=\mathrm{1}\:\:\:\:\:\:\:\:\:\:\:\mathrm{6}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{3}\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{2}\:\:\:\:−\boldsymbol{\mathrm{Case}}\:\boldsymbol{\mathrm{I}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{2}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{2}\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{1}−\boldsymbol{\mathrm{Case}}\:\boldsymbol{\mathrm{II}} \\ $$$$\boldsymbol{\mathrm{q}}=\mathrm{2}\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{20}\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{5}\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{4}\:\:−\:\boldsymbol{\mathrm{Case}}\:\boldsymbol{\mathrm{I}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{4}\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{12}\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{3}\:−\:\boldsymbol{\mathrm{Case}}\:\boldsymbol{\mathrm{II}}\:\:\:\left(\boldsymbol{\mathrm{value}}\:\boldsymbol{\mathrm{of}}\right. \\ $$$$\boldsymbol{\mathrm{q}}=\mathrm{3}\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{42}\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{7}\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{6}−\:\boldsymbol{\mathrm{Case}}\:\boldsymbol{\mathrm{I}}\:\:\:\:\:\:\:\boldsymbol{\mathrm{x}}\:\boldsymbol{\mathrm{and}}\:\boldsymbol{\mathrm{y}}\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{6}\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{30}\:\:\:\:\:\:\:\:\:\:\:\mathrm{5}\:−\:\boldsymbol{\mathrm{Case}}\:\boldsymbol{\mathrm{II}}\:\:\:\:\boldsymbol{\mathrm{can}}\:\boldsymbol{\mathrm{be}}\: \\ $$$$\left.\boldsymbol{\mathrm{q}}=\mathrm{4}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{72}\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{9}\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{8}\:−\:\boldsymbol{\mathrm{Case}}\:\boldsymbol{\mathrm{I}}\:\:\:\:\:\boldsymbol{\mathrm{altered}}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{8}\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{56}\:\:\:\:\:\:\:\:\:\:\:\mathrm{7}\:−\:\boldsymbol{\mathrm{Case}}\:\boldsymbol{\mathrm{II}} \\ $$$$\boldsymbol{\mathrm{and}}\:\boldsymbol{\mathrm{so}}\:\boldsymbol{\mathrm{on}}…… \\ $$$$\boldsymbol{\mathrm{It}}\:\boldsymbol{\mathrm{confirms}}\:\boldsymbol{\mathrm{that}}\:\boldsymbol{\mathrm{the}}\:\boldsymbol{\mathrm{expression}}\:\boldsymbol{\mathrm{has}}\:\boldsymbol{\mathrm{infinite}} \\ $$$$\boldsymbol{\mathrm{number}}\:\boldsymbol{\mathrm{of}}\:\boldsymbol{\mathrm{solutions}}\:. \\ $$
Commented by Rasheed.Sindhi last updated on 03/Oct/19
Sir how can we prove that the above formulas can produce all possible solutions? & What′s the sourse of problem?
$${Sir}\:{how}\:{can}\:{we}\:{prove}\:{that}\:{the}\:{above} \\ $$$${formulas}\:{can}\:{produce}\:\boldsymbol{{all}}\:{possible} \\ $$$${solutions}? \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\& \\ $$$${What}'{s}\:{the}\:{sourse}\:{of}\:{problem}? \\ $$
Commented by mr W last updated on 29/Sep/19
that′s a nice way sir! from given p and q we can construct a solution. but there could be more than just one solution for given p, q. e.g. z=6 (x,y)=(10,15) is a solution,but (8,24) is also a solution.
$${that}'{s}\:{a}\:{nice}\:{way}\:{sir}! \\ $$$${from}\:{given}\:{p}\:{and}\:{q}\:{we}\:{can}\:{construct} \\ $$$${a}\:{solution}.\:{but}\:{there}\:{could}\:{be}\:{more} \\ $$$${than}\:{just}\:{one}\:{solution}\:{for}\:{given}\:{p},\:{q}. \\ $$$${e}.{g}.\:{z}=\mathrm{6} \\ $$$$\left({x},{y}\right)=\left(\mathrm{10},\mathrm{15}\right)\:{is}\:{a}\:{solution},{but} \\ $$$$\left(\mathrm{8},\mathrm{24}\right)\:{is}\:{also}\:{a}\:{solution}. \\ $$
Commented by Rasheed.Sindhi last updated on 30/Sep/19
Yes sir,the formula doesn′t cover all possible solutions.However it assures that there are infinite solutions. For composite z, the formula can give multiple solutions. For example z=6⇒pq=6⇒(p,q)=(1,6) or (2,3) ⇒(x,y^� ,z)=(7,42,6) or (10,15,6). But afterall the formula doesn′t cover all possible solutions.
$${Yes}\:{sir},{the}\:{formula}\:{doesn}'{t}\:{cover}\:{all} \\ $$$${possible}\:{solutions}.{However}\:{it}\:{assures} \\ $$$${that}\:{there}\:{are}\:{infinite}\:{solutions}. \\ $$$${For}\:{composite}\:{z},\:{the}\:{formula}\:{can} \\ $$$${give}\:{multiple}\:{solutions}.\:{For}\:{example} \\ $$$${z}=\mathrm{6}\Rightarrow{pq}=\mathrm{6}\Rightarrow\left({p},{q}\right)=\left(\mathrm{1},\mathrm{6}\right)\:{or}\:\left(\mathrm{2},\mathrm{3}\right) \\ $$$$\Rightarrow\left({x},\bar {{y}},{z}\right)=\left(\mathrm{7},\mathrm{42},\mathrm{6}\right)\:{or}\:\left(\mathrm{10},\mathrm{15},\mathrm{6}\right). \\ $$$${But}\:{afterall}\:{the}\:{formula}\:{doesn}'{t} \\ $$$${cover}\:{all}\:{possible}\:{solutions}. \\ $$
Commented by Prithwish sen last updated on 30/Sep/19
Mr W. Sir please see my solution I have fix my error. I think I get a general formula with a single parameter q.
$$\mathrm{Mr}\:\mathrm{W}.\:\mathrm{Sir}\:\mathrm{please}\:\mathrm{see}\:\mathrm{my}\:\mathrm{solution}\:\mathrm{I}\:\mathrm{have}\: \\ $$$$\mathrm{fix}\:\mathrm{my}\:\mathrm{error}.\:\mathrm{I}\:\mathrm{think}\:\mathrm{I}\:\mathrm{get}\:\mathrm{a}\:\mathrm{general}\:\mathrm{formula}\:\: \\ $$$$\mathrm{with}\:\mathrm{a}\:\mathrm{single}\:\mathrm{parameter}\:\boldsymbol{\mathrm{q}}. \\ $$$$ \\ $$
Commented by Prithwish sen last updated on 30/Sep/19
Sir please waiting for your response.
$$\mathrm{Sir}\:\mathrm{please}\:\mathrm{waiting}\:\mathrm{for}\:\mathrm{your}\:\mathrm{response}. \\ $$
Commented by mr W last updated on 30/Sep/19
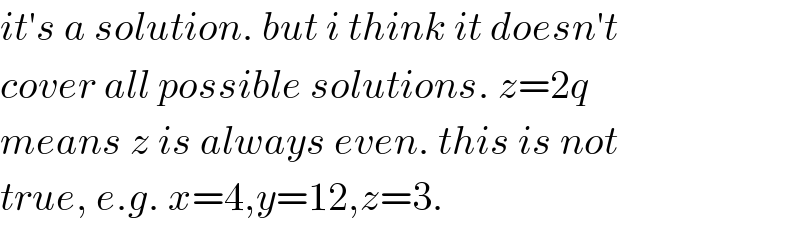
it′s a solution. but i think it doesn′t cover all possible solutions. z=2q means z is always even. this is not true, e.g. x=4,y=12,z=3.
$${it}'{s}\:{a}\:{solution}.\:{but}\:{i}\:{think}\:{it}\:{doesn}'{t} \\ $$$${cover}\:{all}\:{possible}\:{solutions}.\:{z}=\mathrm{2}{q} \\ $$$${means}\:{z}\:{is}\:{always}\:{even}.\:{this}\:{is}\:{not} \\ $$$${true},\:{e}.{g}.\:{x}=\mathrm{4},{y}=\mathrm{12},{z}=\mathrm{3}. \\ $$
Commented by Rasheed.Sindhi last updated on 30/Sep/19
Prithwish sen sir Your ′product machine′ is wonderful! It produces solutions successfully. But there are some solutions which can′t be produced by your machine. Whereas mr W sir demands such machine which can produce all possible solutions! For example for z=6 your machine (farmula) produces single triplet (solution):(42,7,6) by putting q=3. Whereas many other triplets(which aren′t come from your machine) satisfy the equation xy=(x+y)z. Such as (10,15,6) etc (Can this be produced by your formula?)
$${Prithwish}\:{sen}\:{sir} \\ $$$${Your}\:'{product}\:{machine}'\:{is}\:{wonderful}! \\ $$$${It}\:{produces}\:{solutions}\:{successfully}. \\ $$$${But}\:{there}\:{are}\:{some}\:{solutions}\:{which} \\ $$$${can}'{t}\:{be}\:{produced}\:{by}\:{your}\:{machine}. \\ $$$${Whereas}\:{mr}\:{W}\:{sir}\:{demands}\:{such} \\ $$$${machine}\:{which}\:{can}\:{produce}\:{all}\:{possible} \\ $$$${solutions}! \\ $$$${For}\:{example}\:{for}\:{z}=\mathrm{6}\:{your}\:{machine} \\ $$$$\left({farmula}\right)\:{produces}\:{single}\:{triplet} \\ $$$$\left({solution}\right):\left(\mathrm{42},\mathrm{7},\mathrm{6}\right)\:{by}\:{putting}\:{q}=\mathrm{3}. \\ $$$${Whereas}\:{many}\:{other}\:{triplets}\left({which}\right. \\ $$$$\left.{aren}'{t}\:{come}\:{from}\:{your}\:{machine}\right) \\ $$$${satisfy}\:{the}\:{equation}\:\boldsymbol{{xy}}=\left(\boldsymbol{{x}}+\boldsymbol{{y}}\right)\boldsymbol{{z}}. \\ $$$${Such}\:{as}\:\left(\mathrm{10},\mathrm{15},\mathrm{6}\right)\:{etc}\:\left({Can}\:{this}\:{be}\right. \\ $$$$\left.{produced}\:{by}\:{your}\:{formula}?\right) \\ $$
Commented by Prithwish sen last updated on 30/Sep/19
Thank you sir.
$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{sir}. \\ $$
Commented by mr W last updated on 30/Sep/19
thank you all sirs!
$${thank}\:{you}\:{all}\:{sirs}! \\ $$
Commented by Prithwish sen last updated on 01/Oct/19
Thank you sir.
$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{sir}. \\ $$$$ \\ $$
Commented by Rasheed.Sindhi last updated on 01/Oct/19
An Equivalent Expression of xy=(x+y)z. xy=(x+y)z⇒xy−yz−zx=0 ⇒xy+y(−z)+(−z)x=0 Now, (x+y−z)^2 =x^2 +y^2 +z^2 +2(xy+y(−z)+(−z)x) =x^2 +y^2 +z^2 +2(0) x^2 +y^2 +z^2 =(x+y−z)^2 This is equivalent to xy=(x+y)z i-e solutions of both are same. Note:The idea raised in my mind after seeing a comment of Prithwish sen sir.
$${An}\:\mathcal{E}{quivalent}\:\mathcal{E}{xpression}\:{of}\: \\ $$$$\boldsymbol{{xy}}=\left(\boldsymbol{{x}}+\boldsymbol{{y}}\right)\boldsymbol{{z}}. \\ $$$$\boldsymbol{{xy}}=\left(\boldsymbol{{x}}+\boldsymbol{{y}}\right)\boldsymbol{{z}}\Rightarrow\boldsymbol{{xy}}−\boldsymbol{{yz}}−\boldsymbol{{zx}}=\mathrm{0} \\ $$$$\Rightarrow\boldsymbol{{xy}}+\boldsymbol{{y}}\left(−\boldsymbol{{z}}\right)+\left(−\boldsymbol{{z}}\right)\boldsymbol{{x}}=\mathrm{0} \\ $$$${Now}, \\ $$$$\:\left(\boldsymbol{{x}}+\boldsymbol{{y}}−\boldsymbol{{z}}\right)^{\mathrm{2}} =\boldsymbol{{x}}^{\mathrm{2}} +\boldsymbol{{y}}^{\mathrm{2}} +\boldsymbol{{z}}^{\mathrm{2}} +\mathrm{2}\left(\boldsymbol{{xy}}+\boldsymbol{{y}}\left(−\boldsymbol{{z}}\right)+\left(−\boldsymbol{{z}}\right)\boldsymbol{{x}}\right) \\ $$$$\:\:\:\:\:\:\:=\boldsymbol{{x}}^{\mathrm{2}} +\boldsymbol{{y}}^{\mathrm{2}} +\boldsymbol{{z}}^{\mathrm{2}} +\mathrm{2}\left(\mathrm{0}\right) \\ $$$$\boldsymbol{{x}}^{\mathrm{2}} +\boldsymbol{{y}}^{\mathrm{2}} +\boldsymbol{{z}}^{\mathrm{2}} =\left(\boldsymbol{{x}}+\boldsymbol{{y}}−\boldsymbol{{z}}\right)^{\mathrm{2}} \\ $$$$\boldsymbol{{This}}\:\boldsymbol{{is}}\:\boldsymbol{{equivalent}}\:\boldsymbol{{to}}\:\boldsymbol{{xy}}=\left(\boldsymbol{{x}}+\boldsymbol{{y}}\right)\boldsymbol{{z}} \\ $$$$\boldsymbol{{i}}-\boldsymbol{{e}}\:\boldsymbol{{solutions}}\:\boldsymbol{{of}}\:\boldsymbol{{both}}\:\boldsymbol{{are}}\:\boldsymbol{{same}}. \\ $$$${Note}:{The}\:{idea}\:{raised}\:{in}\:{my}\:{mind}\:{after} \\ $$$${seeing}\:{a}\:{comment}\:{of}\:{Prithwish}\:{sen}\:{sir}. \\ $$
Commented by Rasheed.Sindhi last updated on 03/Oct/19
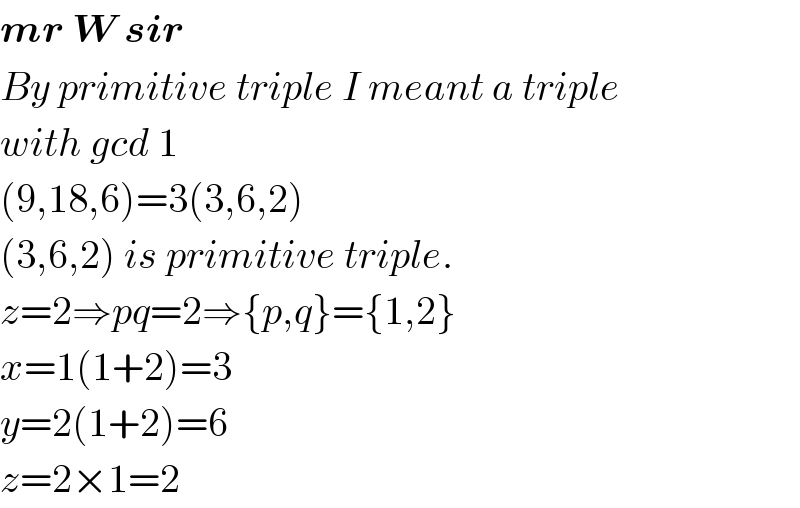
mr W sir By primitive triple I meant a triple with gcd 1 (9,18,6)=3(3,6,2) (3,6,2) is primitive triple. z=2⇒pq=2⇒{p,q}={1,2} x=1(1+2)=3 y=2(1+2)=6 z=2×1=2
$$\boldsymbol{{mr}}\:\boldsymbol{{W}}\:\boldsymbol{{sir}} \\ $$$${By}\:{primitive}\:{triple}\:{I}\:{meant}\:{a}\:{triple} \\ $$$${with}\:{gcd}\:\mathrm{1} \\ $$$$\left(\mathrm{9},\mathrm{18},\mathrm{6}\right)=\mathrm{3}\left(\mathrm{3},\mathrm{6},\mathrm{2}\right) \\ $$$$\left(\mathrm{3},\mathrm{6},\mathrm{2}\right)\:{is}\:{primitive}\:{triple}. \\ $$$${z}=\mathrm{2}\Rightarrow{pq}=\mathrm{2}\Rightarrow\left\{{p},{q}\right\}=\left\{\mathrm{1},\mathrm{2}\right\} \\ $$$${x}=\mathrm{1}\left(\mathrm{1}+\mathrm{2}\right)=\mathrm{3} \\ $$$${y}=\mathrm{2}\left(\mathrm{1}+\mathrm{2}\right)=\mathrm{6} \\ $$$${z}=\mathrm{2}×\mathrm{1}=\mathrm{2} \\ $$
Commented by mr W last updated on 03/Oct/19
i created this question because i became unsure to my solution to Q10944.
$${i}\:{created}\:{this}\:{question}\:{because}\:{i}\:{became} \\ $$$${unsure}\:{to}\:{my}\:{solution}\:{to}\:{Q}\mathrm{10944}. \\ $$
Commented by Rasheed.Sindhi last updated on 02/Oct/19
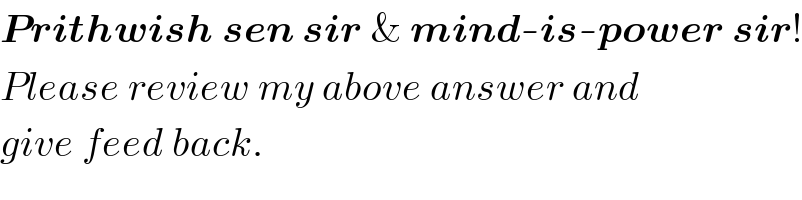
Prithwish sen sir & mind-is-power sir! Please review my above answer and give feed back.
$$\boldsymbol{{Prithwish}}\:\boldsymbol{{sen}}\:\boldsymbol{{sir}}\:\&\:\boldsymbol{{mind}}-\boldsymbol{{is}}-\boldsymbol{{power}}\:\boldsymbol{{sir}}! \\ $$$${Please}\:{review}\:{my}\:{above}\:{answer}\:{and} \\ $$$${give}\:{feed}\:{back}. \\ $$
Commented by Rasheed.Sindhi last updated on 02/Oct/19
Sir mr W Please review my above answer.I′ve added some material in red lines . I′m anxiously waiting for your response.
$$\boldsymbol{{Sir}}\:\boldsymbol{{mr}}\:\boldsymbol{{W}} \\ $$$${Please}\:{review}\:{my}\:{above}\:{answer}.{I}'{ve} \\ $$$${added}\:{some}\:{material}\:{in}\:{red}\:{lines}\:. \\ $$$${I}'{m}\:{anxiously}\:{waiting}\:{for}\:{your}\:{response}. \\ $$
Commented by mr W last updated on 02/Oct/19
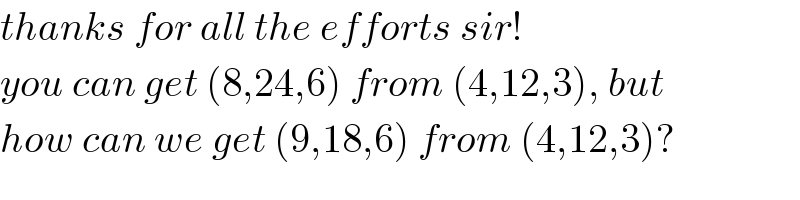
thanks for all the efforts sir! you can get (8,24,6) from (4,12,3), but how can we get (9,18,6) from (4,12,3)?
$${thanks}\:{for}\:{all}\:{the}\:{efforts}\:{sir}! \\ $$$${you}\:{can}\:{get}\:\left(\mathrm{8},\mathrm{24},\mathrm{6}\right)\:{from}\:\left(\mathrm{4},\mathrm{12},\mathrm{3}\right),\:{but} \\ $$$${how}\:{can}\:{we}\:{get}\:\left(\mathrm{9},\mathrm{18},\mathrm{6}\right)\:{from}\:\left(\mathrm{4},\mathrm{12},\mathrm{3}\right)? \\ $$
Commented by mr W last updated on 03/Oct/19
now i see. thanks sir! the task is now how to express x and y x=f(p,q) y=g(p,q) z=pq such that x and y cover all possibilities. can we find the f(p,q) and g(p,q)?
$${now}\:{i}\:{see}.\:{thanks}\:{sir}! \\ $$$${the}\:{task}\:{is}\:{now}\:{how}\:{to}\:{express}\:{x}\:{and}\:{y} \\ $$$${x}={f}\left({p},{q}\right) \\ $$$${y}={g}\left({p},{q}\right) \\ $$$${z}={pq} \\ $$$${such}\:{that}\:{x}\:{and}\:{y}\:{cover}\:{all}\:{possibilities}. \\ $$$${can}\:{we}\:{find}\:{the}\:{f}\left({p},{q}\right)\:{and}\:{g}\left({p},{q}\right)? \\ $$
Commented by Rasheed.Sindhi last updated on 03/Oct/19
x=f(p,q)=p(p+q) y=g(p,q)=q(p+q) z=h(p,q)=pq For a given z=12(say) z=12⇒pq=12 (for primitive solutions take p & q coprime) (p,q)= (1,12),(3,4),(4,3),(12,1) (1,12): x=1(1+12)=13 y=12(1+12)=156 z=12 All possible primitive solutions with z=12 are: (13,156,12),(21,28,12), (28,21,12) & (156,13,12) For primitive solutions with z=12 x & y can′t take other values (If my claim is right).If x & y have values other than above, then the that solution must be non-primitive. please try counter example:(a,b,12) such that gcd(a,b,12)=1 and ab=12(a+b)
$${x}={f}\left({p},{q}\right)={p}\left({p}+{q}\right) \\ $$$${y}={g}\left({p},{q}\right)={q}\left({p}+{q}\right) \\ $$$${z}={h}\left({p},{q}\right)={pq} \\ $$$${For}\:{a}\:{given}\:{z}=\mathrm{12}\left({say}\right) \\ $$$${z}=\mathrm{12}\Rightarrow{pq}=\mathrm{12}\:\left({for}\:{primitive}\:{solutions}\right. \\ $$$$\left.{take}\:{p}\:\&\:{q}\:{coprime}\right) \\ $$$$\left({p},{q}\right)=\:\left(\mathrm{1},\mathrm{12}\right),\left(\mathrm{3},\mathrm{4}\right),\left(\mathrm{4},\mathrm{3}\right),\left(\mathrm{12},\mathrm{1}\right) \\ $$$$\left(\mathrm{1},\mathrm{12}\right):\:{x}=\mathrm{1}\left(\mathrm{1}+\mathrm{12}\right)=\mathrm{13} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{y}=\mathrm{12}\left(\mathrm{1}+\mathrm{12}\right)=\mathrm{156} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{z}=\mathrm{12} \\ $$$${All}\:{possible}\:{primitive}\:{solutions}\:{with} \\ $$$${z}=\mathrm{12}\:{are}:\:\left(\mathrm{13},\mathrm{156},\mathrm{12}\right),\left(\mathrm{21},\mathrm{28},\mathrm{12}\right), \\ $$$$\left(\mathrm{28},\mathrm{21},\mathrm{12}\right)\:\&\:\left(\mathrm{156},\mathrm{13},\mathrm{12}\right) \\ $$$${For}\:{primitive}\:{solutions}\:{with}\:{z}=\mathrm{12} \\ $$$${x}\:\&\:{y}\:{can}'{t}\:{take}\:{other}\:{values}\: \\ $$$$\left({If}\:{my}\:{claim}\:{is}\:{right}\right).{If}\:{x}\:\&\:{y}\:{have} \\ $$$${values}\:{other}\:{than}\:{above},\:{then}\:{the} \\ $$$${that}\:{solution}\:{must}\:{be}\:{non}-{primitive}. \\ $$$${please}\:{try}\:{counter}\:{example}:\left({a},{b},\mathrm{12}\right) \\ $$$${such}\:{that}\:{gcd}\left({a},{b},\mathrm{12}\right)=\mathrm{1}\:{and}\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{ab}=\mathrm{12}\left({a}+{b}\right) \\ $$
Commented by mr W last updated on 03/Oct/19
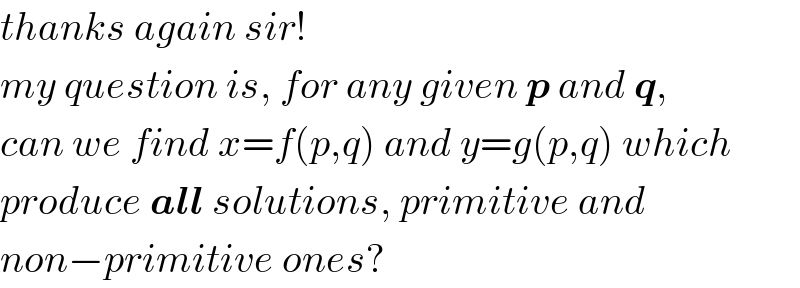
thanks again sir! my question is, for any given p and q, can we find x=f(p,q) and y=g(p,q) which produce all solutions, primitive and non−primitive ones?
$${thanks}\:{again}\:{sir}! \\ $$$${my}\:{question}\:{is},\:{for}\:{any}\:{given}\:\boldsymbol{{p}}\:{and}\:\boldsymbol{{q}}, \\ $$$${can}\:{we}\:{find}\:{x}={f}\left({p},{q}\right)\:{and}\:{y}={g}\left({p},{q}\right)\:{which} \\ $$$${produce}\:\boldsymbol{{all}}\:{solutions},\:{primitive}\:{and} \\ $$$${non}−{primitive}\:{ones}? \\ $$
Commented by Rasheed.Sindhi last updated on 03/Oct/19
By adding one additional parameter k: x=kp(p+q) y=kq(p+q) z=kpq p & q being coprime and k any positive integer. I think sir these formulas can cover all primitive as well as non-primitive solutions.
$${By}\:{adding}\:{one}\:{additional}\:{parameter}\:{k}: \\ $$$${x}={kp}\left({p}+{q}\right) \\ $$$${y}={kq}\left({p}+{q}\right) \\ $$$${z}={kpq} \\ $$$${p}\:\&\:{q}\:{being}\:{coprime}\:{and}\:{k}\:{any}\:{positive} \\ $$$${integer}.\:{I}\:{think}\:{sir}\:{these}\:{formulas} \\ $$$${can}\:{cover}\:\boldsymbol{{all}}\:{primitive}\:{as}\:{well}\:{as} \\ $$$${non}-{primitive}\:{solutions}. \\ $$
Commented by mr W last updated on 03/Oct/19
big big thanks sir!
$${big}\:{big}\:{thanks}\:{sir}! \\ $$
Answered by Rasheed.Sindhi last updated on 02/Oct/19
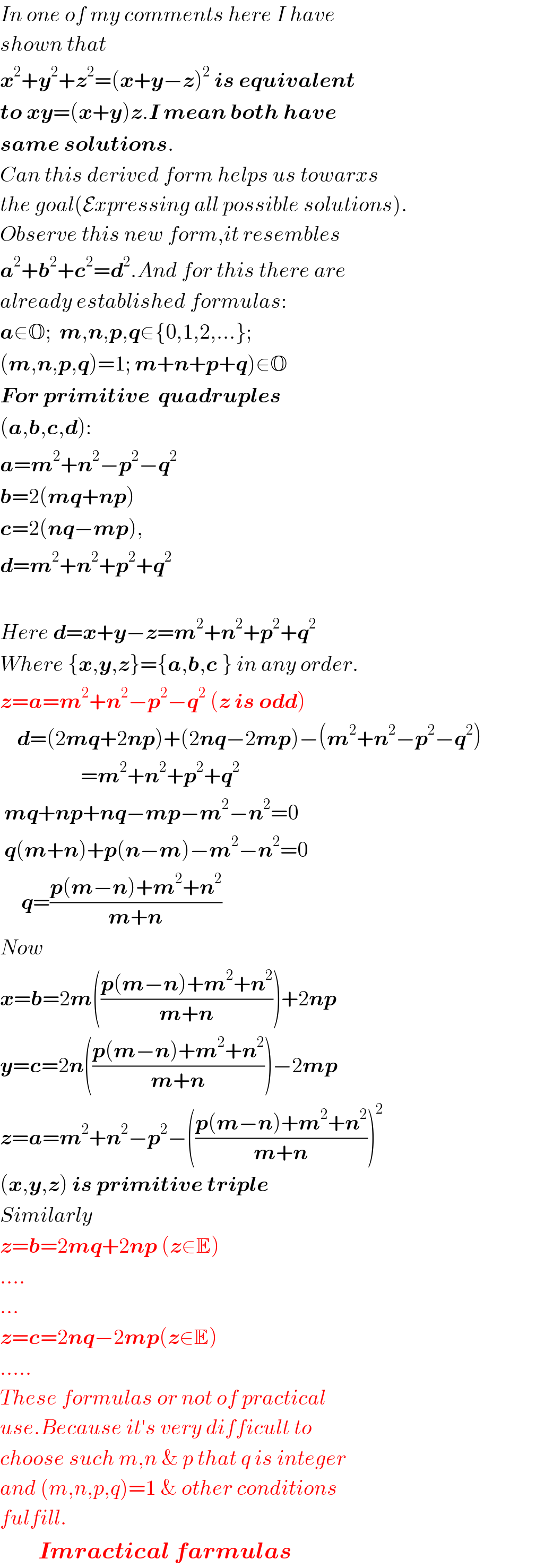
In one of my comments here I have shown that x^2 +y^2 +z^2 =(x+y−z)^2 is equivalent to xy=(x+y)z.I mean both have same solutions. Can this derived form helps us towarxs the goal(Expressing all possible solutions). Observe this new form,it resembles a^2 +b^2 +c^2 =d^2 .And for this there are already established formulas: a∈O; m,n,p,q∈{0,1,2,...}; (m,n,p,q)=1; m+n+p+q)∈O For primitive quadruples (a,b,c,d): a=m^2 +n^2 −p^2 −q^2 b=2(mq+np) c=2(nq−mp), d=m^2 +n^2 +p^2 +q^2 Here d=x+y−z=m^2 +n^2 +p^2 +q^2 Where {x,y,z}={a,b,c } in any order. z=a=m^2 +n^2 −p^2 −q^2 (z is odd) d=(2mq+2np)+(2nq−2mp)−(m^2 +n^2 −p^2 −q^2 ) =m^2 +n^2 +p^2 +q^2 mq+np+nq−mp−m^2 −n^2 =0 q(m+n)+p(n−m)−m^2 −n^2 =0 q=((p(m−n)+m^2 +n^2 )/(m+n)) Now x=b=2m(((p(m−n)+m^2 +n^2 )/(m+n)))+2np y=c=2n(((p(m−n)+m^2 +n^2 )/(m+n)))−2mp z=a=m^2 +n^2 −p^2 −(((p(m−n)+m^2 +n^2 )/(m+n)))^2 (x,y,z) is primitive triple Similarly z=b=2mq+2np (z∈E) .... ... z=c=2nq−2mp(z∈E) ..... These formulas or not of practical use.Because it′s very difficult to choose such m,n & p that q is integer and (m,n,p,q)=1 & other conditions fulfill. Imractical farmulas
$${In}\:{one}\:{of}\:{my}\:{comments}\:{here}\:{I}\:{have} \\ $$$${shown}\:{that}\: \\ $$$$\boldsymbol{{x}}^{\mathrm{2}} +\boldsymbol{{y}}^{\mathrm{2}} +\boldsymbol{{z}}^{\mathrm{2}} =\left(\boldsymbol{{x}}+\boldsymbol{{y}}−\boldsymbol{{z}}\right)^{\mathrm{2}} \:\boldsymbol{{is}}\:\boldsymbol{{equivalent}} \\ $$$$\boldsymbol{{to}}\:\boldsymbol{{xy}}=\left(\boldsymbol{{x}}+\boldsymbol{{y}}\right)\boldsymbol{{z}}.\boldsymbol{{I}}\:\boldsymbol{{mean}}\:\boldsymbol{{both}}\:\boldsymbol{{have}} \\ $$$$\boldsymbol{{same}}\:\boldsymbol{{solutions}}. \\ $$$${Can}\:{this}\:{derived}\:{form}\:{helps}\:{us}\:{towarxs} \\ $$$${the}\:{goal}\left(\mathcal{E}{xpressing}\:{all}\:{possible}\:{solutions}\right). \\ $$$${Observe}\:{this}\:{new}\:{form},{it}\:{resembles} \\ $$$$\boldsymbol{{a}}^{\mathrm{2}} +\boldsymbol{{b}}^{\mathrm{2}} +\boldsymbol{{c}}^{\mathrm{2}} =\boldsymbol{{d}}^{\mathrm{2}} .{And}\:{for}\:{this}\:{there}\:{are} \\ $$$${already}\:{established}\:{formulas}: \\ $$$$\boldsymbol{{a}}\in\mathbb{O};\:\:\boldsymbol{{m}},\boldsymbol{{n}},\boldsymbol{{p}},\boldsymbol{{q}}\in\left\{\mathrm{0},\mathrm{1},\mathrm{2},…\right\}; \\ $$$$\left.\left(\boldsymbol{{m}},\boldsymbol{{n}},\boldsymbol{{p}},\boldsymbol{{q}}\right)=\mathrm{1};\:\boldsymbol{{m}}+\boldsymbol{{n}}+\boldsymbol{{p}}+\boldsymbol{{q}}\right)\in\mathbb{O} \\ $$$$\boldsymbol{{For}}\:\boldsymbol{{primitive}}\:\:\boldsymbol{{quadruples}}\: \\ $$$$\left(\boldsymbol{{a}},\boldsymbol{{b}},\boldsymbol{{c}},\boldsymbol{{d}}\right): \\ $$$$\boldsymbol{{a}}=\boldsymbol{{m}}^{\mathrm{2}} +\boldsymbol{{n}}^{\mathrm{2}} −\boldsymbol{{p}}^{\mathrm{2}} −\boldsymbol{{q}}^{\mathrm{2}} \\ $$$$\boldsymbol{{b}}=\mathrm{2}\left(\boldsymbol{{mq}}+\boldsymbol{{np}}\right) \\ $$$$\boldsymbol{{c}}=\mathrm{2}\left(\boldsymbol{{nq}}−\boldsymbol{{mp}}\right), \\ $$$$\boldsymbol{{d}}=\boldsymbol{{m}}^{\mathrm{2}} +\boldsymbol{{n}}^{\mathrm{2}} +\boldsymbol{{p}}^{\mathrm{2}} +\boldsymbol{{q}}^{\mathrm{2}} \\ $$$$ \\ $$$${Here}\:\boldsymbol{{d}}=\boldsymbol{{x}}+\boldsymbol{{y}}−\boldsymbol{{z}}=\boldsymbol{{m}}^{\mathrm{2}} +\boldsymbol{{n}}^{\mathrm{2}} +\boldsymbol{{p}}^{\mathrm{2}} +\boldsymbol{{q}}^{\mathrm{2}} \\ $$$${Where}\:\left\{\boldsymbol{{x}},\boldsymbol{{y}},\boldsymbol{{z}}\right\}=\left\{\boldsymbol{{a}},\boldsymbol{{b}},\boldsymbol{{c}}\:\right\}\:{in}\:{any}\:{order}. \\ $$$$\boldsymbol{{z}}=\boldsymbol{{a}}=\boldsymbol{{m}}^{\mathrm{2}} +\boldsymbol{{n}}^{\mathrm{2}} −\boldsymbol{{p}}^{\mathrm{2}} −\boldsymbol{{q}}^{\mathrm{2}} \:\left(\boldsymbol{{z}}\:\boldsymbol{{is}}\:\boldsymbol{{odd}}\right) \\ $$$$\:\:\:\:\boldsymbol{{d}}=\left(\mathrm{2}\boldsymbol{{mq}}+\mathrm{2}\boldsymbol{{np}}\right)+\left(\mathrm{2}\boldsymbol{{nq}}−\mathrm{2}\boldsymbol{{mp}}\right)−\left(\boldsymbol{{m}}^{\mathrm{2}} +\boldsymbol{{n}}^{\mathrm{2}} −\boldsymbol{{p}}^{\mathrm{2}} −\boldsymbol{{q}}^{\mathrm{2}} \right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\boldsymbol{{m}}^{\mathrm{2}} +\boldsymbol{{n}}^{\mathrm{2}} +\boldsymbol{{p}}^{\mathrm{2}} +\boldsymbol{{q}}^{\mathrm{2}} \\ $$$$\:\boldsymbol{{mq}}+\boldsymbol{{np}}+\boldsymbol{{nq}}−\boldsymbol{{mp}}−\boldsymbol{{m}}^{\mathrm{2}} −\boldsymbol{{n}}^{\mathrm{2}} =\mathrm{0} \\ $$$$\:\boldsymbol{{q}}\left(\boldsymbol{{m}}+\boldsymbol{{n}}\right)+\boldsymbol{{p}}\left(\boldsymbol{{n}}−\boldsymbol{{m}}\right)−\boldsymbol{{m}}^{\mathrm{2}} −\boldsymbol{{n}}^{\mathrm{2}} =\mathrm{0} \\ $$$$\:\:\:\:\:\boldsymbol{{q}}=\frac{\boldsymbol{{p}}\left(\boldsymbol{{m}}−\boldsymbol{{n}}\right)+\boldsymbol{{m}}^{\mathrm{2}} +\boldsymbol{{n}}^{\mathrm{2}} }{\boldsymbol{{m}}+\boldsymbol{{n}}} \\ $$$${Now} \\ $$$$\boldsymbol{{x}}=\boldsymbol{{b}}=\mathrm{2}\boldsymbol{{m}}\left(\frac{\boldsymbol{{p}}\left(\boldsymbol{{m}}−\boldsymbol{{n}}\right)+\boldsymbol{{m}}^{\mathrm{2}} +\boldsymbol{{n}}^{\mathrm{2}} }{\boldsymbol{{m}}+\boldsymbol{{n}}}\right)+\mathrm{2}\boldsymbol{{np}} \\ $$$$\boldsymbol{{y}}=\boldsymbol{{c}}=\mathrm{2}\boldsymbol{{n}}\left(\frac{\boldsymbol{{p}}\left(\boldsymbol{{m}}−\boldsymbol{{n}}\right)+\boldsymbol{{m}}^{\mathrm{2}} +\boldsymbol{{n}}^{\mathrm{2}} }{\boldsymbol{{m}}+\boldsymbol{{n}}}\right)−\mathrm{2}\boldsymbol{{mp}} \\ $$$$\boldsymbol{{z}}=\boldsymbol{{a}}=\boldsymbol{{m}}^{\mathrm{2}} +\boldsymbol{{n}}^{\mathrm{2}} −\boldsymbol{{p}}^{\mathrm{2}} −\left(\frac{\boldsymbol{{p}}\left(\boldsymbol{{m}}−\boldsymbol{{n}}\right)+\boldsymbol{{m}}^{\mathrm{2}} +\boldsymbol{{n}}^{\mathrm{2}} }{\boldsymbol{{m}}+\boldsymbol{{n}}}\right)^{\mathrm{2}} \:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\: \\ $$$$\left(\boldsymbol{{x}},\boldsymbol{{y}},\boldsymbol{{z}}\right)\:\boldsymbol{{is}}\:\boldsymbol{{primitive}}\:\boldsymbol{{triple}} \\ $$$${Similarly} \\ $$$$\boldsymbol{{z}}=\boldsymbol{{b}}=\mathrm{2}\boldsymbol{{mq}}+\mathrm{2}\boldsymbol{{np}}\:\left(\boldsymbol{{z}}\in\mathbb{E}\right) \\ $$$$…. \\ $$$$… \\ $$$$\boldsymbol{{z}}=\boldsymbol{{c}}=\mathrm{2}\boldsymbol{{nq}}−\mathrm{2}\boldsymbol{{mp}}\left(\boldsymbol{{z}}\in\mathbb{E}\right) \\ $$$$….. \\ $$$${These}\:{formulas}\:{or}\:{not}\:{of}\:{practical} \\ $$$${use}.{Because}\:{it}'{s}\:{very}\:{difficult}\:{to} \\ $$$${choose}\:{such}\:{m},{n}\:\&\:{p}\:{that}\:{q}\:{is}\:{integer} \\ $$$${and}\:\left({m},{n},{p},{q}\right)=\mathrm{1}\:\&\:{other}\:{conditions} \\ $$$${fulfill}. \\ $$$$\:\:\:\:\:\:\:\:\:\boldsymbol{{Imractical}}\:\boldsymbol{{farmulas}} \\ $$
Commented by Rasheed.Sindhi last updated on 01/Oct/19
I hope these formulas cover all possible primitive solutions.Non- primitive solutions are integral multiple of primitive solutions.
$${I}\:{hope}\:{these}\:{formulas}\:{cover}\:{all}\: \\ $$$${possible}\:{primitive}\:{solutions}.{Non}- \\ $$$${primitive}\:{solutions}\:{are}\:{integral}\: \\ $$$${multiple}\:{of}\:{primitive}\:{solutions}. \\ $$
Commented by Prithwish sen last updated on 01/Oct/19
Sir the primitive quadruples which you derived from the lebesgue′s identity. Are you sure that it covers all the possible primitive quadruples ?
$$\mathrm{Sir}\:\mathrm{the}\:\mathrm{primitive}\:\mathrm{quadruples}\:\mathrm{which}\:\mathrm{you}\: \\ $$$$\mathrm{derived}\:\mathrm{from}\:\mathrm{the}\:\mathrm{lebesgue}'\mathrm{s}\:\mathrm{identity}.\:\mathrm{Are}\: \\ $$$$\mathrm{you}\:\mathrm{sure}\:\mathrm{that}\:\mathrm{it}\:\mathrm{covers}\:\mathrm{all}\:\mathrm{the}\:\mathrm{possible}\: \\ $$$$\mathrm{primitive}\:\mathrm{quadruples}\:?\: \\ $$
Commented by Prithwish sen last updated on 01/Oct/19
I think these are the nessecary but not the sufficient condition.
$$\mathrm{I}\:\mathrm{think}\:\mathrm{these}\:\mathrm{are}\:\mathrm{the}\:\mathrm{nessecary}\:\mathrm{but}\:\mathrm{not}\:\mathrm{the} \\ $$$$\mathrm{sufficient}\:\mathrm{condition}. \\ $$
Commented by Rasheed.Sindhi last updated on 01/Oct/19
Commented by Rasheed.Sindhi last updated on 01/Oct/19
“Thus, all primitivePythagorean quadruplesu are characterized by Lebsgue′s identity...” Sir I meant by above that all possible primitive roots can be derived.May be I am wrong.
$$“{Thus},\:{all}\:{primitivePythagorean}\:{quadruplesu} \\ $$$${are}\:{characterized}\:{by}\:{Lebsgue}'{s}\:{identity}…'' \\ $$$${Sir}\:{I}\:{meant}\:{by}\:{above}\:{that}\:{all}\:{possible} \\ $$$${primitive}\:{roots}\:{can}\:{be}\:{derived}.{May}\:{be} \\ $$$${I}\:{am}\:{wrong}. \\ $$
Commented by Prithwish sen last updated on 01/Oct/19
Actually sir I have some doubt to clear. By the way you have done an outstanding work. Excellent thinking.
$$\mathrm{Actually}\:\mathrm{sir}\:\mathrm{I}\:\mathrm{have}\:\mathrm{some}\:\mathrm{doubt}\:\mathrm{to}\:\mathrm{clear}.\:\mathrm{By}\: \\ $$$$\mathrm{the}\:\mathrm{way}\:\mathrm{you}\:\mathrm{have}\:\mathrm{done}\:\mathrm{an}\:\mathrm{outstanding}\:\mathrm{work}. \\ $$$$\mathrm{Excellent}\:\mathrm{thinking}. \\ $$
Commented by Rasheed.Sindhi last updated on 03/Oct/19
THANKS in advance sir! My email is below: rasheedsindhi@yahoo.com
$$\mathcal{THANKS}\:{in}\:{advance}\:{sir}!\:{My}\:{email} \\ $$$$\:{is}\:{below}: \\ $$$$\:\:\:\boldsymbol{{rasheedsindhi}}@\boldsymbol{{yahoo}}.\boldsymbol{{com}} \\ $$
Commented by Prithwish sen last updated on 03/Oct/19
Sir I have already sentyou the pdf. If you received that please give a feedback.
$$\mathrm{Sir}\:\mathrm{I}\:\mathrm{have}\:\mathrm{already}\:\mathrm{sentyou}\:\mathrm{the}\:\mathrm{pdf}.\:\mathrm{If}\:\mathrm{you}\: \\ $$$$\mathrm{received}\:\mathrm{that}\:\mathrm{please}\:\mathrm{give}\:\mathrm{a}\:\mathrm{feedback}. \\ $$
Commented by Prithwish sen last updated on 03/Oct/19
Sir actually I find something on net regarding pythagorean quadruples very interesting. I will post it later. Now I am little busy. So please wait.
$$\mathrm{Sir}\:\mathrm{actually}\:\mathrm{I}\:\mathrm{find}\:\mathrm{something}\:\mathrm{on}\:\mathrm{net}\:\mathrm{regarding} \\ $$$$\mathrm{pythagorean}\:\mathrm{quadruples}\:\mathrm{very}\:\mathrm{interesting}.\:\mathrm{I}\:\mathrm{will}\:\mathrm{post}\:\mathrm{it}\:\mathrm{later}.\: \\ $$$$\mathrm{Now}\:\mathrm{I}\:\mathrm{am}\:\mathrm{little}\:\mathrm{busy}.\:\mathrm{So}\:\mathrm{please}\:\mathrm{wait}. \\ $$
Commented by Prithwish sen last updated on 03/Oct/19
Commented by Prithwish sen last updated on 03/Oct/19
Commented by Prithwish sen last updated on 03/Oct/19
Commented by Rasheed.Sindhi last updated on 03/Oct/19
ThαnX Prithwish sen sir for this precious information! pl share web url also!
$$\mathcal{T}{h}\alpha{n}\mathcal{X}\:{Prithwish}\:{sen}\:{sir}\:{for}\:{this} \\ $$$${precious}\:{information}!\:{pl}\:{share}\:{web} \\ $$$${url}\:{also}! \\ $$
Commented by Prithwish sen last updated on 03/Oct/19
Sir I am not so good at using gadgets. If you send your mail then I can send you the entire pdf.
$$\mathrm{Sir}\:\mathrm{I}\:\mathrm{am}\:\mathrm{not}\:\mathrm{so}\:\mathrm{good}\:\mathrm{at}\:\mathrm{using}\:\mathrm{gadgets}.\:\mathrm{If}\:\mathrm{you} \\ $$$$\mathrm{send}\:\mathrm{your}\:\mathrm{mail}\:\mathrm{then}\:\mathrm{I}\:\mathrm{can}\:\mathrm{send}\:\mathrm{you}\:\mathrm{the}\:\mathrm{entire} \\ $$$$\mathrm{pdf}. \\ $$
Commented by Rasheed.Sindhi last updated on 03/Oct/19
Sir I recieved the pdf.I′ll study it. Thanks again.We should think how can we relate pythagoren quadruples to the question above.My attempt in this connection nearly failed... Pl sir study my first answer again. I think formulas there can be used to produce all possible solutions of xy=(x+y)z
$${Sir}\:{I}\:{recieved}\:{the}\:{pdf}.{I}'{ll}\:{study}\:{it}. \\ $$$${Thanks}\:{again}.{We}\:{should}\:{think}\:{how} \\ $$$${can}\:{we}\:{relate}\:{pythagoren}\:{quadruples} \\ $$$${to}\:{the}\:{question}\:{above}.{My}\:{attempt}\:{in} \\ $$$${this}\:{connection}\:{nearly}\:{failed}… \\ $$$$\:{Pl}\:{sir}\:{study}\:{my}\:{first}\:{answer}\:{again}. \\ $$$${I}\:{think}\:{formulas}\:{there}\:{can}\:{be}\:{used} \\ $$$${to}\:{produce}\:{all}\:{possible}\:{solutions}\:{of} \\ $$$$\boldsymbol{{xy}}=\left(\boldsymbol{{x}}+\boldsymbol{{y}}\right)\boldsymbol{{z}} \\ $$
Commented by Prithwish sen last updated on 03/Oct/19
Excellent work sir. If all the possible primitive solutions can be produced then the all non primitive solutions also can be produced. Great work Sir.
$$\mathrm{Excellent}\:\mathrm{work}\:\mathrm{sir}.\:\mathrm{If}\:\mathrm{all}\:\mathrm{the}\:\mathrm{possible}\: \\ $$$$\mathrm{primitive}\:\mathrm{solutions}\:\mathrm{can}\:\mathrm{be}\:\mathrm{produced}\:\mathrm{then} \\ $$$$\mathrm{the}\:\mathrm{all}\:\mathrm{non}\:\mathrm{primitive}\:\mathrm{solutions}\:\mathrm{also}\:\mathrm{can}\:\mathrm{be} \\ $$$$\mathrm{produced}.\:\mathrm{Great}\:\mathrm{work}\:\mathrm{Sir}. \\ $$
Commented by Rasheed.Sindhi last updated on 03/Oct/19
Thanks for Encouraging sir!
$${Thanks}\:{for}\:\mathcal{E}{ncouraging}\:{sir}! \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *