Question Number 10143 by konen last updated on 26/Jan/17
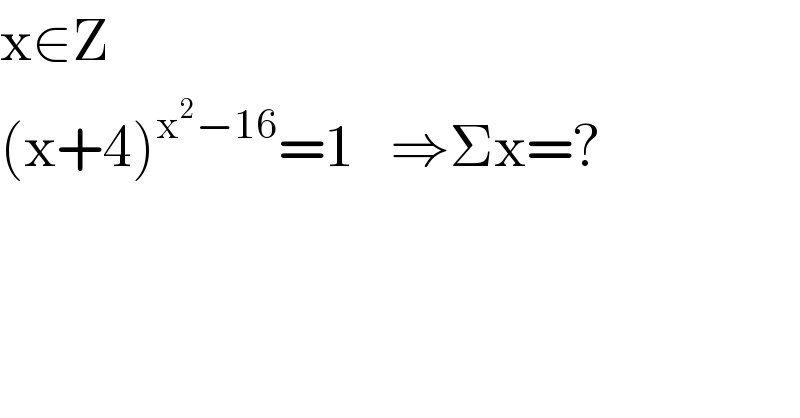
$$\mathrm{x}\in\mathrm{Z} \\ $$$$\left(\mathrm{x}+\mathrm{4}\right)^{\mathrm{x}^{\mathrm{2}} −\mathrm{16}} =\mathrm{1}\:\:\:\Rightarrow\Sigma\mathrm{x}=? \\ $$
Answered by mrW1 last updated on 27/Jan/17
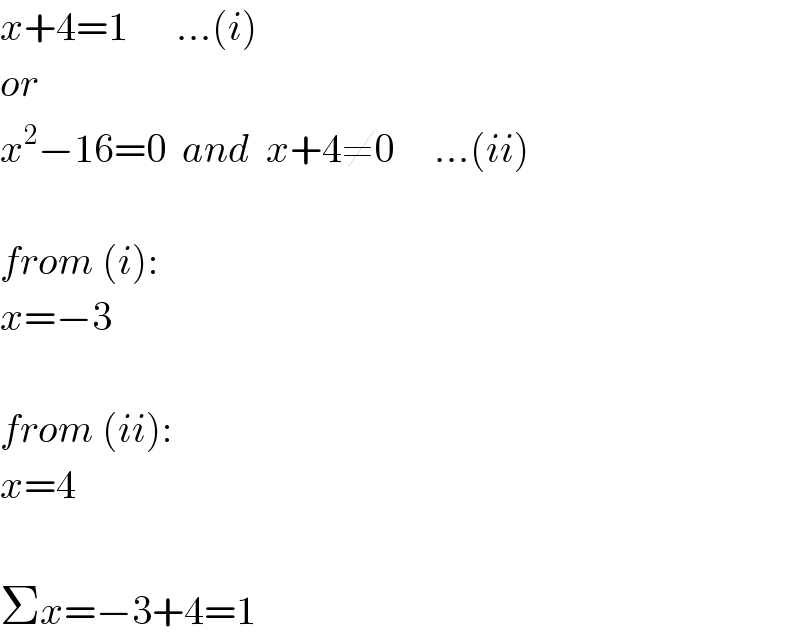
$${x}+\mathrm{4}=\mathrm{1}\:\:\:\:\:\:…\left({i}\right) \\ $$$${or} \\ $$$${x}^{\mathrm{2}} −\mathrm{16}=\mathrm{0}\:\:{and}\:\:{x}+\mathrm{4}\neq\mathrm{0}\:\:\:\:\:…\left({ii}\right) \\ $$$$ \\ $$$${from}\:\left({i}\right): \\ $$$${x}=−\mathrm{3} \\ $$$$ \\ $$$${from}\:\left({ii}\right): \\ $$$${x}=\mathrm{4} \\ $$$$ \\ $$$$\Sigma{x}=−\mathrm{3}+\mathrm{4}=\mathrm{1} \\ $$
Answered by sandy_suhendra last updated on 27/Jan/17
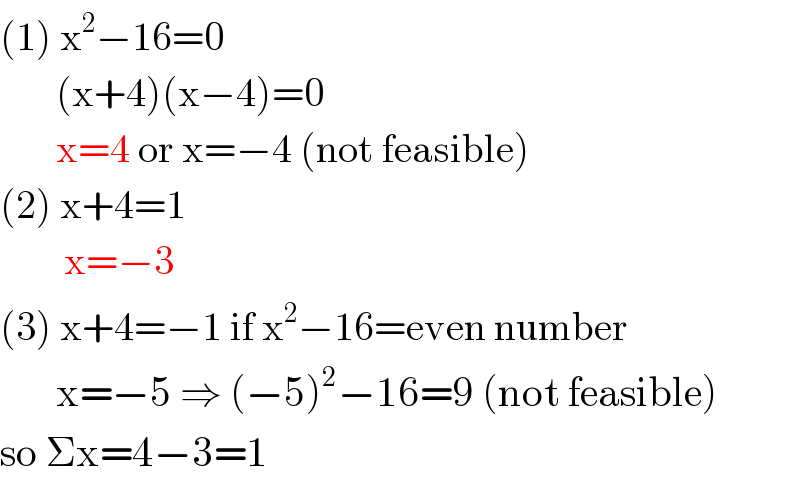
$$\left(\mathrm{1}\right)\:\mathrm{x}^{\mathrm{2}} −\mathrm{16}=\mathrm{0} \\ $$$$\:\:\:\:\:\:\:\left(\mathrm{x}+\mathrm{4}\right)\left(\mathrm{x}−\mathrm{4}\right)=\mathrm{0} \\ $$$$\:\:\:\:\:\:\:\mathrm{x}=\mathrm{4}\:\mathrm{or}\:\mathrm{x}=−\mathrm{4}\:\left(\mathrm{not}\:\mathrm{feasible}\right) \\ $$$$\left(\mathrm{2}\right)\:\mathrm{x}+\mathrm{4}=\mathrm{1} \\ $$$$\:\:\:\:\:\:\:\:\mathrm{x}=−\mathrm{3} \\ $$$$\left(\mathrm{3}\right)\:\mathrm{x}+\mathrm{4}=−\mathrm{1}\:\mathrm{if}\:\mathrm{x}^{\mathrm{2}} −\mathrm{16}=\mathrm{even}\:\mathrm{number} \\ $$$$\:\:\:\:\:\:\:\mathrm{x}=−\mathrm{5}\:\Rightarrow\:\left(−\mathrm{5}\right)^{\mathrm{2}} −\mathrm{16}=\mathrm{9}\:\left(\mathrm{not}\:\mathrm{feasible}\right) \\ $$$$\mathrm{so}\:\Sigma\mathrm{x}=\mathrm{4}−\mathrm{3}=\mathrm{1} \\ $$
