Question Number 77573 by MJS last updated on 08/Jan/20

$${x}\mathrm{ln}\:{x}\:=\frac{\mathrm{143851}}{\mathrm{40000}} \\ $$$$\mathrm{solve}\:\mathrm{for}\:{x}\:\Rightarrow\:\mathrm{nice}\:\mathrm{surprise} \\ $$
Commented by Tony Lin last updated on 08/Jan/20
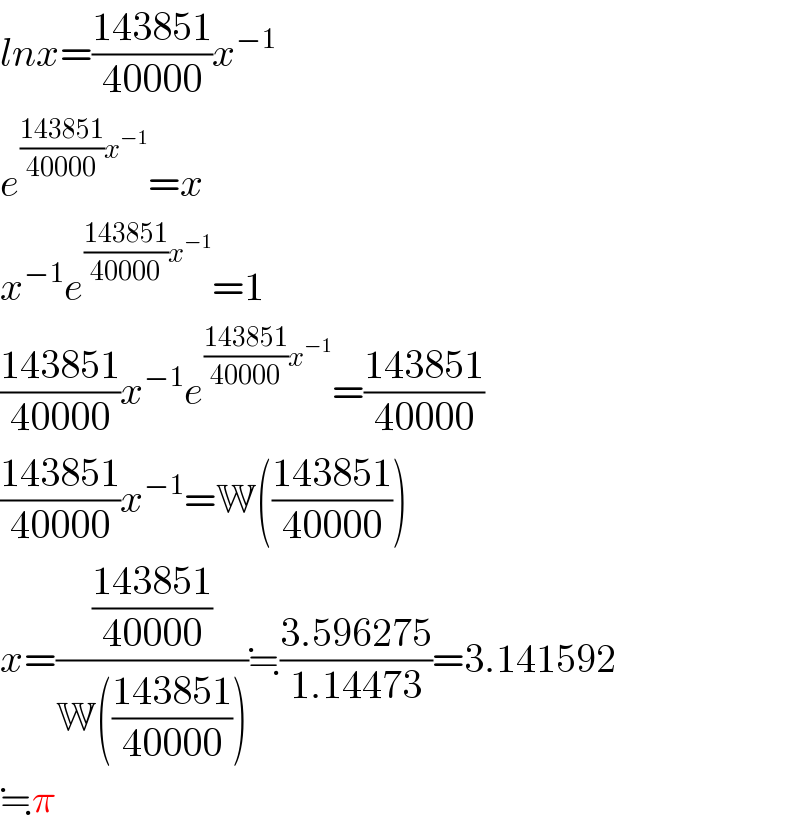
$${lnx}=\frac{\mathrm{143851}}{\mathrm{40000}}{x}^{−\mathrm{1}} \\ $$$${e}^{\frac{\mathrm{143851}}{\mathrm{40000}}{x}^{−\mathrm{1}} } ={x} \\ $$$${x}^{−\mathrm{1}} {e}^{\frac{\mathrm{143851}}{\mathrm{40000}}{x}^{−\mathrm{1}} } =\mathrm{1}\: \\ $$$$\frac{\mathrm{143851}}{\mathrm{40000}}{x}^{−\mathrm{1}} {e}^{\frac{\mathrm{143851}}{\mathrm{40000}}{x}^{−\mathrm{1}} } =\frac{\mathrm{143851}}{\mathrm{40000}} \\ $$$$\frac{\mathrm{143851}}{\mathrm{40000}}{x}^{−\mathrm{1}} =\mathbb{W}\left(\frac{\mathrm{143851}}{\mathrm{40000}}\right) \\ $$$${x}=\frac{\frac{\mathrm{143851}}{\mathrm{40000}}}{\mathbb{W}\left(\frac{\mathrm{143851}}{\mathrm{40000}}\right)}\fallingdotseq\frac{\mathrm{3}.\mathrm{596275}}{\mathrm{1}.\mathrm{14473}}=\mathrm{3}.\mathrm{141592} \\ $$$$\fallingdotseq\pi \\ $$
Commented by MJS last updated on 08/Jan/20

$$\mathrm{yes}.\:\mathrm{somebody}\:\mathrm{tried}\:\mathrm{to}\:\mathrm{prove}\:\mathrm{to}\:\mathrm{me}\:\mathrm{that}\:\pi \\ $$$$\mathrm{was}\:\mathrm{somehow}\:\mathrm{rational}\:\mathrm{with}\:\mathrm{these}\:“\mathrm{equations}'' \\ $$$${p}=\pi\mathrm{ln}\:\pi\:=\frac{\mathrm{143851}}{\mathrm{40000}} \\ $$$${q}=\pi\mathrm{ln}\:\left(\pi\mathrm{ln}\:\pi\right)\:=\frac{\mathrm{100523}}{\mathrm{25000}} \\ $$$$\mathrm{but}\:\mathrm{he}\:\mathrm{used}\:\mathrm{a}\:\mathrm{calculator}\:\mathrm{with}\:\mathrm{only}\:\mathrm{10}\:\mathrm{digits} \\ $$$${p}=\mathrm{3}.\mathrm{5962749997}…\approx\mathrm{3}.\mathrm{596275} \\ $$$${q}=\mathrm{4}.\mathrm{0209199997}…\approx\mathrm{4}.\mathrm{02092} \\ $$$$\mathrm{near}\:\mathrm{enough}\:\mathrm{is}\:\mathrm{not}\:\mathrm{good}\:\mathrm{enough},\:\mathrm{snyway}\:\mathrm{I} \\ $$$$\mathrm{I}\:\mathrm{found}\:\mathrm{it}\:\mathrm{interesting} \\ $$
