Question Number 758 by 13/NaSaNa(N)056565 last updated on 08/Mar/15
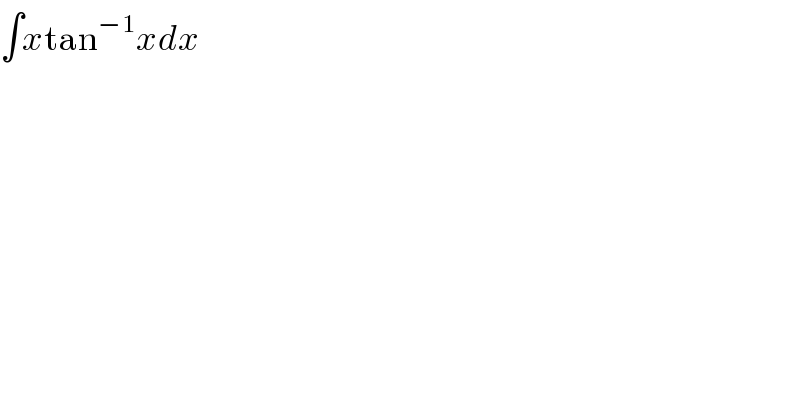
$$\int{x}\mathrm{tan}^{−\mathrm{1}} {xdx} \\ $$$$ \\ $$
Commented by malwaan last updated on 08/Mar/15
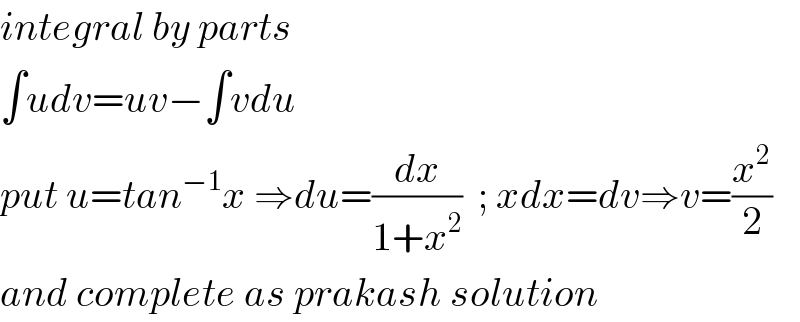
$${integral}\:{by}\:{parts} \\ $$$$\int{udv}={uv}−\int{vdu}\: \\ $$$${put}\:{u}={tan}^{−\mathrm{1}} {x}\:\Rightarrow{du}=\frac{{dx}}{\mathrm{1}+{x}^{\mathrm{2}} }\:\:;\:{xdx}={dv}\Rightarrow{v}=\frac{{x}^{\mathrm{2}} }{\mathrm{2}} \\ $$$${and}\:{complete}\:{as}\:{prakash}\:{solution} \\ $$
Answered by prakash jain last updated on 08/Mar/15
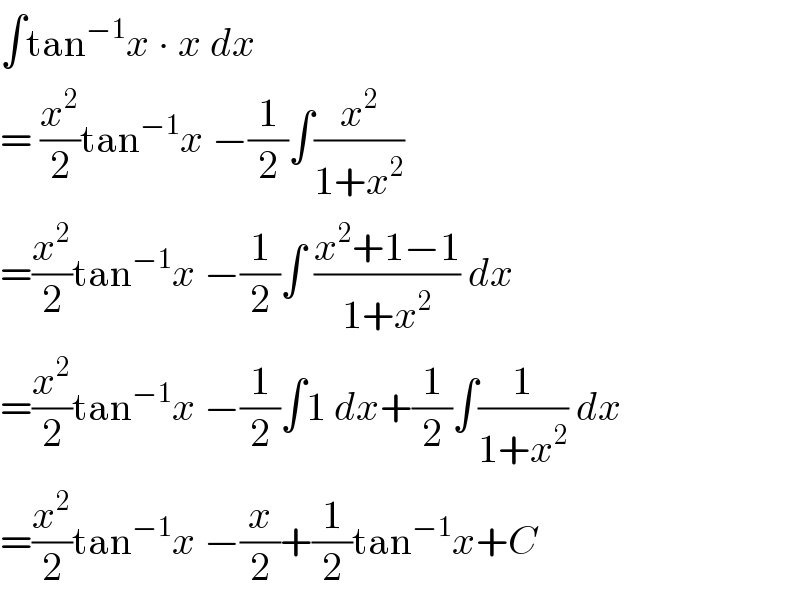
$$\int\mathrm{tan}^{−\mathrm{1}} {x}\:\centerdot\:{x}\:{dx}\:\: \\ $$$$=\:\frac{{x}^{\mathrm{2}} }{\mathrm{2}}\mathrm{tan}^{−\mathrm{1}} {x}\:−\frac{\mathrm{1}}{\mathrm{2}}\int\frac{{x}^{\mathrm{2}} }{\mathrm{1}+{x}^{\mathrm{2}} } \\ $$$$=\frac{{x}^{\mathrm{2}} }{\mathrm{2}}\mathrm{tan}^{−\mathrm{1}} {x}\:−\frac{\mathrm{1}}{\mathrm{2}}\int\:\frac{{x}^{\mathrm{2}} +\mathrm{1}−\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{2}} }\:{dx} \\ $$$$=\frac{{x}^{\mathrm{2}} }{\mathrm{2}}\mathrm{tan}^{−\mathrm{1}} {x}\:−\frac{\mathrm{1}}{\mathrm{2}}\int\mathrm{1}\:{dx}+\frac{\mathrm{1}}{\mathrm{2}}\int\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{2}} }\:{dx} \\ $$$$=\frac{{x}^{\mathrm{2}} }{\mathrm{2}}\mathrm{tan}^{−\mathrm{1}} {x}\:−\frac{{x}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}}\mathrm{tan}^{−\mathrm{1}} {x}+{C} \\ $$
