Question Number 6609 by Temp last updated on 05/Jul/16

Commented by prakash jain last updated on 05/Jul/16

Commented by prakash jain last updated on 05/Jul/16

Commented by prakash jain last updated on 05/Jul/16
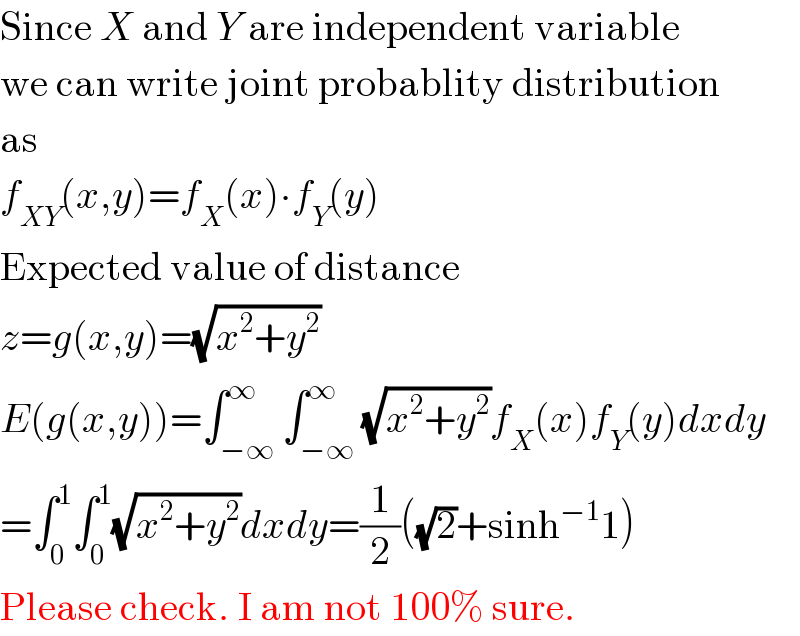
Commented by Temp last updated on 05/Jul/16

Commented by prakash jain last updated on 07/Jul/16

