Question Number 89986 by M±th+et£s last updated on 20/Apr/20
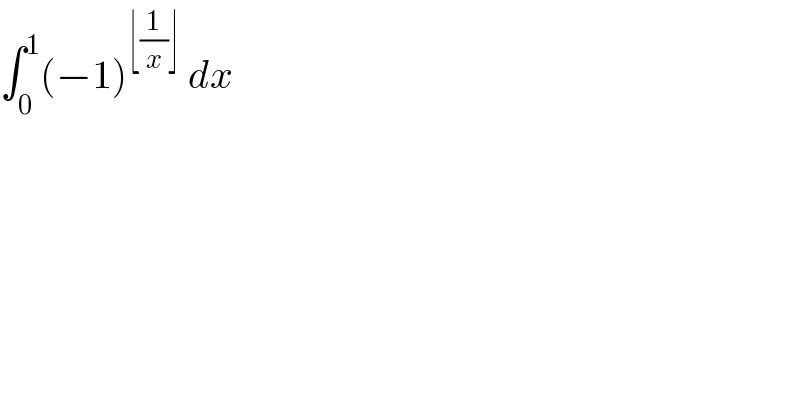
$$\int_{\mathrm{0}} ^{\mathrm{1}} \left(−\mathrm{1}\right)^{\lfloor\frac{\mathrm{1}}{{x}}\rfloor} \:{dx} \\ $$
Commented by M±th+et£s last updated on 20/Apr/20
$${nice}\:{and}\:{correct}\:{solution}\:{thank}\:{you} \\ $$$${sir} \\ $$
Commented by mathmax by abdo last updated on 20/Apr/20
![changement (1/x)=t give ∫_0 ^1 (−1)^([(1/x)]) dx =−∫_1 ^(+∞) (−1)^([t]) (−(dt/t^2 )) =∫_1 ^(+∞) (((−1)^([t]) )/t^2 )dt =Σ_(n=1) ^∞ ∫_n ^(n+1) (((−1)^n )/t^2 )dt =Σ_(n=1) ^∞ (−1)^n [−(1/t)]_n ^(n+1) =Σ_(n=1) ^∞ (−1)^n {(1/n)−(1/(n+1))} =Σ_(n=1) ^∞ (((−1)^n )/n)−Σ_(n=1) ^∞ (((−1)^n )/(n+1)) we have Σ_(n=1) ^∞ (((−1)^n )/n)=−ln(2) Σ_(n=1) ^∞ (((−1)^n )/(n+1)) =Σ_(n=2) ^∞ (((−1)^(n−1) )/n) =−Σ_(n=2) ^∞ (((−1)^n )/n) =−(Σ_(n=1) ^∞ (((−1)^n )/n)+1) =ln(2)−1 ⇒ ∫_0 ^1 (−1)^([(1/x)]) dx =−ln(2)−ln(2)+1 =1−2ln(2)](https://www.tinkutara.com/question/Q89993.png)
$${changement}\:\frac{\mathrm{1}}{{x}}={t}\:{give} \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \left(−\mathrm{1}\right)^{\left[\frac{\mathrm{1}}{{x}}\right]} \:{dx}\:=−\int_{\mathrm{1}} ^{+\infty} \:\left(−\mathrm{1}\right)^{\left[{t}\right]} \left(−\frac{{dt}}{{t}^{\mathrm{2}} }\right) \\ $$$$=\int_{\mathrm{1}} ^{+\infty} \:\frac{\left(−\mathrm{1}\right)^{\left[{t}\right]} }{{t}^{\mathrm{2}} }{dt}\:=\sum_{{n}=\mathrm{1}} ^{\infty} \:\int_{{n}} ^{{n}+\mathrm{1}} \:\frac{\left(−\mathrm{1}\right)^{{n}} }{{t}^{\mathrm{2}} }{dt} \\ $$$$=\sum_{{n}=\mathrm{1}} ^{\infty} \left(−\mathrm{1}\right)^{{n}} \left[−\frac{\mathrm{1}}{{t}}\right]_{{n}} ^{{n}+\mathrm{1}} \:=\sum_{{n}=\mathrm{1}} ^{\infty} \left(−\mathrm{1}\right)^{{n}} \left\{\frac{\mathrm{1}}{{n}}−\frac{\mathrm{1}}{{n}+\mathrm{1}}\right\} \\ $$$$=\sum_{{n}=\mathrm{1}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{{n}} }{{n}}−\sum_{{n}=\mathrm{1}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{{n}} }{{n}+\mathrm{1}}\:\:{we}\:{have} \\ $$$$\sum_{{n}=\mathrm{1}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{{n}} }{{n}}=−{ln}\left(\mathrm{2}\right) \\ $$$$\sum_{{n}=\mathrm{1}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{{n}} }{{n}+\mathrm{1}}\:=\sum_{{n}=\mathrm{2}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{{n}−\mathrm{1}} }{{n}}\:=−\sum_{{n}=\mathrm{2}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{{n}} }{{n}} \\ $$$$=−\left(\sum_{{n}=\mathrm{1}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{{n}} }{{n}}+\mathrm{1}\right)\:={ln}\left(\mathrm{2}\right)−\mathrm{1}\:\Rightarrow \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \:\left(−\mathrm{1}\right)^{\left[\frac{\mathrm{1}}{{x}}\right]} {dx}\:=−{ln}\left(\mathrm{2}\right)−{ln}\left(\mathrm{2}\right)+\mathrm{1}\:=\mathrm{1}−\mathrm{2}{ln}\left(\mathrm{2}\right) \\ $$
Commented by mathmax by abdo last updated on 20/Apr/20
$${you}\:{are}\:{welcome}. \\ $$
