0-1-2-0-pi-2-1-ycos-x-1-dxdy-

Question Number 86613 by Ar Brandon last updated on 29/Mar/20

\int_{0}^{\frac{1}{2}} \int_{0}^{\frac{π}{2}} \frac{1}{y c o s (x) + 1} d x d y

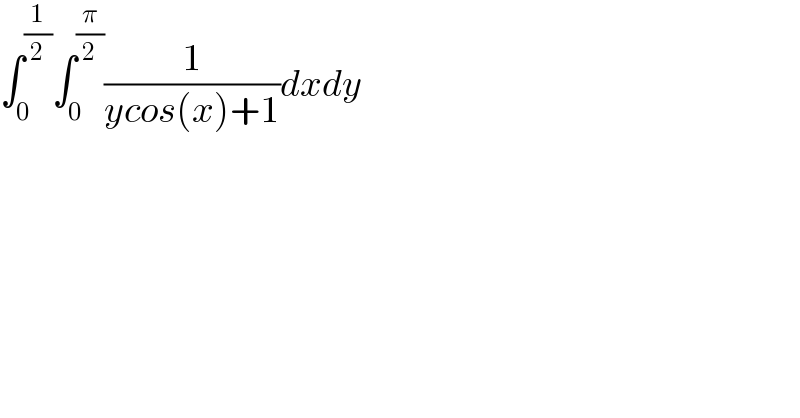
Commented by abdomathmax last updated on 29/Mar/20

\int_{0}^{\frac{π}{2}} \frac{d x}{y c o s x + 1} =_{t a n (\frac{x}{2}) = t} \int_{0}^{1} \frac{2 d t}{(1 + t^{2}) (y \frac{1 - t^{2}}{1 + t^{2}} + 1)}

= 2 \int_{0}^{1} \frac{d t}{y - {y t}^{2} + 1 + t^{2}} = 2 \int_{0}^{1} \frac{d y}{(1 - y) t^{2} + 1 + y}

= \frac{2}{(1 - y)} \int_{0}^{1} \frac{d y}{t^{2} + \frac{1 + y}{1 - y}}

=_{t = \sqrt{\frac{1 + y}{1 - y}} u} \frac{2}{(1 - y)} \times \frac{1 - y}{1 + y} \int_{0}^{\sqrt{\frac{1 - y}{1 + y}}} \frac{1}{1 + u^{2}} \times \sqrt{\frac{1 + y}{1 - y}} d u

= \frac{2}{\sqrt{1 - y^{2}}} a r c t a n (\sqrt{\frac{1 - y}{1 + y}}) \Rightarrow

\int_{0}^{\frac{1}{2}} \int_{0}^{\frac{π}{2}} \frac{d x d y}{y c o s x + 1} = 2 \int_{0}^{\frac{1}{2}} \frac{1}{\sqrt{1 - y^{2}}} a r c t a n (\sqrt{\frac{1 - y}{1 + y}}) d y

\dots b e c o n t i n u e d \dots

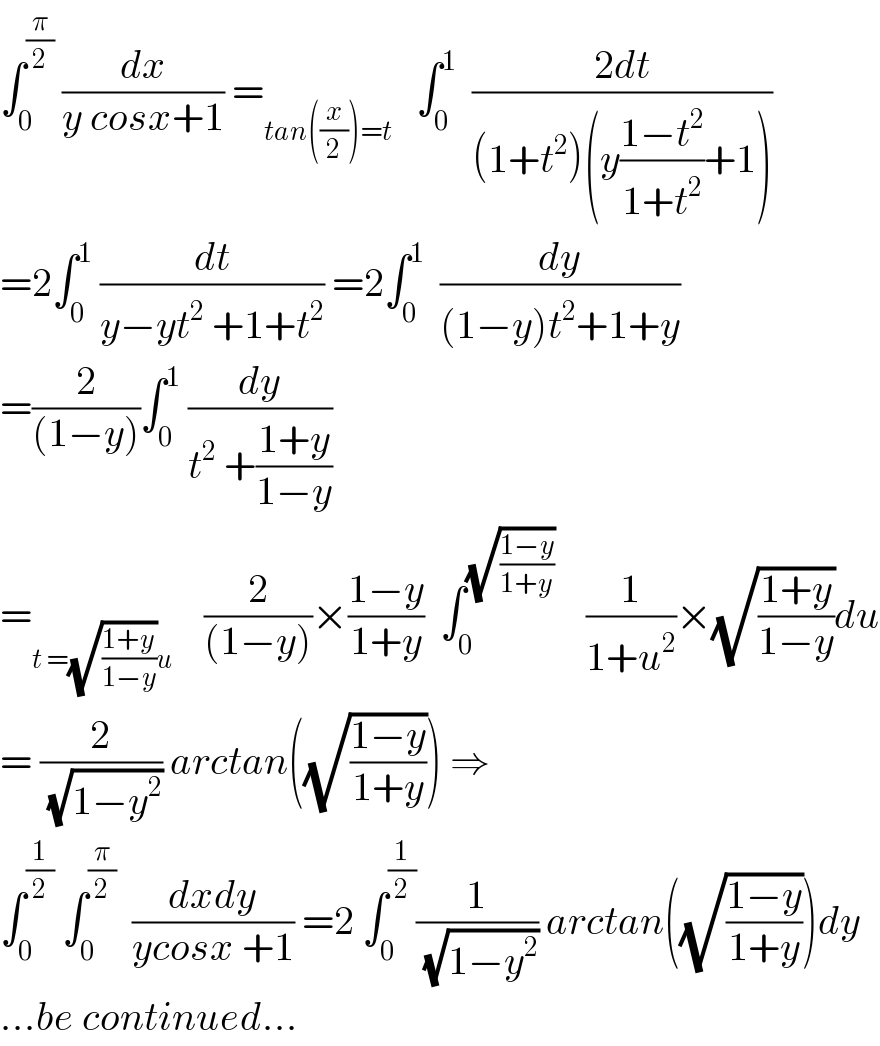
Commented by mind is power last updated on 30/Mar/20

y = c o s (2 θ)

a a r c t a n (\sqrt{\frac{1 - y}{1 + y}}) = a r c t a n (t a n (θ)) = θ

Commented by mathmax by abdo last updated on 30/Mar/20

c h a n g e m e n t y = c o s θ g i v e

\int_{0}^{\frac{1}{2}} \frac{1}{\sqrt{1 - y^{2}}} a r c t a n (\sqrt{\frac{1 - y}{1 + y}}) d y = \int_{\frac{π}{2}}^{\frac{π}{3}} \frac{1}{s i n θ} a r c t a n (\sqrt{\frac{2 {s i n}^{2} (\frac{θ}{2})}{2 {c o s}^{2} (\frac{θ}{2})}}) (- s i n θ) d θ

= \int_{\frac{π}{3}}^{\frac{π}{2}} a r c t a n (t a n (\frac{θ}{2})) d θ = \frac{1}{2} \int_{\frac{π}{3}}^{\frac{π}{2}} θ d θ = \frac{1}{2} {[\frac{θ^{2}}{2}]}_{\frac{π}{3}}^{\frac{π}{2}}

= \frac{1}{4} (\frac{π^{2}}{4} - \frac{π^{2}}{9}) = \frac{1}{4} (\frac{9 π^{2} - 4 π^{2}}{36}) = \frac{5 π^{2}}{144}

Commented by mathmax by abdo last updated on 30/Mar/20

t h a n k y o u s i r m i n d . .

Commented by Ar Brandon last updated on 30/Mar/20

g r e a t j o b

Commented by abdomathmax last updated on 30/Mar/20

y o u a r e w e l c o m e s i r .