Question Number 122751 by bemath last updated on 19/Nov/20
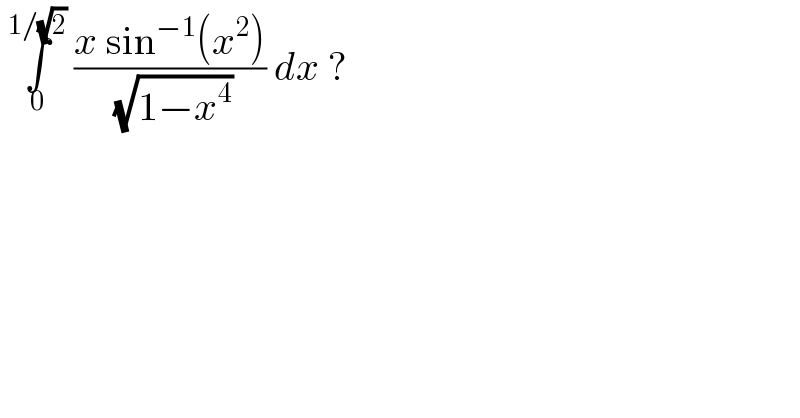
$$\:\underset{\mathrm{0}} {\overset{\mathrm{1}/\sqrt{\mathrm{2}}} {\int}}\:\frac{{x}\:\mathrm{sin}^{−\mathrm{1}} \left({x}^{\mathrm{2}} \right)}{\:\sqrt{\mathrm{1}−{x}^{\mathrm{4}} }}\:{dx}\:?\: \\ $$
Answered by liberty last updated on 19/Nov/20
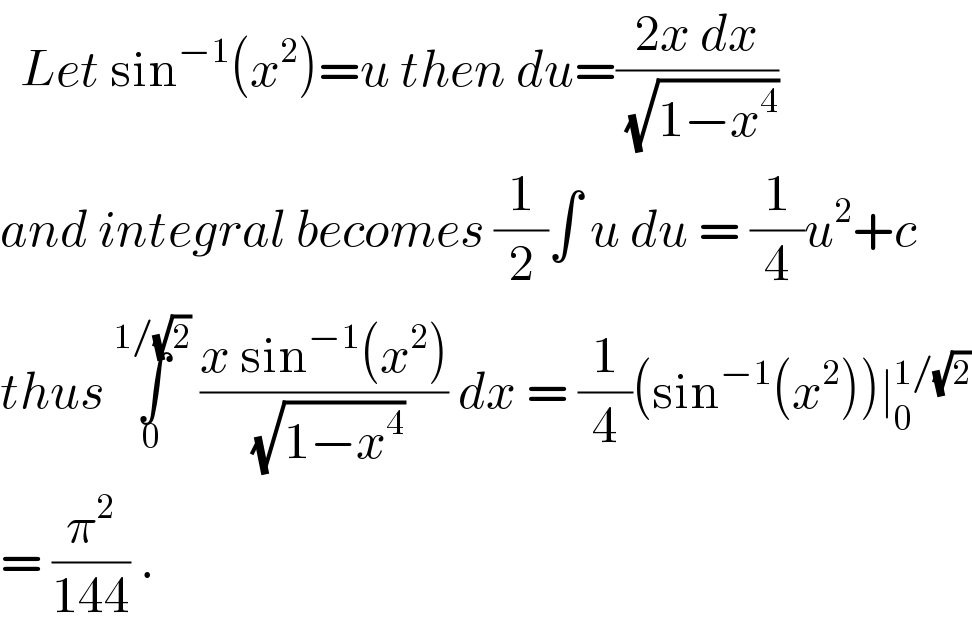
$$\:\:{Let}\:\mathrm{sin}^{−\mathrm{1}} \left({x}^{\mathrm{2}} \right)={u}\:{then}\:{du}=\frac{\mathrm{2}{x}\:{dx}}{\:\sqrt{\mathrm{1}−{x}^{\mathrm{4}} }} \\ $$$${and}\:{integral}\:{becomes}\:\frac{\mathrm{1}}{\mathrm{2}}\int\:{u}\:{du}\:=\:\frac{\mathrm{1}}{\mathrm{4}}{u}^{\mathrm{2}} +{c} \\ $$$${thus}\:\underset{\mathrm{0}} {\overset{\mathrm{1}/\sqrt{\mathrm{2}}} {\int}}\:\frac{{x}\:\mathrm{sin}^{−\mathrm{1}} \left({x}^{\mathrm{2}} \right)}{\:\sqrt{\mathrm{1}−{x}^{\mathrm{4}} }}\:{dx}\:=\:\frac{\mathrm{1}}{\mathrm{4}}\left(\mathrm{sin}^{−\mathrm{1}} \left({x}^{\mathrm{2}} \right)\right)\mid_{\mathrm{0}} ^{\mathrm{1}/\sqrt{\mathrm{2}}} \\ $$$$=\:\frac{\pi^{\mathrm{2}} }{\mathrm{144}}\:.\: \\ $$
Answered by Dwaipayan Shikari last updated on 19/Nov/20
![∫_0 ^(1/( (√2))) ((xsin^(−1) (x^2 ))/( (√(1−x^4 ))))dx x^2 =t⇒2x=(dt/dx) (1/2)∫_0 ^(1/2) ((sin^(−1) (t))/( (√(1−t^2 ))))dt=(1/4)[(sin^(−1) t)^2 ]_0 ^(1/2) =(1/4).(π^2 /(36))=(π^2 /(144))](https://www.tinkutara.com/question/Q122755.png)
$$\int_{\mathrm{0}} ^{\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}} \frac{{xsin}^{−\mathrm{1}} \left({x}^{\mathrm{2}} \right)}{\:\sqrt{\mathrm{1}−{x}^{\mathrm{4}} }}{dx}\:\:\:\:\:\:{x}^{\mathrm{2}} ={t}\Rightarrow\mathrm{2}{x}=\frac{{dt}}{{dx}} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\frac{\mathrm{1}}{\mathrm{2}}} \frac{{sin}^{−\mathrm{1}} \left({t}\right)}{\:\sqrt{\mathrm{1}−{t}^{\mathrm{2}} }}{dt}=\frac{\mathrm{1}}{\mathrm{4}}\left[\left({sin}^{−\mathrm{1}} {t}\right)^{\mathrm{2}} \right]_{\mathrm{0}} ^{\frac{\mathrm{1}}{\mathrm{2}}} =\frac{\mathrm{1}}{\mathrm{4}}.\frac{\pi^{\mathrm{2}} }{\mathrm{36}}=\frac{\pi^{\mathrm{2}} }{\mathrm{144}} \\ $$
Answered by Bird last updated on 19/Nov/20
![I =∫_0 ^(1/( (√2))) ((xarcsin(x^2 ))/( (√(1−x^4 ))))dx we do the changement x^2 =t ⇒2xdx =dt ⇒ I =∫_0 ^(1/2) ((arcsin(t))/( (√(1−t^2 ))))(dt/2) ⇒2I =[arcsin^2 t]_0 ^(1/2) −∫_0 ^(1/2) ((arcsint)/( (√(1−t^2 ))))dt 3I =((π/6))^2 =(π^2 /(36)) ⇒I =(π^2 /(3.36)) =(π^2 /(108))](https://www.tinkutara.com/question/Q122810.png)
$${I}\:=\int_{\mathrm{0}} ^{\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}} \:\:\:\frac{{xarcsin}\left({x}^{\mathrm{2}} \right)}{\:\sqrt{\mathrm{1}−{x}^{\mathrm{4}} }}{dx}\:{we}\:{do}\:{the} \\ $$$${changement}\:{x}^{\mathrm{2}} ={t}\:\Rightarrow\mathrm{2}{xdx}\:={dt}\:\Rightarrow \\ $$$${I}\:=\int_{\mathrm{0}} ^{\frac{\mathrm{1}}{\mathrm{2}}} \:\frac{{arcsin}\left({t}\right)}{\:\sqrt{\mathrm{1}−{t}^{\mathrm{2}} }}\frac{{dt}}{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{2}{I}\:=\left[{arcsin}^{\mathrm{2}} {t}\right]_{\mathrm{0}} ^{\frac{\mathrm{1}}{\mathrm{2}}} −\int_{\mathrm{0}} ^{\frac{\mathrm{1}}{\mathrm{2}}} \:\frac{{arcsint}}{\:\sqrt{\mathrm{1}−{t}^{\mathrm{2}} }}{dt} \\ $$$$\mathrm{3}{I}\:=\left(\frac{\pi}{\mathrm{6}}\right)^{\mathrm{2}} \:=\frac{\pi^{\mathrm{2}} }{\mathrm{36}}\:\Rightarrow{I}\:=\frac{\pi^{\mathrm{2}} }{\mathrm{3}.\mathrm{36}} \\ $$$$=\frac{\pi^{\mathrm{2}} }{\mathrm{108}} \\ $$
Commented by liberty last updated on 19/Nov/20

$${no}.\:{the}\:{answer}\:\frac{\pi^{\mathrm{2}} }{\mathrm{144}} \\ $$
