Question Number 80175 by Rio Michael last updated on 31/Jan/20
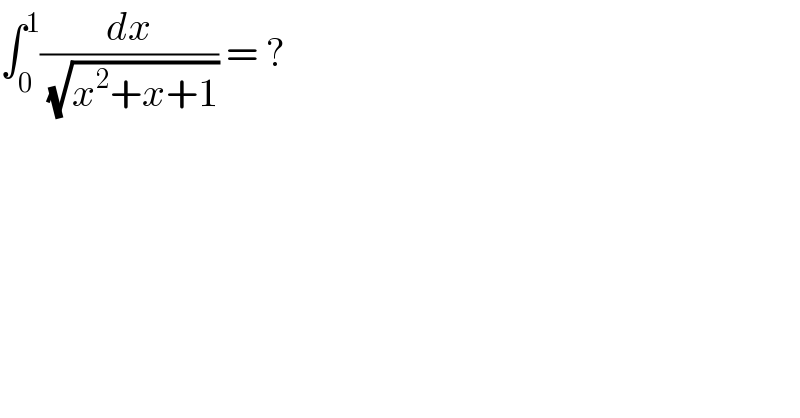
$$\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{dx}}{\:\sqrt{{x}^{\mathrm{2}} +{x}+\mathrm{1}}}\:=\:? \\ $$
Commented by mathmax by abdo last updated on 31/Jan/20
![let I=∫_0 ^1 (dx/( (√(x^2 +x+1)))) ⇒I=∫_0 ^1 (dx/( (√((x+(1/2))^2 +(3/4))))) cha7gement x+(1/2) =((√3)/2)tanθ give θ =arctan(((2x+1)/( (√3)))) I=∫_(π/6) ^(π/3) (1/( (√(3/4))(√(1+tan^2 θ))))×((√3)/2) (1+tan^2 θ)dθ =∫_(π/6) ^(π/3) (√(1+tan^2 θ))dθ =∫_(π/6) ^(π/3) (dθ/(cosθ)) =_(tan((θ/2))=u) ∫_(2−(√3)) ^(1/( (√3))) (1/((1−u^2 )/(1+u^2 )))×((2du)/(1+u^2 )) =∫_(2−(√3)) ^(1/( (√3))) ((1/(1−u))+(1/(1+u)))du =[ln∣((1+u)/(1−u))∣]_(2−(√3)) ^(1/( (√3))) =ln∣((1+(1/( (√3))))/(1−(1/( (√3)))))∣−ln∣((3−(√3))/(−1+(√3)))∣=ln∣(√3)+1∣−ln∣(√3)−1∣−ln∣(((√3)((√3)−1))/( (√3)−1))∣ =ln((√3)+1)−ln((√3)−1)−ln((√3))](https://www.tinkutara.com/question/Q80194.png)
$${let}\:{I}=\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{dx}}{\:\sqrt{{x}^{\mathrm{2}} +{x}+\mathrm{1}}}\:\Rightarrow{I}=\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{dx}}{\:\sqrt{\left({x}+\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} +\frac{\mathrm{3}}{\mathrm{4}}}} \\ $$$${cha}\mathrm{7}{gement}\:\:{x}+\frac{\mathrm{1}}{\mathrm{2}}\:=\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}{tan}\theta\:{give}\:\:\theta\:={arctan}\left(\frac{\mathrm{2}{x}+\mathrm{1}}{\:\sqrt{\mathrm{3}}}\right) \\ $$$${I}=\int_{\frac{\pi}{\mathrm{6}}} ^{\frac{\pi}{\mathrm{3}}} \:\:\frac{\mathrm{1}}{\:\sqrt{\frac{\mathrm{3}}{\mathrm{4}}}\sqrt{\mathrm{1}+{tan}^{\mathrm{2}} \theta}}×\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\:\left(\mathrm{1}+{tan}^{\mathrm{2}} \theta\right){d}\theta\:=\int_{\frac{\pi}{\mathrm{6}}} ^{\frac{\pi}{\mathrm{3}}} \sqrt{\mathrm{1}+{tan}^{\mathrm{2}} \theta}{d}\theta \\ $$$$=\int_{\frac{\pi}{\mathrm{6}}} ^{\frac{\pi}{\mathrm{3}}} \:\frac{{d}\theta}{{cos}\theta}\:=_{{tan}\left(\frac{\theta}{\mathrm{2}}\right)={u}} \:\:\:\:\int_{\mathrm{2}−\sqrt{\mathrm{3}}} ^{\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}} \:\:\:\:\frac{\mathrm{1}}{\frac{\mathrm{1}−{u}^{\mathrm{2}} }{\mathrm{1}+{u}^{\mathrm{2}} }}×\frac{\mathrm{2}{du}}{\mathrm{1}+{u}^{\mathrm{2}} } \\ $$$$=\int_{\mathrm{2}−\sqrt{\mathrm{3}}} ^{\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}} \left(\frac{\mathrm{1}}{\mathrm{1}−{u}}+\frac{\mathrm{1}}{\mathrm{1}+{u}}\right){du}\:=\left[{ln}\mid\frac{\mathrm{1}+{u}}{\mathrm{1}−{u}}\mid\right]_{\mathrm{2}−\sqrt{\mathrm{3}}} ^{\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}} \\ $$$$={ln}\mid\frac{\mathrm{1}+\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}}{\mathrm{1}−\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}}\mid−{ln}\mid\frac{\mathrm{3}−\sqrt{\mathrm{3}}}{−\mathrm{1}+\sqrt{\mathrm{3}}}\mid={ln}\mid\sqrt{\mathrm{3}}+\mathrm{1}\mid−{ln}\mid\sqrt{\mathrm{3}}−\mathrm{1}\mid−{ln}\mid\frac{\sqrt{\mathrm{3}}\left(\sqrt{\mathrm{3}}−\mathrm{1}\right)}{\:\sqrt{\mathrm{3}}−\mathrm{1}}\mid \\ $$$$={ln}\left(\sqrt{\mathrm{3}}+\mathrm{1}\right)−{ln}\left(\sqrt{\mathrm{3}}−\mathrm{1}\right)−{ln}\left(\sqrt{\mathrm{3}}\right) \\ $$
Answered by ~blr237~ last updated on 31/Jan/20
![Let named it A using 1+x+x^2 =(x+(1/2))^2 +(3/4) = (3/4)[(((2x+1)/( (√3))))^2 +1] A=∫_0 ^1 (2/( (√3))) (1/( (√([(((2x+1)/( (√3))))^2 +1)))) dx =[argsh(((2x+1)/( (√3))))]_0 ^1 = argsh((√3) )−argsh((1/( (√3)))) using argshx=ln(x+(√(1+x^2 )) ) A=ln(2+(√3))−ln((√3) )](https://www.tinkutara.com/question/Q80177.png)
$${Let}\:{named}\:{it}\:{A} \\ $$$${using}\:\:\mathrm{1}+{x}+{x}^{\mathrm{2}} =\left({x}+\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} +\frac{\mathrm{3}}{\mathrm{4}}\:=\:\frac{\mathrm{3}}{\mathrm{4}}\left[\left(\frac{\mathrm{2}{x}+\mathrm{1}}{\:\sqrt{\mathrm{3}}}\right)^{\mathrm{2}} +\mathrm{1}\right] \\ $$$$ \\ $$$${A}=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{\mathrm{2}}{\:\sqrt{\mathrm{3}}}\:\:\frac{\mathrm{1}}{\:\sqrt{\left[\left(\frac{\mathrm{2}{x}+\mathrm{1}}{\:\sqrt{\mathrm{3}}}\right)^{\mathrm{2}} +\mathrm{1}\right.}}\:{dx} \\ $$$$\:\:=\left[{argsh}\left(\frac{\mathrm{2}{x}+\mathrm{1}}{\:\sqrt{\mathrm{3}}}\right)\right]_{\mathrm{0}} ^{\mathrm{1}} =\:{argsh}\left(\sqrt{\mathrm{3}}\:\right)−{argsh}\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}\right) \\ $$$${using}\:\:{argshx}={ln}\left({x}+\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }\:\right)\: \\ $$$${A}={ln}\left(\mathrm{2}+\sqrt{\mathrm{3}}\right)−{ln}\left(\sqrt{\mathrm{3}}\:\right) \\ $$$$\: \\ $$
