Question Number 170578 by mnjuly1970 last updated on 27/May/22
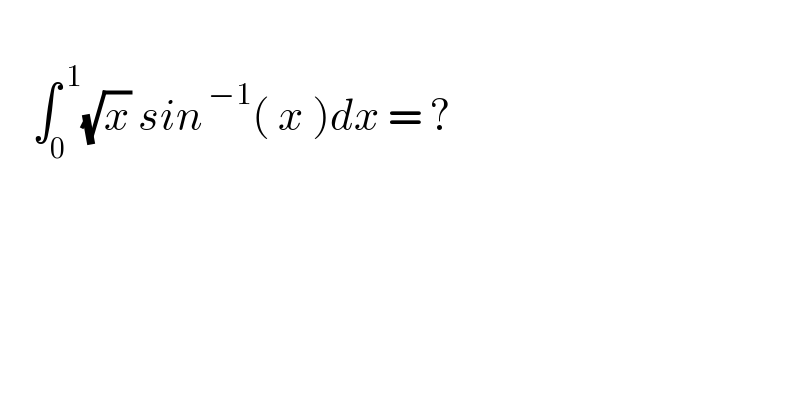
$$ \\ $$$$\:\:\:\:\int_{\mathrm{0}} ^{\:\mathrm{1}} \sqrt{{x}}\:{sin}^{\:−\mathrm{1}} \left(\:{x}\:\right){dx}\:=\:? \\ $$$$ \\ $$
Answered by Mathspace last updated on 27/May/22
![I=∫_0 ^1 (√x)arcsin(x)dx x=sint ⇒I=∫_0 ^(π/2) (√(sint))t cost dt =∫_0 ^(π/2) t(cost(√(sint)))dt let=u=t and v^′ =cost (√(sint)) ⇒v=(2/3)(sint)^(3/2) ⇒ I=[(2/3)t(sint)^(3/2) ]_0 ^(π/2) −(2/3)∫_0 ^(π/2) (sint)^(3/2) dt =(π/3)−(2/3)∫_0 ^(π/2) (sint)^(3/2) dt but we know 2∫_0 ^(π/2) cos^(2p−1) t sin^(2q−1) tdt =B(p,q)=((Γ(p).Γ(q))/(Γ(p+q))) ⇒ ∫_0 ^(π/2) (sint)^(3/2) dt=∫_0 ^(π/2) (sint)^(2.(5/4)−1) cos^(2((1/2))−1) dt =(1/2)B((5/4),(1/2))=(1/2)((Γ((5/4))Γ((1/2)))/(Γ((5/4)+(1/2)))) =((√π)/2)×((Γ((5/4)))/(Γ((7/4)))) ⇒ I=(π/3)−((√π)/3)×((Γ((5/4)))/(Γ((7/4))))](https://www.tinkutara.com/question/Q170598.png)
$${I}=\int_{\mathrm{0}} ^{\mathrm{1}} \sqrt{{x}}{arcsin}\left({x}\right){dx} \\ $$$${x}={sint}\:\Rightarrow{I}=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \sqrt{{sint}}{t}\:\:{cost}\:{dt} \\ $$$$=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {t}\left({cost}\sqrt{{sint}}\right){dt} \\ $$$${let}={u}={t}\:{and}\:{v}^{'} ={cost}\:\sqrt{{sint}} \\ $$$$\Rightarrow{v}=\frac{\mathrm{2}}{\mathrm{3}}\left({sint}\right)^{\frac{\mathrm{3}}{\mathrm{2}}} \:\Rightarrow \\ $$$${I}=\left[\frac{\mathrm{2}}{\mathrm{3}}{t}\left({sint}\right)^{\frac{\mathrm{3}}{\mathrm{2}}} \right]_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} −\frac{\mathrm{2}}{\mathrm{3}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \left({sint}\right)^{\frac{\mathrm{3}}{\mathrm{2}}} {dt} \\ $$$$=\frac{\pi}{\mathrm{3}}−\frac{\mathrm{2}}{\mathrm{3}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \left({sint}\right)^{\frac{\mathrm{3}}{\mathrm{2}}} {dt} \\ $$$${but}\:{we}\:{know}\:\mathrm{2}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {cos}^{\mathrm{2}{p}−\mathrm{1}} {t}\:{sin}^{\mathrm{2}{q}−\mathrm{1}} {tdt} \\ $$$$={B}\left({p},{q}\right)=\frac{\Gamma\left({p}\right).\Gamma\left({q}\right)}{\Gamma\left({p}+{q}\right)}\:\Rightarrow \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \left({sint}\right)^{\frac{\mathrm{3}}{\mathrm{2}}} {dt}=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \left({sint}\right)^{\mathrm{2}.\frac{\mathrm{5}}{\mathrm{4}}−\mathrm{1}} \:{cos}^{\mathrm{2}\left(\frac{\mathrm{1}}{\mathrm{2}}\right)−\mathrm{1}} {dt} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}{B}\left(\frac{\mathrm{5}}{\mathrm{4}},\frac{\mathrm{1}}{\mathrm{2}}\right)=\frac{\mathrm{1}}{\mathrm{2}}\frac{\Gamma\left(\frac{\mathrm{5}}{\mathrm{4}}\right)\Gamma\left(\frac{\mathrm{1}}{\mathrm{2}}\right)}{\Gamma\left(\frac{\mathrm{5}}{\mathrm{4}}+\frac{\mathrm{1}}{\mathrm{2}}\right)} \\ $$$$=\frac{\sqrt{\pi}}{\mathrm{2}}×\frac{\Gamma\left(\frac{\mathrm{5}}{\mathrm{4}}\right)}{\Gamma\left(\frac{\mathrm{7}}{\mathrm{4}}\right)}\:\Rightarrow \\ $$$${I}=\frac{\pi}{\mathrm{3}}−\frac{\sqrt{\pi}}{\mathrm{3}}×\frac{\Gamma\left(\frac{\mathrm{5}}{\mathrm{4}}\right)}{\Gamma\left(\frac{\mathrm{7}}{\mathrm{4}}\right)} \\ $$
Commented by mnjuly1970 last updated on 28/May/22
$${verynice}\:\:{solution}\:\:{thank}\:{you}.. \\ $$
Commented by Tawa11 last updated on 08/Oct/22

$$\mathrm{Great}\:\mathrm{sir} \\ $$
