Menu Close

0-1-x-x-dx-

Tinku Tara 0 Comments
Pin




Question Number 103343 by Dwaipayan Shikari last updated on 14/Jul/20
∫_0 ^1 x^(−x) dx
$$\int_{\mathrm{0}} ^{\mathrm{1}} {x}^{−{x}} {dx} \\ $$
Answered by mathmax by abdo last updated on 15/Jul/20
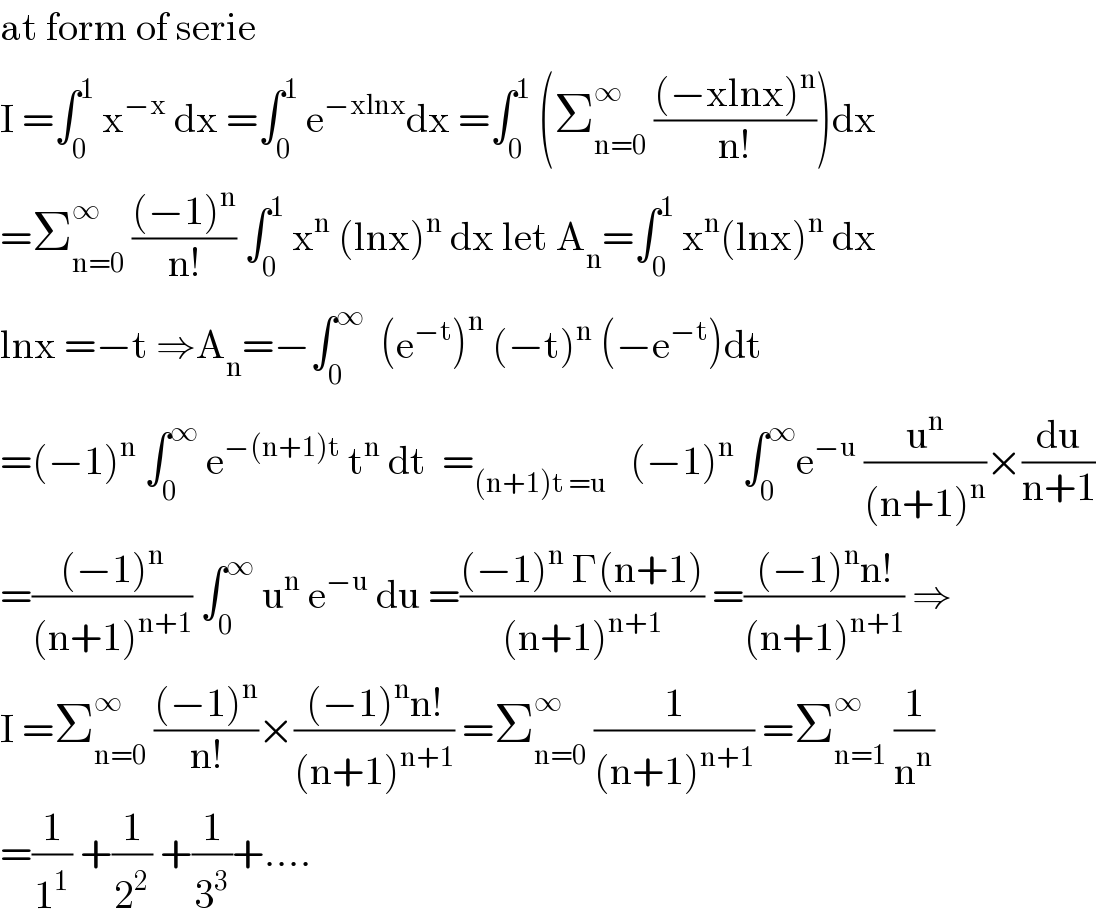
at form of serie I =∫_0 ^1 x^(−x) dx =∫_0 ^1 e^(−xlnx) dx =∫_0 ^1 (Σ_(n=0) ^∞ (((−xlnx)^n )/(n!)))dx =Σ_(n=0) ^∞ (((−1)^n )/(n!)) ∫_0 ^1 x^n (lnx)^n dx let A_n =∫_0 ^1 x^n (lnx)^n dx lnx =−t ⇒A_n =−∫_0 ^∞ (e^(−t) )^n (−t)^n (−e^(−t) )dt =(−1)^n ∫_0 ^∞ e^(−(n+1)t) t^n dt =_((n+1)t =u) (−1)^n ∫_0 ^∞ e^(−u) (u^n /((n+1)^n ))×(du/(n+1)) =(((−1)^n )/((n+1)^(n+1) )) ∫_0 ^∞ u^n e^(−u) du =(((−1)^n Γ(n+1))/((n+1)^(n+1) )) =(((−1)^n n!)/((n+1)^(n+1) )) ⇒ I =Σ_(n=0) ^∞ (((−1)^n )/(n!))×(((−1)^n n!)/((n+1)^(n+1) )) =Σ_(n=0) ^∞ (1/((n+1)^(n+1) )) =Σ_(n=1) ^∞ (1/n^n ) =(1/1^1 ) +(1/2^2 ) +(1/3^3 )+....
$$\mathrm{at}\:\mathrm{form}\:\mathrm{of}\:\mathrm{serie}\: \\ $$$$\mathrm{I}\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\mathrm{x}^{−\mathrm{x}} \:\mathrm{dx}\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\mathrm{e}^{−\mathrm{xlnx}} \mathrm{dx}\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\left(\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\frac{\left(−\mathrm{xlnx}\right)^{\mathrm{n}} }{\mathrm{n}!}\right)\mathrm{dx} \\ $$$$=\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{\mathrm{n}} }{\mathrm{n}!}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\mathrm{x}^{\mathrm{n}} \:\left(\mathrm{lnx}\right)^{\mathrm{n}} \:\mathrm{dx}\:\mathrm{let}\:\mathrm{A}_{\mathrm{n}} =\int_{\mathrm{0}} ^{\mathrm{1}} \:\mathrm{x}^{\mathrm{n}} \left(\mathrm{lnx}\right)^{\mathrm{n}} \:\mathrm{dx} \\ $$$$\mathrm{lnx}\:=−\mathrm{t}\:\Rightarrow\mathrm{A}_{\mathrm{n}} =−\int_{\mathrm{0}} ^{\infty} \:\:\left(\mathrm{e}^{−\mathrm{t}} \right)^{\mathrm{n}} \:\left(−\mathrm{t}\right)^{\mathrm{n}} \:\left(−\mathrm{e}^{−\mathrm{t}} \right)\mathrm{dt} \\ $$$$=\left(−\mathrm{1}\right)^{\mathrm{n}} \:\int_{\mathrm{0}} ^{\infty} \:\mathrm{e}^{−\left(\mathrm{n}+\mathrm{1}\right)\mathrm{t}} \:\mathrm{t}^{\mathrm{n}} \:\mathrm{dt}\:\:=_{\left(\mathrm{n}+\mathrm{1}\right)\mathrm{t}\:=\mathrm{u}} \:\:\:\left(−\mathrm{1}\right)^{\mathrm{n}} \:\int_{\mathrm{0}} ^{\infty} \mathrm{e}^{−\mathrm{u}} \:\frac{\mathrm{u}^{\mathrm{n}} }{\left(\mathrm{n}+\mathrm{1}\right)^{\mathrm{n}} }×\frac{\mathrm{du}}{\mathrm{n}+\mathrm{1}} \\ $$$$=\frac{\left(−\mathrm{1}\right)^{\mathrm{n}} }{\left(\mathrm{n}+\mathrm{1}\right)^{\mathrm{n}+\mathrm{1}} }\:\int_{\mathrm{0}} ^{\infty} \:\mathrm{u}^{\mathrm{n}} \:\mathrm{e}^{−\mathrm{u}} \:\mathrm{du}\:=\frac{\left(−\mathrm{1}\right)^{\mathrm{n}} \:\Gamma\left(\mathrm{n}+\mathrm{1}\right)}{\left(\mathrm{n}+\mathrm{1}\right)^{\mathrm{n}+\mathrm{1}} }\:=\frac{\left(−\mathrm{1}\right)^{\mathrm{n}} \mathrm{n}!}{\left(\mathrm{n}+\mathrm{1}\right)^{\mathrm{n}+\mathrm{1}} }\:\Rightarrow \\ $$$$\mathrm{I}\:=\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{\mathrm{n}} }{\mathrm{n}!}×\frac{\left(−\mathrm{1}\right)^{\mathrm{n}} \mathrm{n}!}{\left(\mathrm{n}+\mathrm{1}\right)^{\mathrm{n}+\mathrm{1}} }\:=\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\frac{\mathrm{1}}{\left(\mathrm{n}+\mathrm{1}\right)^{\mathrm{n}+\mathrm{1}} }\:=\sum_{\mathrm{n}=\mathrm{1}} ^{\infty} \:\frac{\mathrm{1}}{\mathrm{n}^{\mathrm{n}} } \\ $$$$=\frac{\mathrm{1}}{\mathrm{1}^{\mathrm{1}} }\:+\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{2}} }\:+\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{3}} }+…. \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *