Question Number 149516 by mathdanisur last updated on 05/Aug/21
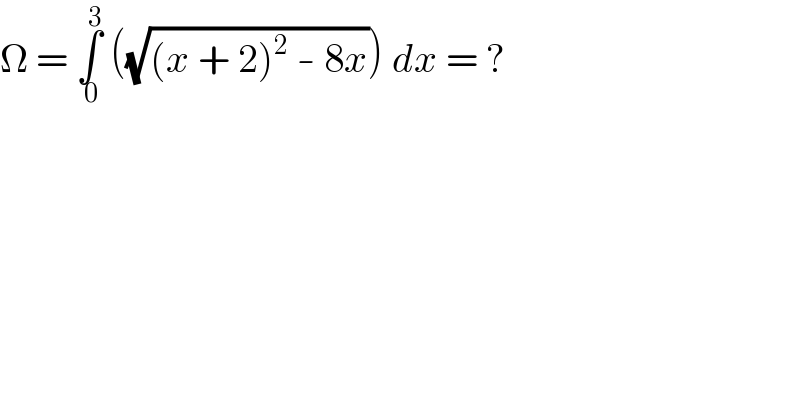
$$\Omega\:=\:\underset{\:\mathrm{0}} {\overset{\:\mathrm{3}} {\int}}\:\left(\sqrt{\left({x}\:+\:\mathrm{2}\right)^{\mathrm{2}} \:-\:\mathrm{8}{x}}\right)\:{dx}\:=\:? \\ $$
Answered by Ar Brandon last updated on 05/Aug/21
![=∫_0 ^3 (√(x^2 −4x+4))dx=∫_0 ^3 (√((x−2)^2 ))dx =∫_0 ^3 ∣x−2∣dx=∫_2 ^3 (x−2)dx−∫_0 ^2 (x−2)dx =[(x^2 /2)−2x]_2 ^3 −[(x^2 /2)−2x]_0 ^2 =((9/2)−6)−2(2−4) =−(3/2)+4=(5/2)](https://www.tinkutara.com/question/Q149518.png)
$$\:\:=\int_{\mathrm{0}} ^{\mathrm{3}} \sqrt{{x}^{\mathrm{2}} −\mathrm{4}{x}+\mathrm{4}}{dx}=\int_{\mathrm{0}} ^{\mathrm{3}} \sqrt{\left({x}−\mathrm{2}\right)^{\mathrm{2}} }{dx} \\ $$$$\:\:=\int_{\mathrm{0}} ^{\mathrm{3}} \mid{x}−\mathrm{2}\mid{dx}=\int_{\mathrm{2}} ^{\mathrm{3}} \left({x}−\mathrm{2}\right){dx}−\int_{\mathrm{0}} ^{\mathrm{2}} \left({x}−\mathrm{2}\right){dx} \\ $$$$\:\:=\left[\frac{{x}^{\mathrm{2}} }{\mathrm{2}}−\mathrm{2}{x}\right]_{\mathrm{2}} ^{\mathrm{3}} −\left[\frac{{x}^{\mathrm{2}} }{\mathrm{2}}−\mathrm{2}{x}\right]_{\mathrm{0}} ^{\mathrm{2}} =\left(\frac{\mathrm{9}}{\mathrm{2}}−\mathrm{6}\right)−\mathrm{2}\left(\mathrm{2}−\mathrm{4}\right) \\ $$$$\:\:=−\frac{\mathrm{3}}{\mathrm{2}}+\mathrm{4}=\frac{\mathrm{5}}{\mathrm{2}} \\ $$
Commented by mathdanisur last updated on 06/Aug/21

$$\mathrm{Thankyou}\:\boldsymbol{\mathrm{Ser}} \\ $$
