Question Number 117811 by Lordose last updated on 13/Oct/20
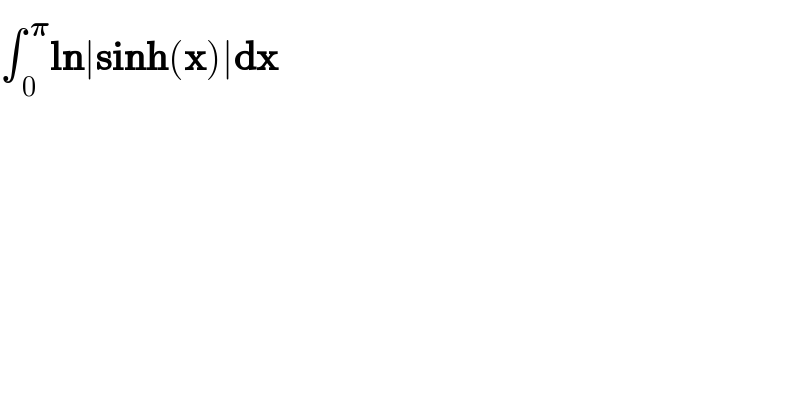
$$\int_{\:\mathrm{0}} ^{\:\boldsymbol{\pi}} \boldsymbol{\mathrm{ln}}\mid\boldsymbol{\mathrm{sinh}}\left(\boldsymbol{\mathrm{x}}\right)\mid\boldsymbol{\mathrm{dx}} \\ $$
Answered by mindispower last updated on 13/Oct/20
![=β«_0 ^Ο ln(sh(x))dx sh(x)=((e^x βe^(βx) )/2) =β«_0 ^Ο (ln(e^x (1βe^(β2x) ))βln(2))dx =β«_0 ^Ο (x+ln(1βe^(β2x) )βln(2))dx =[(1/2)x^2 βxln(2)]_0 ^Ο _(=A) +β«_0 ^Ο (ln(1βe^(β2x) )dx)_(=B) ln(1βe^(β2x) )=βΞ£_(nβ₯0) (e^(β2(n+1x) /(n+1)) β«_0 ^Ο ln(1βe^(β2x) )dx=βΞ£_(nβ₯0) (1/(n+1))β«_0 ^Ο e^(β2(n+)x) dx Ξ£_(nβ₯0) ((e^(β2(n+1)Ο) β1)/(2(n+1)^2 ))=Ξ£_(nβ₯1) (((e^(β2Ο) )^n )/n^2 )βΞ£_(nβ₯1) (1/(2n^2 )) Li_s (x)=Ξ£_(kβ₯1) (x^k /k^s ) we get=(1/2)(Li_2 (e^(β2Ο) )βLi_2 (1))=(1/2)Li_2 (e^(β2Ο) )β(1/2)ΞΆ(2) =(1/2)Li_2 (e^(β2Ο) )β(Ο^2 /(12)) A=(1/2)Ο^2 βΟln(2) A+B=((5Ο^2 )/(12))βΟln(2)+Li_2 (e^(β2Ο) ) β«_0 ^Ο ln(sh(x))dx=((5Ο^2 )/(12))βΟln(2)+Li_2 (e^(β2Ο) )](https://www.tinkutara.com/question/Q117815.png)
$$=\int_{\mathrm{0}} ^{\pi} {ln}\left({sh}\left({x}\right)\right){dx} \\ $$$${sh}\left({x}\right)=\frac{{e}^{{x}} β{e}^{β{x}} }{\mathrm{2}} \\ $$$$=\int_{\mathrm{0}} ^{\pi} \left({ln}\left({e}^{{x}} \:\left(\mathrm{1}β{e}^{β\mathrm{2}{x}} \right)\right)β{ln}\left(\mathrm{2}\right)\right){dx} \\ $$$$=\int_{\mathrm{0}} ^{\pi} \left({x}+{ln}\left(\mathrm{1}β{e}^{β\mathrm{2}{x}} \right)β{ln}\left(\mathrm{2}\right)\right){dx} \\ $$$$=\left[\frac{\mathrm{1}}{\mathrm{2}}{x}^{\mathrm{2}} β{xln}\left(\mathrm{2}\right)\right]_{\mathrm{0}} ^{\pi} \:\underset{={A}} {\:}+\int_{\mathrm{0}} ^{\pi} \left({ln}\left(\mathrm{1}β{e}^{β\mathrm{2}{x}} \right){dx}\underset{={B}} {\right)} \\ $$$${ln}\left(\mathrm{1}β{e}^{β\mathrm{2}{x}} \right)=β\underset{{n}\geqslant\mathrm{0}} {\sum}\frac{{e}^{β\mathrm{2}\left({n}+\mathrm{1}{x}\right.} }{{n}+\mathrm{1}} \\ $$$$\int_{\mathrm{0}} ^{\pi} {ln}\left(\mathrm{1}β{e}^{β\mathrm{2}{x}} \right){dx}=β\underset{{n}\geqslant\mathrm{0}} {\sum}\frac{\mathrm{1}}{{n}+\mathrm{1}}\int_{\mathrm{0}} ^{\pi} {e}^{β\mathrm{2}\left({n}+\right){x}} {dx} \\ $$$$\underset{{n}\geqslant\mathrm{0}} {\sum}\frac{{e}^{β\mathrm{2}\left({n}+\mathrm{1}\right)\pi} β\mathrm{1}}{\mathrm{2}\left({n}+\mathrm{1}\right)^{\mathrm{2}} }=\underset{{n}\geqslant\mathrm{1}} {\sum}\frac{\left({e}^{β\mathrm{2}\pi} \right)^{{n}} }{{n}^{\mathrm{2}} }β\underset{{n}\geqslant\mathrm{1}} {\sum}\frac{\mathrm{1}}{\mathrm{2}{n}^{\mathrm{2}} } \\ $$$${Li}_{{s}} \left({x}\right)=\underset{{k}\geqslant\mathrm{1}} {\sum}\frac{{x}^{{k}} }{{k}^{{s}} } \\ $$$${we}\:{get}=\frac{\mathrm{1}}{\mathrm{2}}\left({Li}_{\mathrm{2}} \left({e}^{β\mathrm{2}\pi} \right)β{Li}_{\mathrm{2}} \left(\mathrm{1}\right)\right)=\frac{\mathrm{1}}{\mathrm{2}}{Li}_{\mathrm{2}} \left({e}^{β\mathrm{2}\pi} \right)β\frac{\mathrm{1}}{\mathrm{2}}\zeta\left(\mathrm{2}\right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}{Li}_{\mathrm{2}} \left({e}^{β\mathrm{2}\pi} \right)β\frac{\pi^{\mathrm{2}} }{\mathrm{12}} \\ $$$${A}=\frac{\mathrm{1}}{\mathrm{2}}\pi^{\mathrm{2}} β\pi{ln}\left(\mathrm{2}\right) \\ $$$${A}+{B}=\frac{\mathrm{5}\pi^{\mathrm{2}} }{\mathrm{12}}β\pi{ln}\left(\mathrm{2}\right)+{Li}_{\mathrm{2}} \left({e}^{β\mathrm{2}\pi} \right) \\ $$$$\int_{\mathrm{0}} ^{\pi} {ln}\left({sh}\left({x}\right)\right){dx}=\frac{\mathrm{5}\pi^{\mathrm{2}} }{\mathrm{12}}β\pi{ln}\left(\mathrm{2}\right)+{Li}_{\mathrm{2}} \left({e}^{β\mathrm{2}\pi} \right) \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$