Question Number 145385 by mnjuly1970 last updated on 04/Jul/21
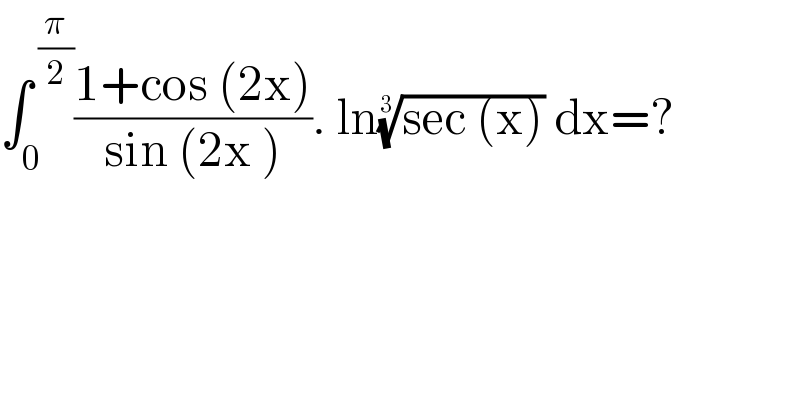
$$\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \frac{\mathrm{1}+\mathrm{cos}\:\left(\mathrm{2x}\right)}{\mathrm{sin}\:\left(\mathrm{2x}\:\right)}.\:\mathrm{ln}\sqrt[{\mathrm{3}}]{\mathrm{sec}\:\left(\mathrm{x}\right)}\:\mathrm{dx}=? \\ $$
Answered by mindispower last updated on 04/Jul/21
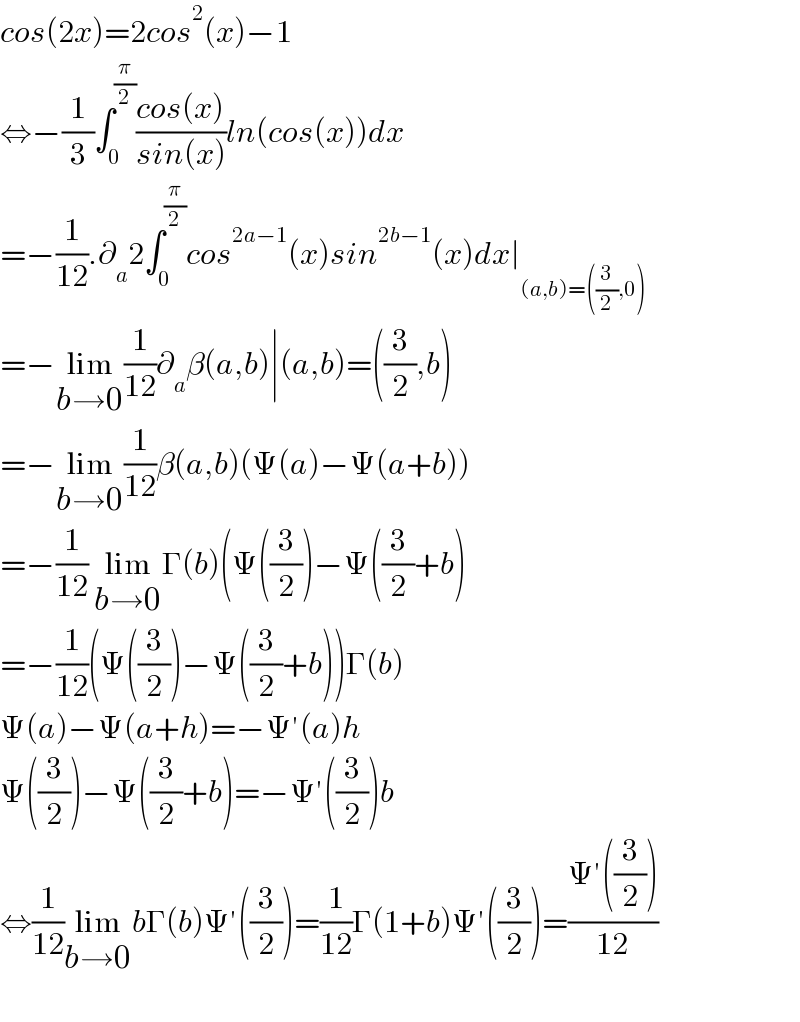
$${cos}\left(\mathrm{2}{x}\right)=\mathrm{2}{cos}^{\mathrm{2}} \left({x}\right)−\mathrm{1} \\ $$$$\Leftrightarrow−\frac{\mathrm{1}}{\mathrm{3}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{{cos}\left({x}\right)}{{sin}\left({x}\right)}{ln}\left({cos}\left({x}\right)\right){dx} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{12}}.\partial_{{a}} \mathrm{2}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {cos}^{\mathrm{2}{a}−\mathrm{1}} \left({x}\right){sin}^{\mathrm{2}{b}−\mathrm{1}} \left({x}\right){dx}\mid_{\left({a},{b}\right)=\left(\frac{\mathrm{3}}{\mathrm{2}},\mathrm{0}\right)} \\ $$$$=−\underset{{b}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{1}}{\mathrm{12}}\partial_{{a}} \beta\left({a},{b}\right)\mid\left({a},{b}\right)=\left(\frac{\mathrm{3}}{\mathrm{2}},{b}\right) \\ $$$$=−\underset{{b}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{1}}{\mathrm{12}}\beta\left({a},{b}\right)\left(\Psi\left({a}\right)−\Psi\left({a}+{b}\right)\right) \\ $$$$=−\frac{\mathrm{1}}{\mathrm{12}}\:\underset{{b}\rightarrow\mathrm{0}} {\mathrm{lim}}\Gamma\left({b}\right)\left(\Psi\left(\frac{\mathrm{3}}{\mathrm{2}}\right)−\Psi\left(\frac{\mathrm{3}}{\mathrm{2}}+{b}\right)\right. \\ $$$$=−\frac{\mathrm{1}}{\mathrm{12}}\left(\Psi\left(\frac{\mathrm{3}}{\mathrm{2}}\right)−\Psi\left(\frac{\mathrm{3}}{\mathrm{2}}+{b}\right)\right)\Gamma\left({b}\right) \\ $$$$\Psi\left({a}\right)−\Psi\left({a}+{h}\right)=−\Psi'\left({a}\right){h} \\ $$$$\Psi\left(\frac{\mathrm{3}}{\mathrm{2}}\right)−\Psi\left(\frac{\mathrm{3}}{\mathrm{2}}+{b}\right)=−\Psi'\left(\frac{\mathrm{3}}{\mathrm{2}}\right){b} \\ $$$$\Leftrightarrow\frac{\mathrm{1}}{\mathrm{12}}\underset{{b}\rightarrow\mathrm{0}} {\mathrm{lim}}{b}\Gamma\left({b}\right)\Psi'\left(\frac{\mathrm{3}}{\mathrm{2}}\right)=\frac{\mathrm{1}}{\mathrm{12}}\Gamma\left(\mathrm{1}+{b}\right)\Psi'\left(\frac{\mathrm{3}}{\mathrm{2}}\right)=\frac{\Psi'\left(\frac{\mathrm{3}}{\mathrm{2}}\right)}{\mathrm{12}} \\ $$$$ \\ $$
Commented by mnjuly1970 last updated on 04/Jul/21
$${thank}\:{you}\:{so}\:{much}.. \\ $$
Commented by mindispower last updated on 04/Jul/21

$${pleasur}\:{sir} \\ $$
