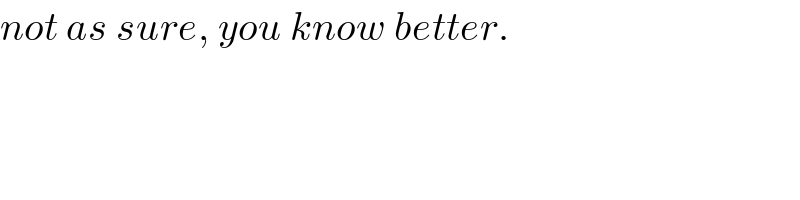0-pi-cos-x-1-2sin-2x-dx-

Question Number 34992 by MJS last updated on 14/May/18

\underset{0}{\int^{π}} \frac{\cos (x)}{1 + 2 \sin (2 x)} d x

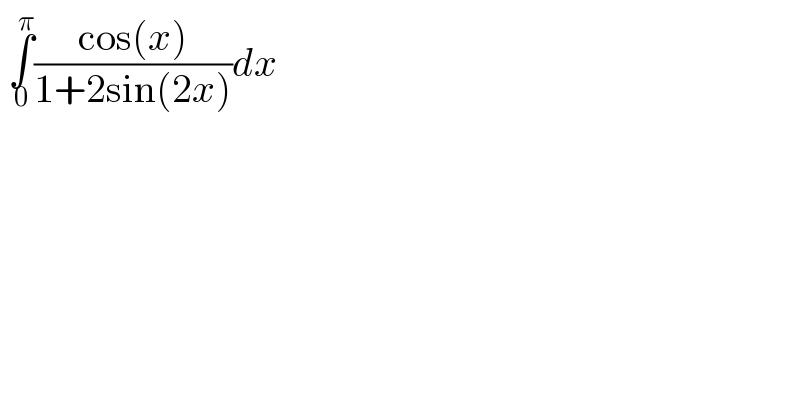
Commented by math khazana by abdo last updated on 14/May/18

l e t p u t I = \int_{0}^{π} \frac{c o s x}{1 + 2 s i n (2 x)} d x

I = \int_{0}^{π} \frac{c o s x}{1 + 4 c o s x s i n x} d x . c h a n g e m e n t t a n (\frac{x}{2}) = t

g i v e I = \int_{0}^{\infty} \frac{\frac{1 - t^{2}}{1 + t^{2}}}{1 + 4 \frac{1 - t^{2}}{1 + t^{2}} \frac{2 t}{1 + t^{2}}} \frac{2 d t}{1 + t^{2}}

= 2 \int_{0}^{\infty} \frac{1 - t^{2}}{{(1 + t^{2})}^{2} (1 + \frac{8 t (1 - t^{2})}{{(1 + t^{2})}^{2}})} d t

= 2 \int_{0}^{\infty} \frac{1 - t^{2}}{{(1 + t^{2})}^{2} + 8 t (1 - t^{2})} d t

= 2 \int_{0}^{\infty} \frac{1 - t^{2}}{t^{4} + 2 t^{2} + 1 + 8 t - 8 t^{3}} d t

= 2 \int_{0}^{\infty} \frac{1 - t^{2}}{t^{4} - 8 t^{3} + 2 t^{2} + 8 t + 1} d t l e t d r c o m p o s e

F (t) = \frac{1 - t^{2}}{t^{4} - 8 t^{3} + 2 t^{2} + 8 t + 1}

t h e r o o t s o f p (t) = t^{4} - 8 t^{3} + 2 t^{2} + 8 t + 1 a r e

t_{1} \sim 7, 59 t_{2} \sim 1, 3 t_{3} \sim - 0, 76 t_{4} \sim - 0, 13 \Rightarrow

F (t) = \frac{1 - t^{2}}{(t - t_{1}) (t - t_{2}) (t - t_{3}) (t - t_{4})}

= \frac{a}{t - t_{1}} + \frac{b}{t - t_{2}} + \frac{c}{t - t_{3}} + \frac{d}{t - t_{4}}

a = {l i m}_{t \to t_{1}} (t - t_{1}) F (t) = \frac{1 - t_{1}^{2}}{(t_{1} - t_{2}) (t_{1} - t_{3}) (t_{1} - t_{4})}

= ξ (t_{1}) (1 - t_{1}^{2}) w i t h ξ (t_{1}) = \frac{1}{(t_{1} - t_{2}) (t_{1} - t_{3}) (t_{1} - t_{4})}

b = ξ (t_{2}) (1 - t_{2}^{2}) w i t h ξ (t_{2}) = \frac{1}{(t_{2} - t_{1}) (t_{2} - t_{3}) (t_{2} - t_{4})}

c = ξ (t_{3}) (1 - t_{3}^{2})

d = ξ (t_{4}) (1 - t_{4}^{2}) \Rightarrow

I = 2 \int_{0}^{\infty} F (t) d t

{= 2 [a l n ∣ t - t_{1} ∣ + b l n ∣ t - t_{2} ∣ + c l n ∣ t - t_{3}) + d l n ∣ t - t_{4} ∣]}_{0}^{+ \infty}

= 2 {[l n ∣ \frac{(t - t_{1}) a}{{(t - t_{2})}^{b}} ∣ + l n ∣ \frac{{(t - t_{3})}^{c}}{{(t - t_{4})}^{d}} ∣]}_{0}^{+ \infty}

= 2 {- l n ∣ \frac{{(- t_{1})}^{a}}{{(- t_{2})}^{b}} ∣ - l n ∣ \frac{{(- t_{3})}^{c}}{{(- t_{4})}^{d}} ∣}

= 2 {b l n ∣ t_{2} ∣ - a l n ∣ t_{1} ∣ + d l n ∣ t_{4} ∣ - c l n ∣ t_{3} ∣} \dots

t h e a p p r o x i m a t e v a l u e o f I i s d e t e r m i n e d \dots

Answered by MJS last updated on 14/May/18

\underset{0}{\int^{π}} \frac{\cos (x)}{1 + 2 \sin (2 x)} d x = \underset{- \frac{π}{2}}{\int^{\frac{π}{2}}} \frac{\sin (x)}{2 \sin (2 x) - 1} d x =

= \underset{- \frac{π}{2}}{\int^{\frac{π}{2}}} \frac{\sin (x)}{4 \sin (x) \cos (x) - 1} d x =

[\begin{matrix} t = \tan (\frac{x}{2}) \to d x = \frac{2 d t}{t^{2} + 1} \\ \sin (x) = \frac{2 t}{t^{2} + 1}; \cos (x) = - \frac{t^{2} - 1}{t^{2} + 1} \end{matrix}]

= - 4 \underset{- 1}{\int^{1}} \frac{t}{t^{4} + 8 t^{3} + 2 t^{2} - 8 t + 1} d t =

[\begin{matrix} t^{4} + 8 t^{3} + 2 t^{2} - 8 t + 1 = 0 \\ t_{1} = - 2 - \sqrt{6} - \sqrt{3} - \sqrt{2} \\ t_{2} = - 2 - \sqrt{6} + \sqrt{3} + \sqrt{2} \\ t_{3} = - 2 + \sqrt{6} - \sqrt{3} + \sqrt{2} \\ t_{4} = - 2 + \sqrt{6} + \sqrt{3} - \sqrt{2} \end{matrix}]

= - 4 \underset{- 1}{\int^{1}} (\frac{A}{t - t_{1}} + \frac{B}{t - t_{2}} + \frac{C}{t - t_{3}} + \frac{D}{t - t_{4}}) d t =

= - 4 {[A \ln ∣ t - t_{1} ∣ + B \ln ∣ t - t_{2} ∣ + C \ln ∣ t - t_{3} ∣ + D \ln ∣ t - t_{4} ∣]}_{- 1}^{1} =

Missing \left or extra \right

[\begin{matrix} A = \frac{t_{1}}{(t_{1} - t_{2}) (t_{1} - t_{3}) (t_{1} - t_{4})} = \frac{- \sqrt{6} + 3 \sqrt{2}}{96} \\ B = \frac{t_{2}}{(t_{2} - t_{1}) (t_{2} - t_{3}) (t_{2} - t_{4})} = \frac{- \sqrt{6} - 3 \sqrt{2}}{96} \\ C = \frac{t_{3}}{(t_{3} - t_{1}) (t_{3} - t_{2}) (t_{3} - t_{4})} = \frac{\sqrt{6} - 3 \sqrt{2}}{96} \\ D = \frac{t_{4}}{(t_{4} - t_{1}) (t_{4} - t_{2}) (t_{4} - t_{3})} = \frac{\sqrt{6} + 3 \sqrt{2}}{96} \end{matrix}]

Missing \left or extra \right

\approx 1.091166

Commented by MJS last updated on 14/May/18

I {couldn}^{'} t get this out of my head \dots

this should be the solution, please check for

mistakes of method .

I can guarantee the correctness of the zeros

t_{1}, t_{2}, t_{3}, t_{4} and the coefficients A, B, C, D .

Commented by ajfour last updated on 14/May/18

f o r x = \frac{π}{12}, \frac{\sin x}{2 \sin 2 x - 1} i s n o t d e f i n e d .

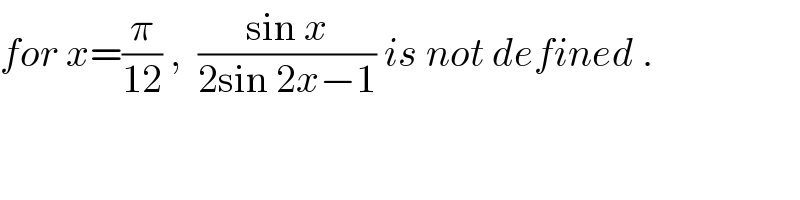
Commented by MJS last updated on 14/May/18

but {it}^{'} s the Cauchy principal value :

\int \frac{p}{x - q} d x = p \ln ∣ x - q ∣

a < q < b; h > 0

\underset{a}{\int^{b}} \frac{p}{x - q} d x = \lim_{h \to 0} (\underset{a}{\int^{q - h}} \frac{p}{x - q} d x + \underset{q + h}{\int^{b}} \frac{p}{x - q} d x) =

= \lim_{h \to 0} ({[p \ln ∣ x - q ∣]}_{a}^{q - h} + {[p \ln ∣ x - q ∣]}_{q + h}^{b}) =

= \lim_{h \to 0} (p \ln ∣ h ∣ - p \ln ∣ a - q ∣ + p \ln ∣ b - q ∣ - p \ln ∣ h ∣) =

= \lim_{h \to 0} (p \ln ∣ b - q ∣ - p \ln ∣ a - q ∣) =

= p \ln ∣ b - q ∣ - p \ln ∣ a - q ∣= \underset{a}{\int^{b}} \frac{p}{x - q} d x

q . e . d .

Commented by ajfour last updated on 14/May/18

n o t a s s u r e, y o u k n o w b e t t e r .

Facebook Tweet Pin