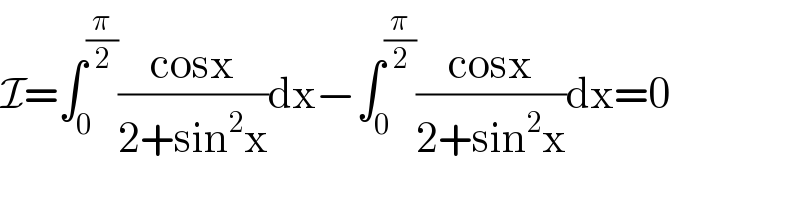0-pi-cosx-2-sin-2-x-dx-

Question Number 107051 by Ar Brandon last updated on 08/Aug/20

\int_{0}^{π} \frac{cosx}{2 + \sin^{2} x} dx

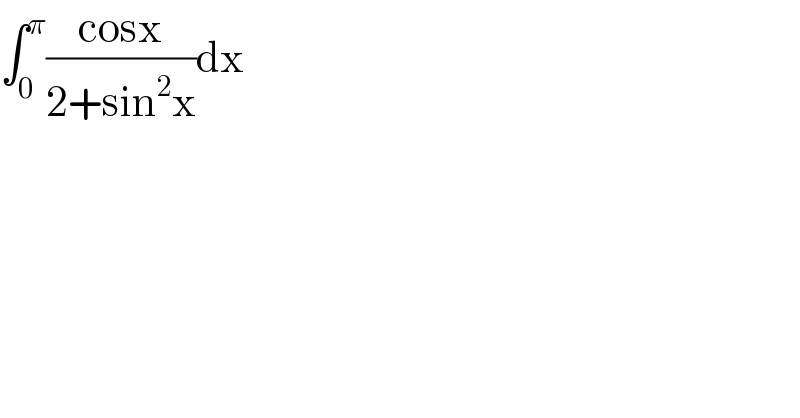
Answered by Dwaipayan Shikari last updated on 08/Aug/20

\int_{0}^{π} \frac{dt}{2 + t^{2}} (sinx = t)

\frac{1}{\sqrt{2}} \tan^{- 1} (\frac{t}{\sqrt{2}}) + C = {[\frac{1}{\sqrt{2}} \tan^{- 1} (\frac{sinx}{\sqrt{2}})]}_{0}^{π} = 0

Commented by Ar Brandon last updated on 08/Aug/20

OK, I got it now��

Commented by Dwaipayan Shikari last updated on 08/Aug/20

��

Answered by Ar Brandon last updated on 08/Aug/20

I = \int_{0}^{\frac{π}{2}} \frac{cosx}{2 + \sin^{2} x} dx - \int_{0}^{\frac{π}{2}} \frac{cosx}{2 + \sin^{2} x} dx = 0