0-pi-x-1-cos-2-x-dx-

Question Number 106932 by Ar Brandon last updated on 07/Aug/20

\int_{0}^{π} \frac{x}{1 + \cos^{2} x} dx

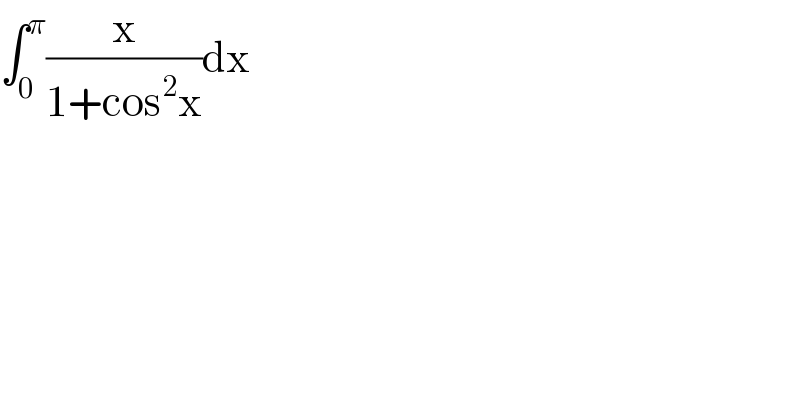
Answered by mathmax by abdo last updated on 08/Aug/20

I = \int_{0}^{π} \frac{xdx}{1 + \cos^{2} x} changement x = π - t give

I = \int_{0}^{π} \frac{(π - t) dt}{1 + \cos^{2} t} = π \int_{0}^{π} \frac{dt}{1 + \cos^{2} t} - I \Rightarrow 2 I = π \int_{0}^{π} \frac{dt}{1 + \cos^{2} t}

but we have proved \int_{0}^{π} \frac{dt}{1 + \cos^{2} t} = \frac{π}{\sqrt{2}} \Rightarrow 2 I = \frac{π^{2}}{\sqrt{2}} \Rightarrow I = \frac{π^{2}}{2 \sqrt{2}}

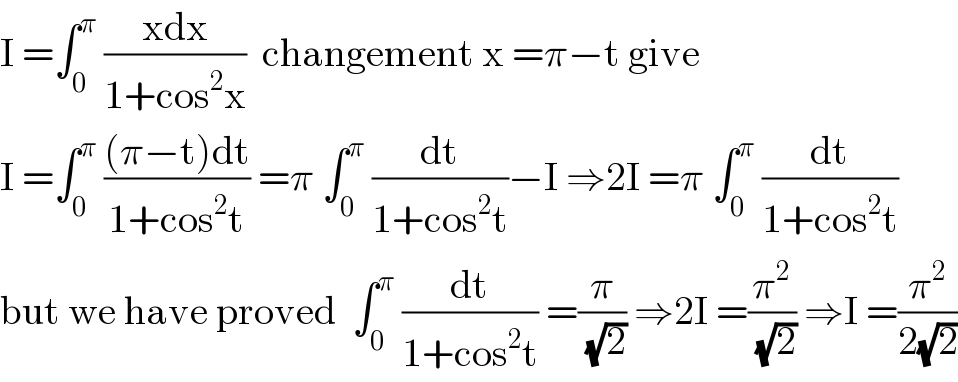
Answered by Dwaipayan Shikari last updated on 08/Aug/20

\int_{0}^{π} \frac{x}{1 + {c o s}^{2} x} = \int \frac{π - x}{1 + {c o s}^{2} x} = I

2 I = \int_{0}^{π} \frac{π}{1 + {c o s}^{2} x}

2 I = π \int_{0}^{π} \frac{{s e c}^{2} x}{{s e c}^{2} x + 1}

2 I = π \int_{0}^{π} \frac{d t}{t^{2} + 2} (t a n x = t

2 I = \frac{π}{\sqrt{2}} {[{t a n}^{- 1} (\frac{tanx}{\sqrt{2}})]}_{0}^{π} = \frac{π}{\sqrt{2}} . π = \frac{π^{2}}{\sqrt{2}}

I = \frac{π^{2}}{2 \sqrt{2}}

Commented by Ar Brandon last updated on 08/Aug/20

��

Answered by Ar Brandon last updated on 08/Aug/20

J = \int_{0}^{π} \frac{x}{1 + \cos^{2} x} dx

= \int_{0}^{π} \frac{π - x}{1 + \cos^{2} x} dx = \int_{0}^{π} \frac{π}{1 + \cos^{2} x} dx - J

2 J = \int_{0}^{π} \frac{π}{1 + \cos^{2} x} dx = π \int_{0}^{π} \frac{\sec^{2} x}{\sec^{2} x + 1} dx

= π \int_{0}^{π} \frac{\sec^{2} x}{\tan^{2} x + 2} dx = π \int_{0}^{π} \frac{d (tanx)}{\tan^{2} x + 2} dx

= π {[\frac{1}{\sqrt{2}} Arctan (\frac{tanx}{\sqrt{2}})]}_{0}^{π}

= \frac{π}{\sqrt{2}} [Arctan (- \frac{0}{\sqrt{2}}) - Arctan (\frac{0}{\sqrt{2}})] = \frac{π (π - 0)}{\sqrt{2}} = \frac{π^{2}}{\sqrt{2}}

J = \frac{π^{2}}{2 \sqrt{2}}