Question Number 123495 by bemath last updated on 25/Nov/20
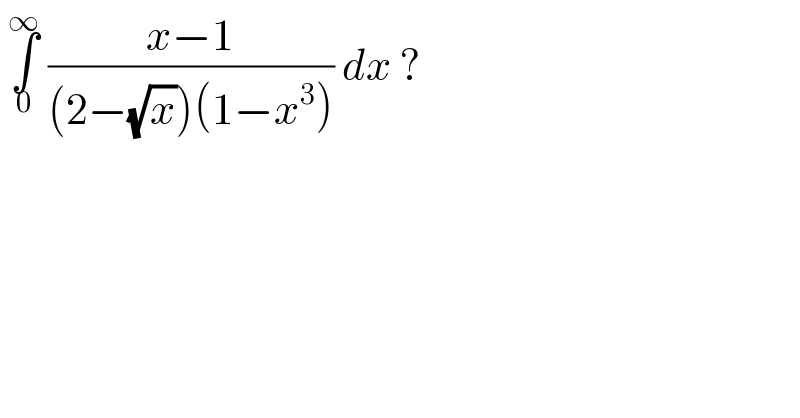
$$\:\underset{\mathrm{0}} {\overset{\infty} {\int}}\:\frac{{x}−\mathrm{1}}{\left(\mathrm{2}−\sqrt{{x}}\right)\left(\mathrm{1}−{x}^{\mathrm{3}} \right)}\:{dx}\:? \\ $$
Answered by MJS_new last updated on 26/Nov/20
![∫((x−1)/((2+(√x))(1−x^3 )))dx=∫(dx/(((√x)−2)(x^2 +x+1)))= [t=(√x) → dx=2(√x)dt] =2∫(t/((t−2)(t^2 −t+1)(t^2 +t+1)))dt= =(4/(21))∫(dt/(t−2))−(1/3)∫((t+1)/(t^2 −t+1))dt+(1/7)∫((t+3)/(t^2 +t+1))= =(4/(21))∫(dt/(t−2))−(1/6)∫((2t−1)/(t^2 −t+1))dt−(1/2)∫(dt/(t^2 −t+1))+(1/(14))∫((2t+1)/(t^2 +t+1))dt+(5/(14))∫(dt/(t^2 +t+1))= =(4/(21))ln (t−2) −(1/6)ln (t^2 −t+1) −((√3)/3)arctan (((√3)(2t−1))/3) +(1/(14))ln (t^2 +t+1) +((5(√3))/(21))arctan (((√3)(2t+1))/3) = =(4/(21))ln ∣2−(√x)∣ −(1/6)ln (x+1−(√x)) +((√3)/3)arctan (((√3)(1−2(√x)))/3) +(1/(14))ln (x+1+(√x)) +((5(√3))/(21))arctan (((√3)(1+2(√x)))/3) +C ⇒ the integral doesn′t converge](https://www.tinkutara.com/question/Q123500.png)
$$\int\frac{{x}−\mathrm{1}}{\left(\mathrm{2}+\sqrt{{x}}\right)\left(\mathrm{1}−{x}^{\mathrm{3}} \right)}{dx}=\int\frac{{dx}}{\left(\sqrt{{x}}−\mathrm{2}\right)\left({x}^{\mathrm{2}} +{x}+\mathrm{1}\right)}= \\ $$$$\:\:\:\:\:\left[{t}=\sqrt{{x}}\:\rightarrow\:{dx}=\mathrm{2}\sqrt{{x}}{dt}\right] \\ $$$$=\mathrm{2}\int\frac{{t}}{\left({t}−\mathrm{2}\right)\left({t}^{\mathrm{2}} −{t}+\mathrm{1}\right)\left({t}^{\mathrm{2}} +{t}+\mathrm{1}\right)}{dt}= \\ $$$$=\frac{\mathrm{4}}{\mathrm{21}}\int\frac{{dt}}{{t}−\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{3}}\int\frac{{t}+\mathrm{1}}{{t}^{\mathrm{2}} −{t}+\mathrm{1}}{dt}+\frac{\mathrm{1}}{\mathrm{7}}\int\frac{{t}+\mathrm{3}}{{t}^{\mathrm{2}} +{t}+\mathrm{1}}= \\ $$$$=\frac{\mathrm{4}}{\mathrm{21}}\int\frac{{dt}}{{t}−\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{6}}\int\frac{\mathrm{2}{t}−\mathrm{1}}{{t}^{\mathrm{2}} −{t}+\mathrm{1}}{dt}−\frac{\mathrm{1}}{\mathrm{2}}\int\frac{{dt}}{{t}^{\mathrm{2}} −{t}+\mathrm{1}}+\frac{\mathrm{1}}{\mathrm{14}}\int\frac{\mathrm{2}{t}+\mathrm{1}}{{t}^{\mathrm{2}} +{t}+\mathrm{1}}{dt}+\frac{\mathrm{5}}{\mathrm{14}}\int\frac{{dt}}{{t}^{\mathrm{2}} +{t}+\mathrm{1}}= \\ $$$$=\frac{\mathrm{4}}{\mathrm{21}}\mathrm{ln}\:\left({t}−\mathrm{2}\right)\:−\frac{\mathrm{1}}{\mathrm{6}}\mathrm{ln}\:\left({t}^{\mathrm{2}} −{t}+\mathrm{1}\right)\:−\frac{\sqrt{\mathrm{3}}}{\mathrm{3}}\mathrm{arctan}\:\frac{\sqrt{\mathrm{3}}\left(\mathrm{2}{t}−\mathrm{1}\right)}{\mathrm{3}}\:+\frac{\mathrm{1}}{\mathrm{14}}\mathrm{ln}\:\left({t}^{\mathrm{2}} +{t}+\mathrm{1}\right)\:+\frac{\mathrm{5}\sqrt{\mathrm{3}}}{\mathrm{21}}\mathrm{arctan}\:\frac{\sqrt{\mathrm{3}}\left(\mathrm{2}{t}+\mathrm{1}\right)}{\mathrm{3}}\:= \\ $$$$=\frac{\mathrm{4}}{\mathrm{21}}\mathrm{ln}\:\mid\mathrm{2}−\sqrt{{x}}\mid\:−\frac{\mathrm{1}}{\mathrm{6}}\mathrm{ln}\:\left({x}+\mathrm{1}−\sqrt{{x}}\right)\:+\frac{\sqrt{\mathrm{3}}}{\mathrm{3}}\mathrm{arctan}\:\frac{\sqrt{\mathrm{3}}\left(\mathrm{1}−\mathrm{2}\sqrt{{x}}\right)}{\mathrm{3}}\:+\frac{\mathrm{1}}{\mathrm{14}}\mathrm{ln}\:\left({x}+\mathrm{1}+\sqrt{{x}}\right)\:+\frac{\mathrm{5}\sqrt{\mathrm{3}}}{\mathrm{21}}\mathrm{arctan}\:\frac{\sqrt{\mathrm{3}}\left(\mathrm{1}+\mathrm{2}\sqrt{{x}}\right)}{\mathrm{3}}\:+{C} \\ $$$$\Rightarrow \\ $$$$\mathrm{the}\:\mathrm{integral}\:\mathrm{doesn}'\mathrm{t}\:\mathrm{converge} \\ $$
