0-x-1-n-x-3-x-2-x-1-dx-

Question Number 156860 by amin96 last updated on 16/Oct/21

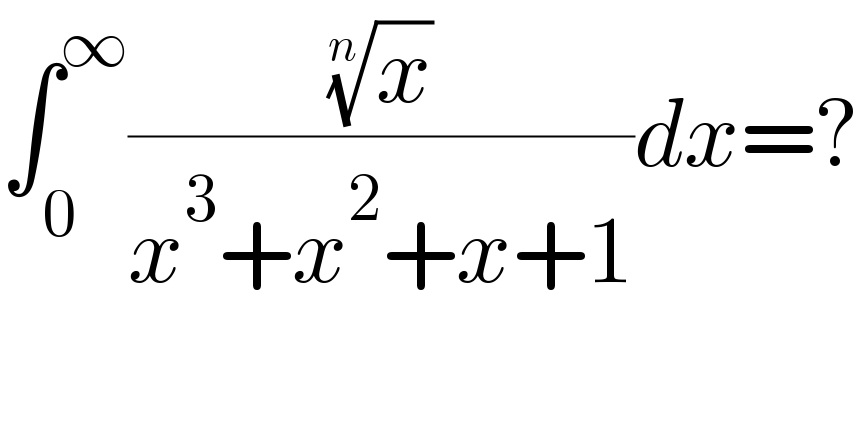
$$\int_{\mathrm{0}} ^{\infty} \frac{\sqrt[{{n}}]{{x}}}{{x}^{\mathrm{3}} +{x}^{\mathrm{2}} +{x}+\mathrm{1}}{dx}=? \\ $$

Answered by mindispower last updated on 16/Oct/21

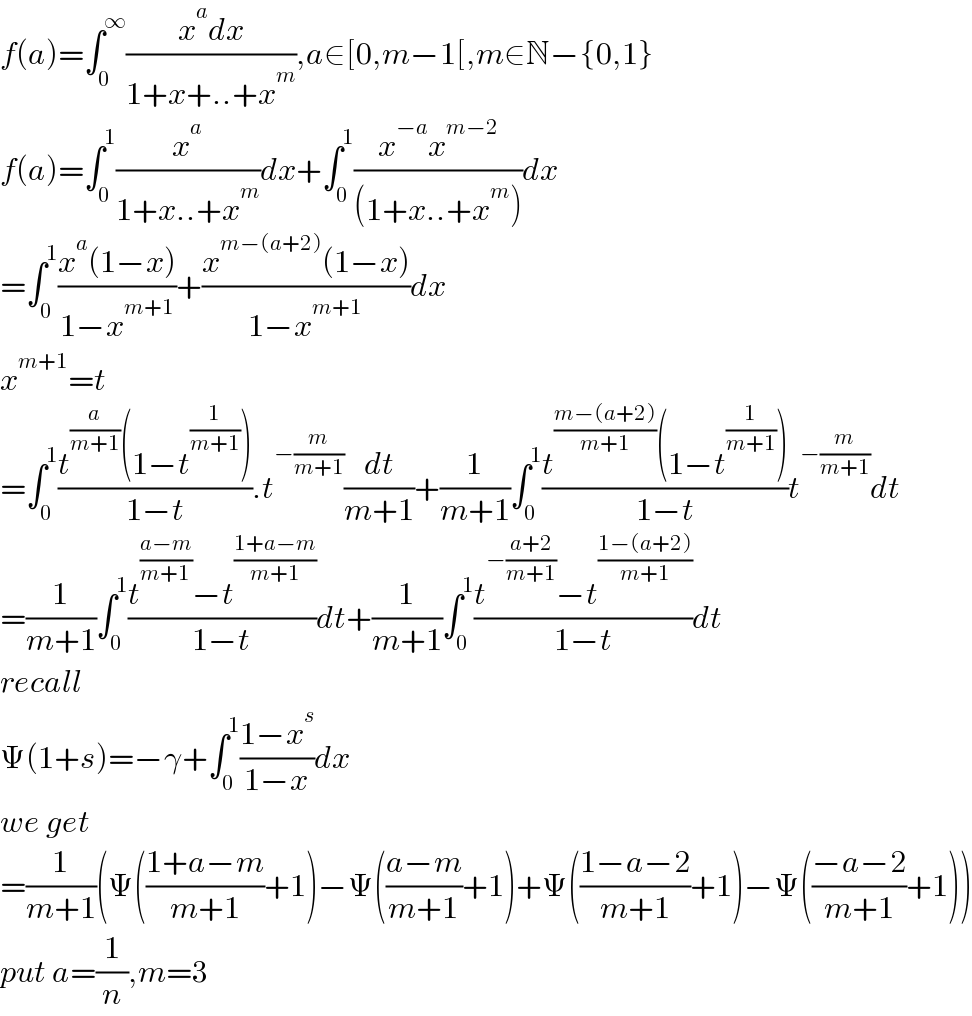
f(a)=∫_0 ^∞ ((x^a dx)/(1+x+..+x^m )),a∈[0,m−1[,m∈N−{0,1} f(a)=∫_0 ^1 (x^a /(1+x..+x^m ))dx+∫_0 ^1 ((x^(−a) x^(m−2) )/((1+x..+x^m )))dx =∫_0 ^1 ((x^a (1−x))/(1−x^(m+1) ))+((x^(m−(a+2)) (1−x))/(1−x^(m+1) ))dx x^(m+1) =t =∫_0 ^1 ((t^(a/(m+1)) (1−t^(1/(m+1)) ))/(1−t)).t^(−(m/(m+1))) (dt/(m+1))+(1/(m+1))∫_0 ^1 ((t^((m−(a+2))/(m+1)) (1−t^(1/(m+1)) ))/(1−t))t^(−(m/(m+1))) dt =(1/(m+1))∫_0 ^1 ((t^((a−m)/(m+1)) −t^((1+a−m)/(m+1)) )/(1−t))dt+(1/(m+1))∫_0 ^1 ((t^(−((a+2)/(m+1))) −t^((1−(a+2))/(m+1)) )/(1−t))dt recall Ψ(1+s)=−γ+∫_0 ^1 ((1−x^s )/(1−x))dx we get =(1/(m+1))(Ψ(((1+a−m)/(m+1))+1)−Ψ(((a−m)/(m+1))+1)+Ψ(((1−a−2)/(m+1))+1)−Ψ(((−a−2)/(m+1))+1)) put a=(1/n),m=3

$${f}\left({a}\right)=\int_{\mathrm{0}} ^{\infty} \frac{{x}^{{a}} {dx}}{\mathrm{1}+{x}+..+{x}^{{m}} },{a}\in\left[\mathrm{0},{m}−\mathrm{1}\left[,{m}\in\mathbb{N}−\left\{\mathrm{0},\mathrm{1}\right\}\right.\right. \\ $$$${f}\left({a}\right)=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{{a}} }{\mathrm{1}+{x}..+{x}^{{m}} }{dx}+\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{−{a}} {x}^{{m}−\mathrm{2}} }{\left(\mathrm{1}+{x}..+{x}^{{m}} \right)}{dx} \\ $$$$=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{{a}} \left(\mathrm{1}−{x}\right)}{\mathrm{1}−{x}^{{m}+\mathrm{1}} }+\frac{{x}^{{m}−\left({a}+\mathrm{2}\right)} \left(\mathrm{1}−{x}\right)}{\mathrm{1}−{x}^{{m}+\mathrm{1}} }{dx} \\ $$$${x}^{{m}+\mathrm{1}} ={t} \\ $$$$=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{t}^{\frac{{a}}{{m}+\mathrm{1}}} \left(\mathrm{1}−{t}^{\frac{\mathrm{1}}{{m}+\mathrm{1}}} \right)}{\mathrm{1}−{t}}.{t}^{−\frac{{m}}{{m}+\mathrm{1}}} \frac{{dt}}{{m}+\mathrm{1}}+\frac{\mathrm{1}}{{m}+\mathrm{1}}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{t}^{\frac{{m}−\left({a}+\mathrm{2}\right)}{{m}+\mathrm{1}}} \left(\mathrm{1}−{t}^{\frac{\mathrm{1}}{{m}+\mathrm{1}}} \right)}{\mathrm{1}−{t}}{t}^{−\frac{{m}}{{m}+\mathrm{1}}} {dt} \\ $$$$=\frac{\mathrm{1}}{{m}+\mathrm{1}}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{t}^{\frac{{a}−{m}}{{m}+\mathrm{1}}} −{t}^{\frac{\mathrm{1}+{a}−{m}}{{m}+\mathrm{1}}} }{\mathrm{1}−{t}}{dt}+\frac{\mathrm{1}}{{m}+\mathrm{1}}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{t}^{−\frac{{a}+\mathrm{2}}{{m}+\mathrm{1}}} −{t}^{\frac{\mathrm{1}−\left({a}+\mathrm{2}\right)}{{m}+\mathrm{1}}} }{\mathrm{1}−{t}}{dt} \\ $$$${recall} \\ $$$$\Psi\left(\mathrm{1}+{s}\right)=−\gamma+\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{1}−{x}^{{s}} }{\mathrm{1}−{x}}{dx} \\ $$$${we}\:{get} \\ $$$$=\frac{\mathrm{1}}{{m}+\mathrm{1}}\left(\Psi\left(\frac{\mathrm{1}+{a}−{m}}{{m}+\mathrm{1}}+\mathrm{1}\right)−\Psi\left(\frac{{a}−{m}}{{m}+\mathrm{1}}+\mathrm{1}\right)+\Psi\left(\frac{\mathrm{1}−{a}−\mathrm{2}}{{m}+\mathrm{1}}+\mathrm{1}\right)−\Psi\left(\frac{−{a}−\mathrm{2}}{{m}+\mathrm{1}}+\mathrm{1}\right)\right) \\ $$$${put}\:{a}=\frac{\mathrm{1}}{{n}},{m}=\mathrm{3} \\ $$

Commented by mnjuly1970 last updated on 16/Oct/21