Question Number 148816 by ArielVyny last updated on 31/Jul/21

$$\int_{\mathrm{0}} ^{\infty} {x}^{{m}} {e}^{{ix}^{{n}} } {dx}=?? \\ $$
Answered by mathmax by abdo last updated on 01/Aug/21
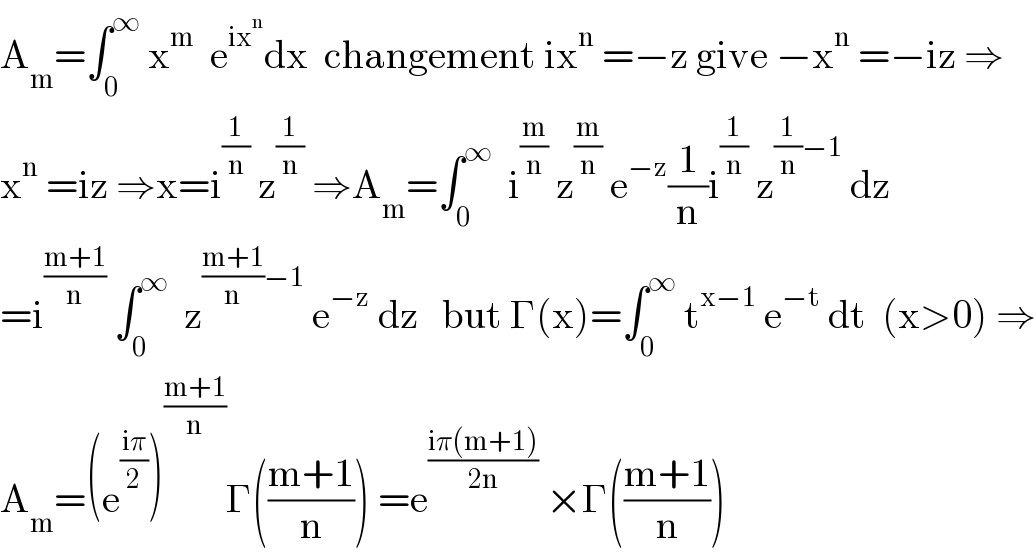
$$\mathrm{A}_{\mathrm{m}} =\int_{\mathrm{0}} ^{\infty} \:\mathrm{x}^{\mathrm{m}} \:\:\mathrm{e}^{\mathrm{ix}^{\mathrm{n}} } \mathrm{dx}\:\:\mathrm{changement}\:\mathrm{ix}^{\mathrm{n}} \:=−\mathrm{z}\:\mathrm{give}\:−\mathrm{x}^{\mathrm{n}} \:=−\mathrm{iz}\:\Rightarrow \\ $$$$\mathrm{x}^{\mathrm{n}} \:=\mathrm{iz}\:\Rightarrow\mathrm{x}=\mathrm{i}^{\frac{\mathrm{1}}{\mathrm{n}}} \:\mathrm{z}^{\frac{\mathrm{1}}{\mathrm{n}}} \:\Rightarrow\mathrm{A}_{\mathrm{m}} =\int_{\mathrm{0}} ^{\infty} \:\:\mathrm{i}^{\frac{\mathrm{m}}{\mathrm{n}}} \:\mathrm{z}^{\frac{\mathrm{m}}{\mathrm{n}}} \:\mathrm{e}^{−\mathrm{z}} \frac{\mathrm{1}}{\mathrm{n}}\mathrm{i}^{\frac{\mathrm{1}}{\mathrm{n}}} \:\mathrm{z}^{\frac{\mathrm{1}}{\mathrm{n}}−\mathrm{1}} \:\mathrm{dz} \\ $$$$=\mathrm{i}^{\frac{\mathrm{m}+\mathrm{1}}{\mathrm{n}}} \:\int_{\mathrm{0}} ^{\infty} \:\:\mathrm{z}^{\frac{\mathrm{m}+\mathrm{1}}{\mathrm{n}}−\mathrm{1}} \:\mathrm{e}^{−\mathrm{z}} \:\mathrm{dz}\:\:\:\mathrm{but}\:\Gamma\left(\mathrm{x}\right)=\int_{\mathrm{0}} ^{\infty} \:\mathrm{t}^{\mathrm{x}−\mathrm{1}} \:\mathrm{e}^{−\mathrm{t}} \:\mathrm{dt}\:\:\left(\mathrm{x}>\mathrm{0}\right)\:\Rightarrow \\ $$$$\mathrm{A}_{\mathrm{m}} =\left(\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{2}}} \right)^{\frac{\mathrm{m}+\mathrm{1}}{\mathrm{n}}} \Gamma\left(\frac{\mathrm{m}+\mathrm{1}}{\mathrm{n}}\right)\:=\mathrm{e}^{\frac{\mathrm{i}\pi\left(\mathrm{m}+\mathrm{1}\right)}{\mathrm{2n}}} \:×\Gamma\left(\frac{\mathrm{m}+\mathrm{1}}{\mathrm{n}}\right) \\ $$
Commented by ArielVyny last updated on 01/Aug/21
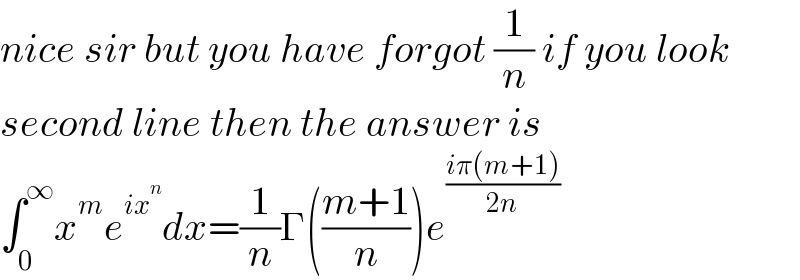
$${nice}\:{sir}\:{but}\:{you}\:{have}\:{forgot}\:\frac{\mathrm{1}}{{n}}\:{if}\:{you}\:{look} \\ $$$${second}\:{line}\:{then}\:{the}\:{answer}\:{is} \\ $$$$\int_{\mathrm{0}} ^{\infty} {x}^{{m}} {e}^{{ix}^{{n}} } {dx}=\frac{\mathrm{1}}{{n}}\Gamma\left(\frac{{m}+\mathrm{1}}{{n}}\right){e}^{\frac{{i}\pi\left({m}+\mathrm{1}\right)}{\mathrm{2}{n}}} \\ $$
