1-0-10pi-sec-1-x-cot-1-x-dx-2-area-bounded-by-curve-y-ln-x-and-the-lines-y-0-y-ln-3-and-x-0-is-equal-to-

Question Number 59474 by rahul 19 last updated on 10/May/19

1) \int_{0}^{10 π} ([\sec^{- 1} x] + [co t^{- 1} x]) d x = ?

2) a r e a b o u n d e d b y c u r v e y = l n (x) a n d

t h e l i n e s y = 0, y = l n (3) a n d x = 0 i s

e q u a l t o ?

Commented by tanmay last updated on 10/May/19

1) f r o m g r a p h i t c l e a e r n o w \dots

[{c o t}^{- 1} x] = 0 w h e n x [0, 10 π]

\int_{0}^{10 π} [{s e c}^{- 1} x] d x + \int_{0}^{10 π} [{c o t}^{- 1} x] d x

\int_{0}^{s e c 1} [{s e c}^{- 1} x] d x + \int_{s e c 1}^{10 π} [{s e c}^{- 1} x] d x

= \int_{0}^{s e c 1} 0 \times d x + \int_{s e c 1}^{10 π} 1 \times d x

= 0 + (10 π - s e c 1)

= 10 π - s e c 1

Commented by tanmay last updated on 10/May/19

Commented by tanmay last updated on 10/May/19

Answered by tanmay last updated on 10/May/19

2) \int_{0}^{l n 3} x d y

y = l n x x = e^{y}

\int_{0}^{l n 3} e^{y} d y \to∣ e^{y} ∣_{0}^{l n 3}

= e^{l n 3} - 1

= 3 - 1 = 2

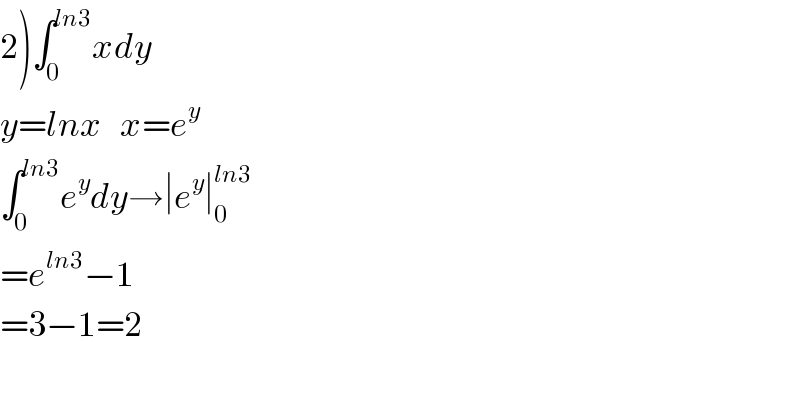
Commented by tanmay last updated on 10/May/19

a n s o f 2 p l s c h e c k

Commented by rahul 19 last updated on 10/May/19

t h a n k s s i r .