1-1-1-2-3-2-2-5-3-2-7-4-2-9-

Question Number 126934 by Dwaipayan Shikari last updated on 25/Dec/20

\frac{1}{1!} + \frac{1!^{2}}{3!} + \frac{2!^{2}}{5!} + \frac{3!^{2}}{7!} + \frac{4!^{2}}{9!} + \dots .

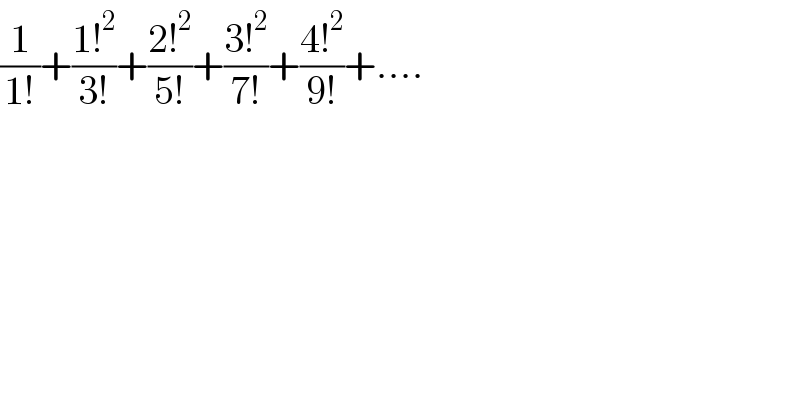
Commented by Dwaipayan Shikari last updated on 25/Dec/20

I h a v e f o u n d \frac{2 π}{3 \sqrt{3}}

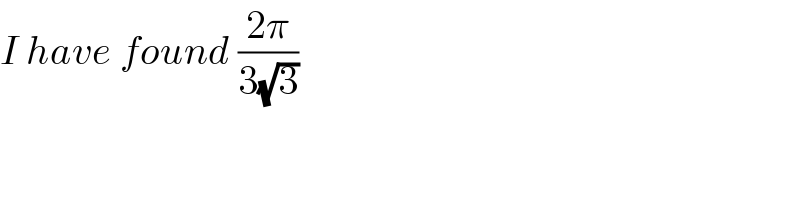
Commented by Olaf last updated on 25/Dec/20

I try to find the solution

with Wallis I_{n} = \int_{0}^{\frac{π}{2}} \sin^{2 n + 1} x d x

\dots to be continued \dots

Commented by Dwaipayan Shikari last updated on 25/Dec/20

I h a v e t r i e d

\underset{n = 0}{\sum^{\infty}} \frac{n!^{2}}{(2 n + 1)!} = \underset{n = 0}{\sum^{\infty}} \frac{Γ^{2} (n + 1)}{Γ (2 n + 2)} = \underset{n = 0}{\sum^{\infty}} B (n + 1, n + 1)

= \underset{n = 0}{\sum^{\infty}} \int_{0}^{1} x^{n} {(1 - x)}^{n} = \int_{0}^{1} \underset{n = 0}{\sum^{\infty}} x^{n} {(1 - x)}^{n} = \int_{0}^{1} \frac{1}{1 - x (1 - x)} d x

= \int_{0}^{1} \frac{1}{x^{2} - x + 1} d x = {[\frac{2}{\sqrt{3}} {t a n}^{- 1} \frac{2 x - 1}{\sqrt{3}}]}_{0}^{1} = \frac{2 π}{3 \sqrt{3}}

Answered by mindispower last updated on 25/Dec/20

= \sum_{n ⩾ 0} \frac{n! . n!}{(2 n + 1)!} = \sum_{n ⩾ 0} \frac{Γ (n + 1) Γ (n + 1)}{Γ {(2 n + 2)}_{}}

= \sum_{n ⩾ 0} β (n + 1, n + 1) = \sum_{n ⩾ 0} \int_{0}^{1} t^{n - 1} {(1 - t)}^{n - 1} d t

= \int_{0}^{1} \sum_{n ⩾ 0} {(t (1 - t))}^{n - 1} d t

= \int_{0}^{1} \frac{1}{1 - t + t^{2}} d t

= \int_{0}^{1} \frac{1}{{(t - \frac{1}{2})}^{2} + \frac{3}{4}} d t

= \int_{0}^{1} \frac{d t}{\frac{3}{4} ({(\frac{2 t - 1}{\sqrt{3}})}^{2} + 1)} = [_{0}^{1} \frac{2}{\sqrt{3}} {t a n}^{-} (\frac{2 t - 1}{\sqrt{3}})]

= \frac{4}{\sqrt{3}} {t a n}^{-} (\frac{1}{\sqrt{3}}) = 4 \frac{π}{6 \sqrt{3}} = \frac{2 π}{3 \sqrt{3}}

Commented by Dwaipayan Shikari last updated on 25/Dec/20

T h a n k i n g y o u