1-1-2-1-2-1-4-1-2-3-4-1-8-1-3-5-2-4-6-

Question Number 124459 by Dwaipayan Shikari last updated on 03/Dec/20

1 - \frac{1}{2} (\frac{1}{2}) + \frac{1}{4} (\frac{1}{2} . \frac{3}{4}) - \frac{1}{8} (\frac{1.3.5}{2.4.6}) + \dots

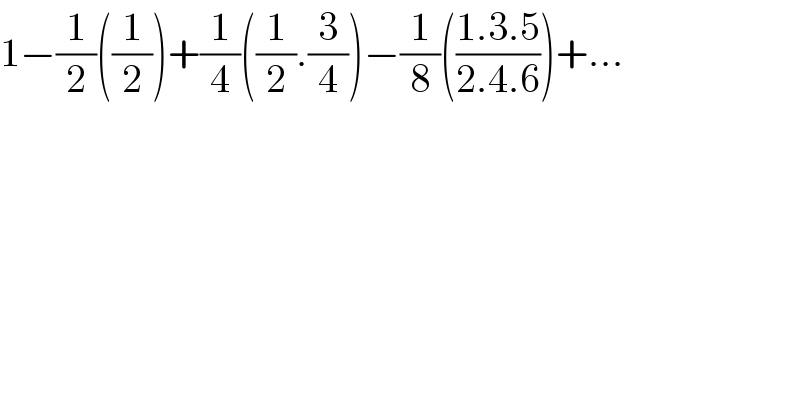
Commented by Dwaipayan Shikari last updated on 03/Dec/20

I h a v e f o u n d \sqrt{\frac{2}{3}}

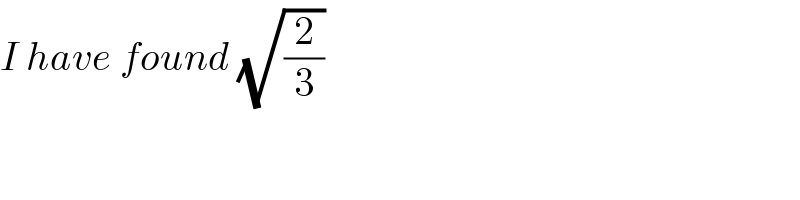
Answered by Olaf last updated on 03/Dec/20

S = 1 + \underset{n = 1}{\sum^{\infty}} {(- 1)}^{n} \frac{1}{2^{n}} (\frac{1 \times 3 \times \dots (2 n - 1)}{2 \times 4 \times \dots (2 n)})

S = 1 + \underset{n = 1}{\sum^{\infty}} {(- 1)}^{n} \frac{1}{2^{n}} (\frac{1 \times 3 \times \dots (2 n - 1)}{2 \times 4 \times \dots (2 n)})

S = 1 + \underset{n = 1}{\sum^{\infty}} \frac{{(- 1)}^{n}}{2^{n} (2 n)!} {(1 \times 3 \times \dots (2 n - 1))}^{2}

S = 1 + \underset{n = 1}{\sum^{\infty}} \frac{{(- 1)}^{n}}{2^{n} (2 n)!} {[\frac{(1 \times (2.1) \times 3 \times (2.2) \times \dots . (2 n - 1) \times (2 n)}{(2.1) \times (2.2) \dots . (2 n)}]}^{2}

S = 1 + \underset{n = 1}{\sum^{\infty}} \frac{{(- 1)}^{n}}{2^{n} (2 n)!} {[\frac{(2 n)!}{(2^{n} n!}]}^{2}

S = 1 + \underset{n = 1}{\sum^{\infty}} \frac{{(- 1)}^{n} (2 n)!}{2^{3 n} n!^{2}}

S = 1 + (\sqrt{\frac{2}{3}} - 1) = \sqrt{\frac{2}{3}}

Commented by mnjuly1970 last updated on 03/Dec/20

v e r y n i c e s i r o l a f

Commented by Dwaipayan Shikari last updated on 03/Dec/20

T h a n k i n g y o u