Menu Close

1-1-2-1-2-2-1-3-2-1-4-2-1-6-2-

Tinku Tara 0 Comments
Pin




Question Number 99853 by Dwaipayan Shikari last updated on 23/Jun/20
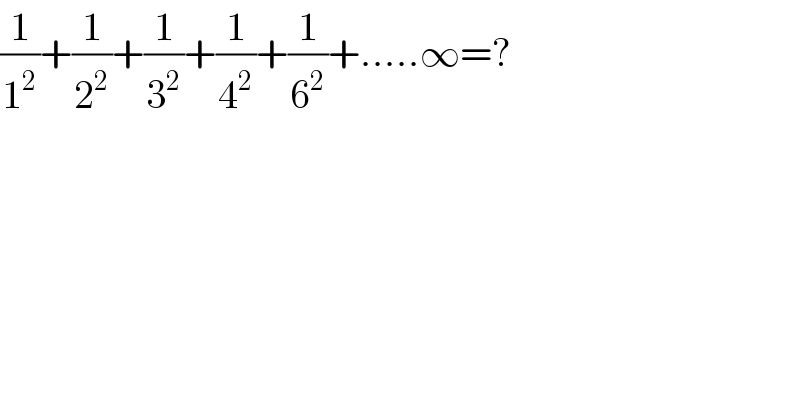
(1/1^2 )+(1/2^2 )+(1/3^2 )+(1/4^2 )+(1/6^2 )+.....∞=?
$$\frac{\mathrm{1}}{\mathrm{1}^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{4}^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{6}^{\mathrm{2}} }+…..\infty=? \\ $$
Answered by smridha last updated on 23/Jun/20
Ξ£_(n=1) ^∞ n^(βˆ’2) =𝛇(2)=(𝛑^2 /6)
$$\underset{\boldsymbol{{n}}=\mathrm{1}} {\overset{\infty} {\sum}}{n}^{βˆ’\mathrm{2}} =\boldsymbol{\zeta}\left(\mathrm{2}\right)=\frac{\boldsymbol{\pi}^{\mathrm{2}} }{\mathrm{6}} \\ $$
Commented by Dwaipayan Shikari last updated on 23/Jun/20
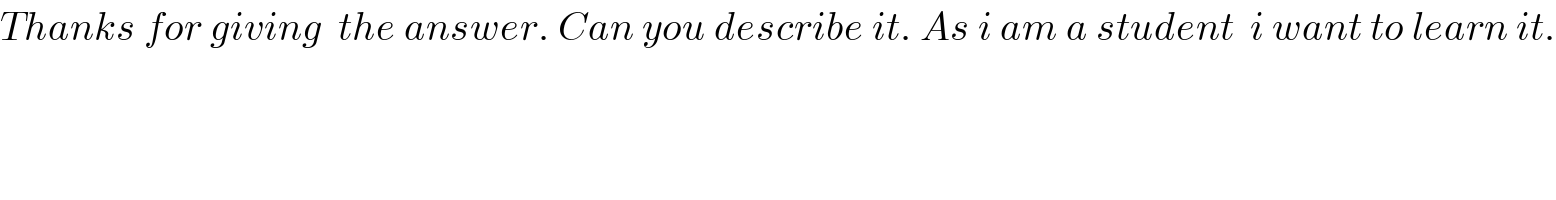
Thanks for giving the answer. Can you describe it. As i am a student i want to learn it.
$${Thanks}\:{for}\:{giving}\:\:{the}\:{answer}.\:{Can}\:{you}\:{describe}\:{it}.\:{As}\:{i}\:{am}\:{a}\:{student}\:\:{i}\:{want}\:{to}\:{learn}\:{it}. \\ $$
Commented by abdomathmax last updated on 23/Jun/20
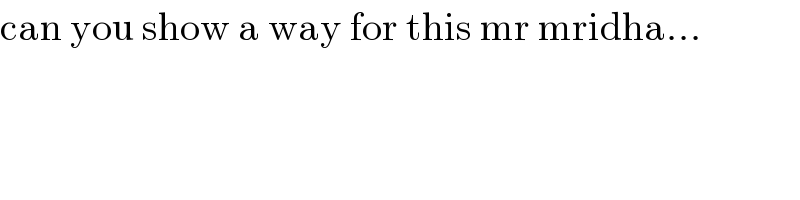
can you show a way for this mr mridha...
$$\mathrm{can}\:\mathrm{you}\:\mathrm{show}\:\mathrm{a}\:\mathrm{way}\:\mathrm{for}\:\mathrm{this}\:\mathrm{mr}\:\mathrm{mridha}… \\ $$
Commented by smridha last updated on 23/Jun/20
okkk i can
$$\boldsymbol{{okkk}}\:\boldsymbol{{i}}\:\boldsymbol{{can}} \\ $$
Commented by smridha last updated on 23/Jun/20
B_(2n) =(βˆ’1)^(nβˆ’1) ((2(2n)!)/((2𝛑)^(2n) ))𝛇(2n) this is the relation bet^n Bernoulli number and Reimann zeta f^n now put n=1 you get.. B_2 =(βˆ’1)^(1βˆ’1) ((2.2!)/((2𝛑)^2 ))𝛇(2) we know B_2 =(1/6) putting it you get 𝛇(2)=(𝛑^2 /6).
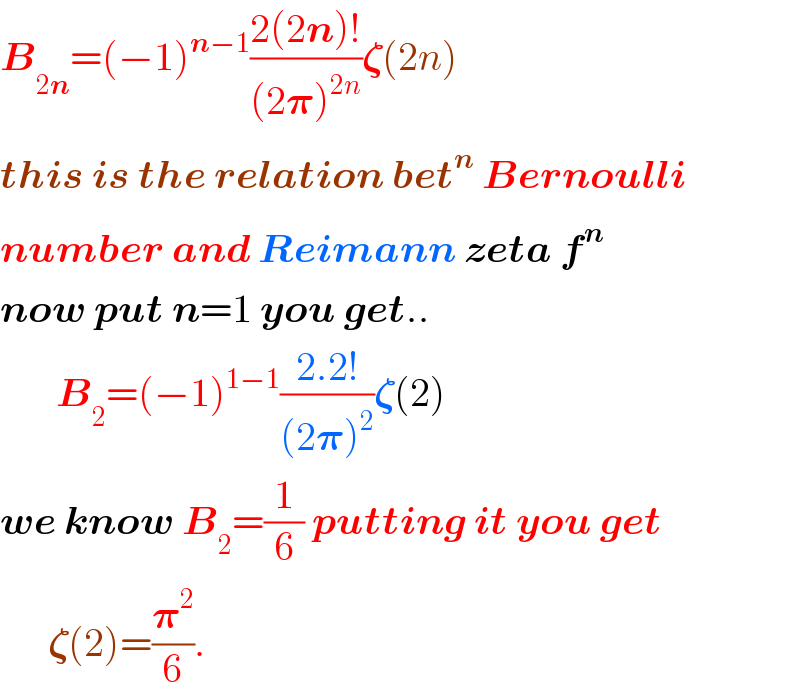
$$\boldsymbol{{B}}_{\mathrm{2}\boldsymbol{{n}}} =\left(βˆ’\mathrm{1}\right)^{\boldsymbol{{n}}βˆ’\mathrm{1}} \frac{\mathrm{2}\left(\mathrm{2}\boldsymbol{{n}}\right)!}{\left(\mathrm{2}\boldsymbol{\pi}\right)^{\mathrm{2}{n}} }\boldsymbol{\zeta}\left(\mathrm{2}{n}\right) \\ $$$$\boldsymbol{{this}}\:\boldsymbol{{is}}\:\boldsymbol{{the}}\:\boldsymbol{{relation}}\:\boldsymbol{{bet}}^{\boldsymbol{{n}}} \:\boldsymbol{{Bernoulli}} \\ $$$$\boldsymbol{{number}}\:\boldsymbol{{and}}\:\boldsymbol{{Reimann}}\:\boldsymbol{{zeta}}\:\boldsymbol{{f}}^{\boldsymbol{{n}}} \\ $$$$\boldsymbol{{now}}\:\boldsymbol{{put}}\:\boldsymbol{{n}}=\mathrm{1}\:\boldsymbol{{you}}\:\boldsymbol{{get}}.. \\ $$$$\:\:\:\:\:\:\:\boldsymbol{{B}}_{\mathrm{2}} =\left(βˆ’\mathrm{1}\right)^{\mathrm{1}βˆ’\mathrm{1}} \frac{\mathrm{2}.\mathrm{2}!}{\left(\mathrm{2}\boldsymbol{\pi}\right)^{\mathrm{2}} }\boldsymbol{\zeta}\left(\mathrm{2}\right) \\ $$$$\boldsymbol{{we}}\:\boldsymbol{{know}}\:\boldsymbol{{B}}_{\mathrm{2}} =\frac{\mathrm{1}}{\mathrm{6}}\:\boldsymbol{{putting}}\:\boldsymbol{{it}}\:\boldsymbol{{you}}\:\boldsymbol{{get}} \\ $$$$\:\:\:\:\:\:\boldsymbol{\zeta}\left(\mathrm{2}\right)=\frac{\boldsymbol{\pi}^{\mathrm{2}} }{\mathrm{6}}. \\ $$
Commented by smridha last updated on 23/Jun/20
another way: sinx=xΞ _(n=1) ^∞ (1βˆ’(x^2 /(n^2 𝛑^2 ))) β‡’((sin(x))/x)=(1βˆ’(x^2 /(1^2 𝛑^2 )))(1βˆ’(x^2 /(2^2 𝛑^2 )))(1βˆ’(x^2 /(3^2 𝛑^2 )))........ .............................. (i) we know:sinx=xβˆ’(x^3 /(3!))+(x^5 /(5!))βˆ’...... so ((sin(x))/x)=1βˆ’(x^2 /(3!))+(x^4 /(5!))βˆ’........ now compare the x^2 terms both sides of the eq^n (i) we will get (1/(1^2 𝛑^2 ))+(1/(2^2 𝛑^2 ))+(1/(3^2 𝛑^2 ))+.....=(1/(3!)) so finally we get: (1/1^2 )+(1/2^2 )+(1/3^2 )+(1/4^2 )+....∞=𝛇(2)=(𝛑^2 /6).
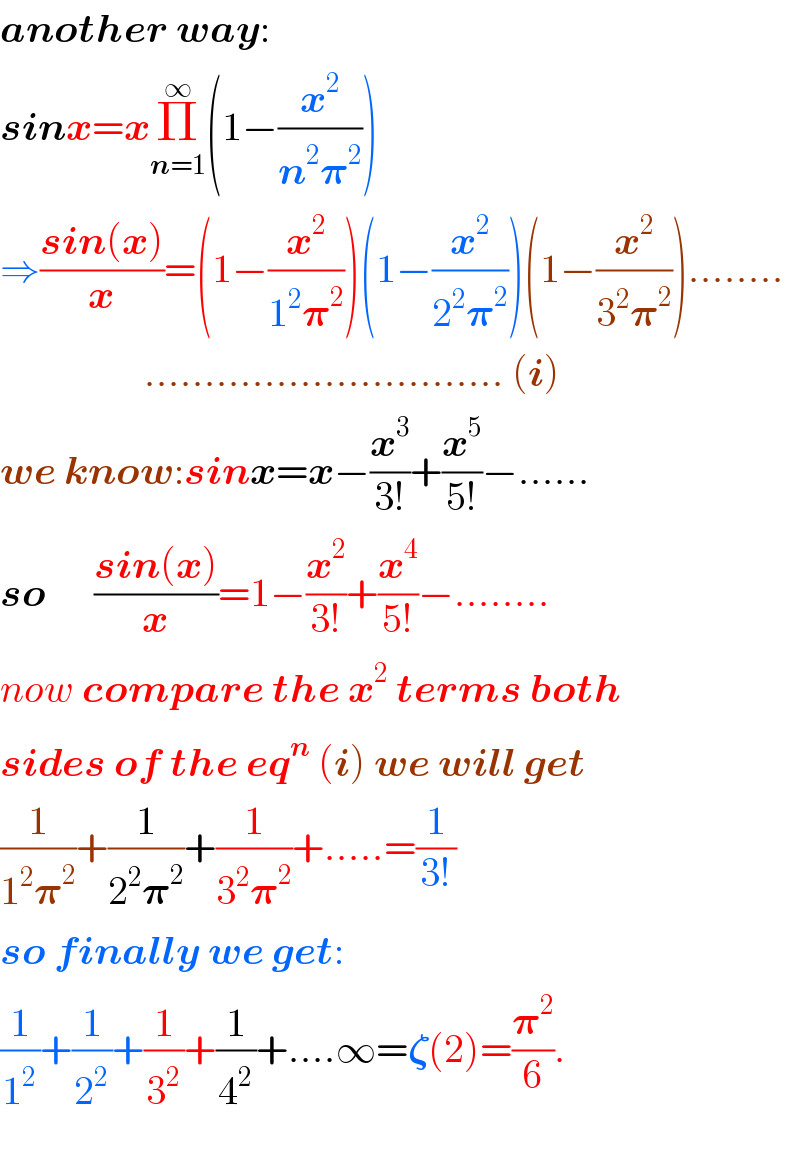
$$\boldsymbol{{another}}\:\boldsymbol{{way}}: \\ $$$$\boldsymbol{{sinx}}=\boldsymbol{{x}}\underset{\boldsymbol{{n}}=\mathrm{1}} {\overset{\infty} {\prod}}\left(\mathrm{1}βˆ’\frac{\boldsymbol{{x}}^{\mathrm{2}} }{\boldsymbol{{n}}^{\mathrm{2}} \boldsymbol{\pi}^{\mathrm{2}} }\right) \\ $$$$\Rightarrow\frac{\boldsymbol{{sin}}\left(\boldsymbol{{x}}\right)}{\boldsymbol{{x}}}=\left(\mathrm{1}βˆ’\frac{\boldsymbol{{x}}^{\mathrm{2}} }{\mathrm{1}^{\mathrm{2}} \boldsymbol{\pi}^{\mathrm{2}} }\right)\left(\mathrm{1}βˆ’\frac{\boldsymbol{{x}}^{\mathrm{2}} }{\mathrm{2}^{\mathrm{2}} \boldsymbol{\pi}^{\mathrm{2}} }\right)\left(\mathrm{1}βˆ’\frac{\boldsymbol{{x}}^{\mathrm{2}} }{\mathrm{3}^{\mathrm{2}} \boldsymbol{\pi}^{\mathrm{2}} }\right)…….. \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:…………………………\:\left(\boldsymbol{{i}}\right) \\ $$$$\boldsymbol{{we}}\:\boldsymbol{{know}}:\boldsymbol{{sinx}}=\boldsymbol{{x}}βˆ’\frac{\boldsymbol{{x}}^{\mathrm{3}} }{\mathrm{3}!}+\frac{\boldsymbol{{x}}^{\mathrm{5}} }{\mathrm{5}!}βˆ’…… \\ $$$$\boldsymbol{{so}}\:\:\:\:\:\:\frac{\boldsymbol{{sin}}\left(\boldsymbol{{x}}\right)}{\boldsymbol{{x}}}=\mathrm{1}βˆ’\frac{\boldsymbol{{x}}^{\mathrm{2}} }{\mathrm{3}!}+\frac{\boldsymbol{{x}}^{\mathrm{4}} }{\mathrm{5}!}βˆ’…….. \\ $$$${now}\:\boldsymbol{{compare}}\:\boldsymbol{{the}}\:\boldsymbol{{x}}^{\mathrm{2}} \:\boldsymbol{{terms}}\:\boldsymbol{{both}} \\ $$$$\boldsymbol{{sides}}\:\boldsymbol{{of}}\:\boldsymbol{{the}}\:\boldsymbol{{eq}}^{\boldsymbol{{n}}} \:\left(\boldsymbol{{i}}\right)\:\boldsymbol{{we}}\:\boldsymbol{{will}}\:\boldsymbol{{get}} \\ $$$$\frac{\mathrm{1}}{\mathrm{1}^{\mathrm{2}} \boldsymbol{\pi}^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{2}} \boldsymbol{\pi}^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{2}} \boldsymbol{\pi}^{\mathrm{2}} }+…..=\frac{\mathrm{1}}{\mathrm{3}!} \\ $$$$\boldsymbol{{so}}\:\boldsymbol{{finally}}\:\boldsymbol{{we}}\:\boldsymbol{{get}}: \\ $$$$\frac{\mathrm{1}}{\mathrm{1}^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{4}^{\mathrm{2}} }+….\infty=\boldsymbol{\zeta}\left(\mathrm{2}\right)=\frac{\boldsymbol{\pi}^{\mathrm{2}} }{\mathrm{6}}. \\ $$$$ \\ $$
Commented by I want to learn more last updated on 23/Jun/20
sir what of ΞΆ(3) ???
$$\mathrm{sir}\:\mathrm{what}\:\mathrm{of}\:\:\zeta\left(\mathrm{3}\right)\:\:??? \\ $$
Commented by abdomathmax last updated on 23/Jun/20
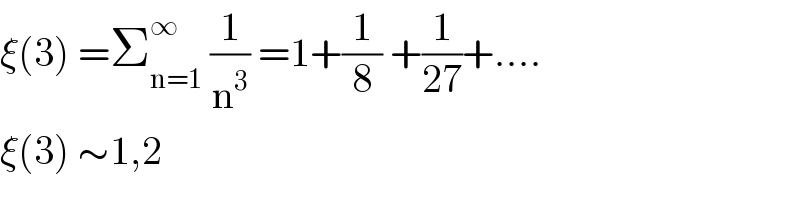
ξ(3) =Σ_(n=1) ^∞ (1/n^3 ) =1+(1/8) +(1/(27))+.... ξ(3) ∼1,2
$$\xi\left(\mathrm{3}\right)\:=\sum_{\mathrm{n}=\mathrm{1}} ^{\infty} \:\frac{\mathrm{1}}{\mathrm{n}^{\mathrm{3}} }\:=\mathrm{1}+\frac{\mathrm{1}}{\mathrm{8}}\:+\frac{\mathrm{1}}{\mathrm{27}}+…. \\ $$$$\xi\left(\mathrm{3}\right)\:\sim\mathrm{1},\mathrm{2} \\ $$
Commented by I want to learn more last updated on 23/Jun/20
Sir, please full workings.
$$\mathrm{Sir},\:\mathrm{please}\:\mathrm{full}\:\mathrm{workings}. \\ $$
Commented by smridha last updated on 23/Jun/20
by Eulerβˆ’ Maclaurin integration formula... 𝛇(3)=(1/2)+(1/2)+Ξ£_(p=1) ^∞ (((2p+1)B_(2p) )/(2x^(2p+2) ))+remaider. 𝛇(3)β‰ˆ1.202057..
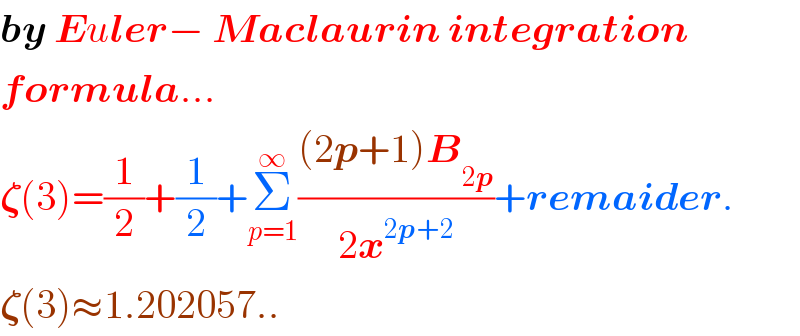
$$\boldsymbol{{by}}\:\boldsymbol{{E}}{u}\boldsymbol{{ler}}βˆ’\:\boldsymbol{{Maclaurin}}\:\boldsymbol{{integration}} \\ $$$$\boldsymbol{{formula}}… \\ $$$$\boldsymbol{\zeta}\left(\mathrm{3}\right)=\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}}+\underset{{p}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(\mathrm{2}\boldsymbol{{p}}+\mathrm{1}\right)\boldsymbol{{B}}_{\mathrm{2}\boldsymbol{{p}}} }{\mathrm{2}\boldsymbol{{x}}^{\mathrm{2}\boldsymbol{{p}}+\mathrm{2}} }+\boldsymbol{{remaider}}. \\ $$$$\boldsymbol{\zeta}\left(\mathrm{3}\right)\approx\mathrm{1}.\mathrm{202057}.. \\ $$
Commented by mathmax by abdo last updated on 23/Jun/20
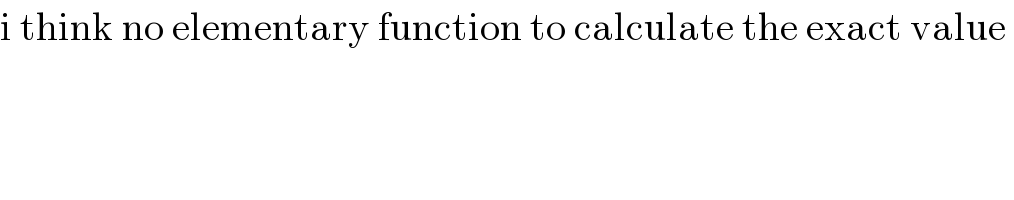
i think no elementary function to calculate the exact value
$$\mathrm{i}\:\mathrm{think}\:\mathrm{no}\:\mathrm{elementary}\:\mathrm{function}\:\mathrm{to}\:\mathrm{calculate}\:\mathrm{the}\:\mathrm{exact}\:\mathrm{value}\: \\ $$
Commented by smridha last updated on 24/Jun/20
therefore I used β‰ˆ instead of =.
$$\boldsymbol{{therefore}}\:\boldsymbol{{I}}\:\boldsymbol{{used}}\:\approx\:{in}\boldsymbol{{stead}}\:\boldsymbol{{of}} \\ $$$$=. \\ $$$$ \\ $$
Answered by abdomathmax last updated on 23/Jun/20
let f(x)=1 2Ο€ periodic odd let developp f at fourier serie f(x) =Ξ£_(n=1) ^∞ a_n sin(nx) a_n =(2/T)∫_([T]) sin(nx)dx =(1/Ο€)∫_(βˆ’Ο€) ^Ο€ sin(nx)dx =(2/Ο€) ∫_0 ^Ο€ sin(nx)dx =βˆ’(2/Ο€)[(1/n)cos(nx)]_0 ^Ο€ =βˆ’(2/(nΟ€)){ (βˆ’1)^n βˆ’1) β‡’ f(x) =Ξ£_(n=1) ^(∞ ) (2/(nΟ€))(1βˆ’(βˆ’1)^n )sin(nx) =(2/Ο€) Ξ£_(n=0) ^∞ (2/(2n+1))sin(2n+1)x =1 β‡’ (4/Ο€) Ξ£_(n=0) ^∞ ((sin(2n+1)x)/((2n+1))) =1 β‡’ Ξ£_(n=0) ^∞ ((sin(2n+1)x)/(2n+1)) =(Ο€/4) let integrate β‡’ βˆ’Ξ£_(n=0) ^∞ ((cos(2n+1)x)/((2n+1)^2 )) =((Ο€x)/4) +c x=0 β‡’βˆ’Ξ£_(n=0) ^∞ (1/((2n+1)^2 )) =c β‡’ ((Ο€x)/4) =βˆ’Ξ£_(n=0) ^∞ ((cos(2n+1)x)/((2n+1)^2 ))+Ξ£_(n=0) ^∞ (1/((2n+1)^2 )) x=Ο€ β‡’(Ο€^2 /4) =Ξ£_(n=0) ^∞ (1/((2n+1)^2 )) +Ξ£_(n=0) ^∞ (1/((2n+1)^2 )) β‡’ Ξ£_(n=0) ^∞ (1/((2n+1)^2 )) =(Ο€^2 /8) we have Ξ£_(n=1) ^∞ (1/n^2 ) =(1/4)Ξ£_(n=1) ^∞ (1/n^2 ) +Ξ£_(n=0) ^∞ (1/((2n+1)^2 )) β‡’(3/4)Ξ£_(n=1) ^∞ (1/n^2 ) =(Ο€^2 /8) β‡’ Ξ£_(n=1) ^∞ (1/n^2 ) =(4/3)Γ—(Ο€^2 /8) Ξ£_(n=1) ^∞ (1/n^2 ) =(Ο€^2 /6)
$$\mathrm{let}\:\mathrm{f}\left(\mathrm{x}\right)=\mathrm{1}\:\:\mathrm{2}\pi\:\mathrm{periodic}\:\mathrm{odd}\:\mathrm{let}\:\mathrm{developp}\:\mathrm{f}\:\mathrm{at}\: \\ $$$$\mathrm{fourier}\:\mathrm{serie}\:\mathrm{f}\left(\mathrm{x}\right)\:=\sum_{\mathrm{n}=\mathrm{1}} ^{\infty} \:\mathrm{a}_{\mathrm{n}} \mathrm{sin}\left(\mathrm{nx}\right) \\ $$$$\mathrm{a}_{\mathrm{n}} =\frac{\mathrm{2}}{\mathrm{T}}\int_{\left[\mathrm{T}\right]} \:\mathrm{sin}\left(\mathrm{nx}\right)\mathrm{dx}\:=\frac{\mathrm{1}}{\pi}\int_{βˆ’\pi} ^{\pi} \:\mathrm{sin}\left(\mathrm{nx}\right)\mathrm{dx} \\ $$$$=\frac{\mathrm{2}}{\pi}\:\int_{\mathrm{0}} ^{\pi} \:\mathrm{sin}\left(\mathrm{nx}\right)\mathrm{dx}\:\:=βˆ’\frac{\mathrm{2}}{\pi}\left[\frac{\mathrm{1}}{\mathrm{n}}\mathrm{cos}\left(\mathrm{nx}\right)\right]_{\mathrm{0}} ^{\pi} \\ $$$$=βˆ’\frac{\mathrm{2}}{\mathrm{n}\pi}\left\{\:\left(βˆ’\mathrm{1}\right)^{\mathrm{n}} βˆ’\mathrm{1}\right)\:\Rightarrow \\ $$$$\mathrm{f}\left(\mathrm{x}\right)\:=\sum_{\mathrm{n}=\mathrm{1}} ^{\infty\:} \:\frac{\mathrm{2}}{\mathrm{n}\pi}\left(\mathrm{1}βˆ’\left(βˆ’\mathrm{1}\right)^{\mathrm{n}} \right)\mathrm{sin}\left(\mathrm{nx}\right) \\ $$$$=\frac{\mathrm{2}}{\pi}\:\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\frac{\mathrm{2}}{\mathrm{2n}+\mathrm{1}}\mathrm{sin}\left(\mathrm{2n}+\mathrm{1}\right)\mathrm{x}\:=\mathrm{1}\:\Rightarrow \\ $$$$\frac{\mathrm{4}}{\pi}\:\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\frac{\mathrm{sin}\left(\mathrm{2n}+\mathrm{1}\right)\mathrm{x}}{\left(\mathrm{2n}+\mathrm{1}\right)}\:=\mathrm{1}\:\Rightarrow \\ $$$$\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\frac{\mathrm{sin}\left(\mathrm{2n}+\mathrm{1}\right)\mathrm{x}}{\mathrm{2n}+\mathrm{1}}\:=\frac{\pi}{\mathrm{4}}\:\:\mathrm{let}\:\mathrm{integrate}\:\Rightarrow \\ $$$$βˆ’\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\frac{\mathrm{cos}\left(\mathrm{2n}+\mathrm{1}\right)\mathrm{x}}{\left(\mathrm{2n}+\mathrm{1}\right)^{\mathrm{2}} }\:=\frac{\pi\mathrm{x}}{\mathrm{4}}\:+\mathrm{c} \\ $$$$\mathrm{x}=\mathrm{0}\:\Rightarrowβˆ’\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\frac{\mathrm{1}}{\left(\mathrm{2n}+\mathrm{1}\right)^{\mathrm{2}} }\:=\mathrm{c}\:\Rightarrow \\ $$$$\frac{\pi\mathrm{x}}{\mathrm{4}}\:=βˆ’\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\frac{\mathrm{cos}\left(\mathrm{2n}+\mathrm{1}\right)\mathrm{x}}{\left(\mathrm{2n}+\mathrm{1}\right)^{\mathrm{2}} }+\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\frac{\mathrm{1}}{\left(\mathrm{2n}+\mathrm{1}\right)^{\mathrm{2}} } \\ $$$$\mathrm{x}=\pi\:\Rightarrow\frac{\pi^{\mathrm{2}} }{\mathrm{4}}\:=\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\frac{\mathrm{1}}{\left(\mathrm{2n}+\mathrm{1}\right)^{\mathrm{2}} }\:+\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\frac{\mathrm{1}}{\left(\mathrm{2n}+\mathrm{1}\right)^{\mathrm{2}} }\:\Rightarrow \\ $$$$\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\frac{\mathrm{1}}{\left(\mathrm{2n}+\mathrm{1}\right)^{\mathrm{2}} }\:=\frac{\pi^{\mathrm{2}} }{\mathrm{8}} \\ $$$$\mathrm{we}\:\mathrm{have}\:\sum_{\mathrm{n}=\mathrm{1}} ^{\infty} \:\frac{\mathrm{1}}{\mathrm{n}^{\mathrm{2}} }\:=\frac{\mathrm{1}}{\mathrm{4}}\sum_{\mathrm{n}=\mathrm{1}} ^{\infty} \:\frac{\mathrm{1}}{\mathrm{n}^{\mathrm{2}} }\:+\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\frac{\mathrm{1}}{\left(\mathrm{2n}+\mathrm{1}\right)^{\mathrm{2}} } \\ $$$$\Rightarrow\frac{\mathrm{3}}{\mathrm{4}}\sum_{\mathrm{n}=\mathrm{1}} ^{\infty} \:\frac{\mathrm{1}}{\mathrm{n}^{\mathrm{2}} }\:=\frac{\pi^{\mathrm{2}} }{\mathrm{8}}\:\Rightarrow\:\sum_{\mathrm{n}=\mathrm{1}} ^{\infty} \:\frac{\mathrm{1}}{\mathrm{n}^{\mathrm{2}} }\:=\frac{\mathrm{4}}{\mathrm{3}}Γ—\frac{\pi^{\mathrm{2}} }{\mathrm{8}} \\ $$$$\sum_{\mathrm{n}=\mathrm{1}} ^{\infty} \:\frac{\mathrm{1}}{\mathrm{n}^{\mathrm{2}} }\:=\frac{\pi^{\mathrm{2}} }{\mathrm{6}} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *