Menu Close

1-1-arctan-x-arctan-x-1-x-2-arctan-1-x-1-x-dx-

Tinku Tara 0 Comments
Pin




Question Number 81290 by mind is power last updated on 11/Feb/20
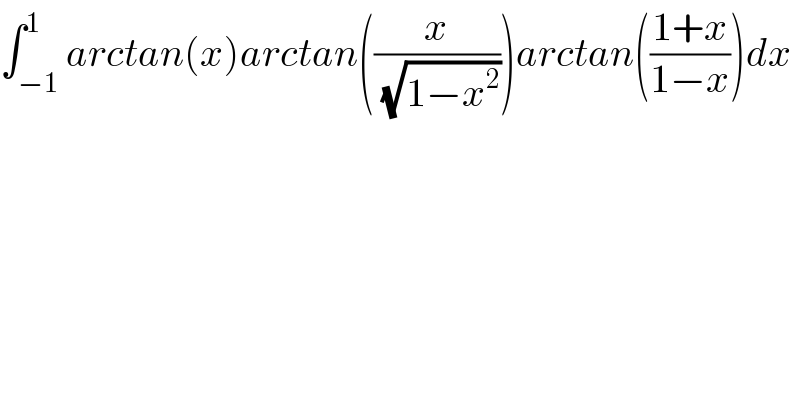
∫_(−1) ^1 arctan(x)arctan((x/( (√(1−x^2 )))))arctan(((1+x)/(1−x)))dx
$$\int_{−\mathrm{1}} ^{\mathrm{1}} {arctan}\left({x}\right){arctan}\left(\frac{{x}}{\:\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }}\right){arctan}\left(\frac{\mathrm{1}+{x}}{\mathrm{1}−{x}}\right){dx} \\ $$
Commented by msup trace by abdo last updated on 11/Feb/20
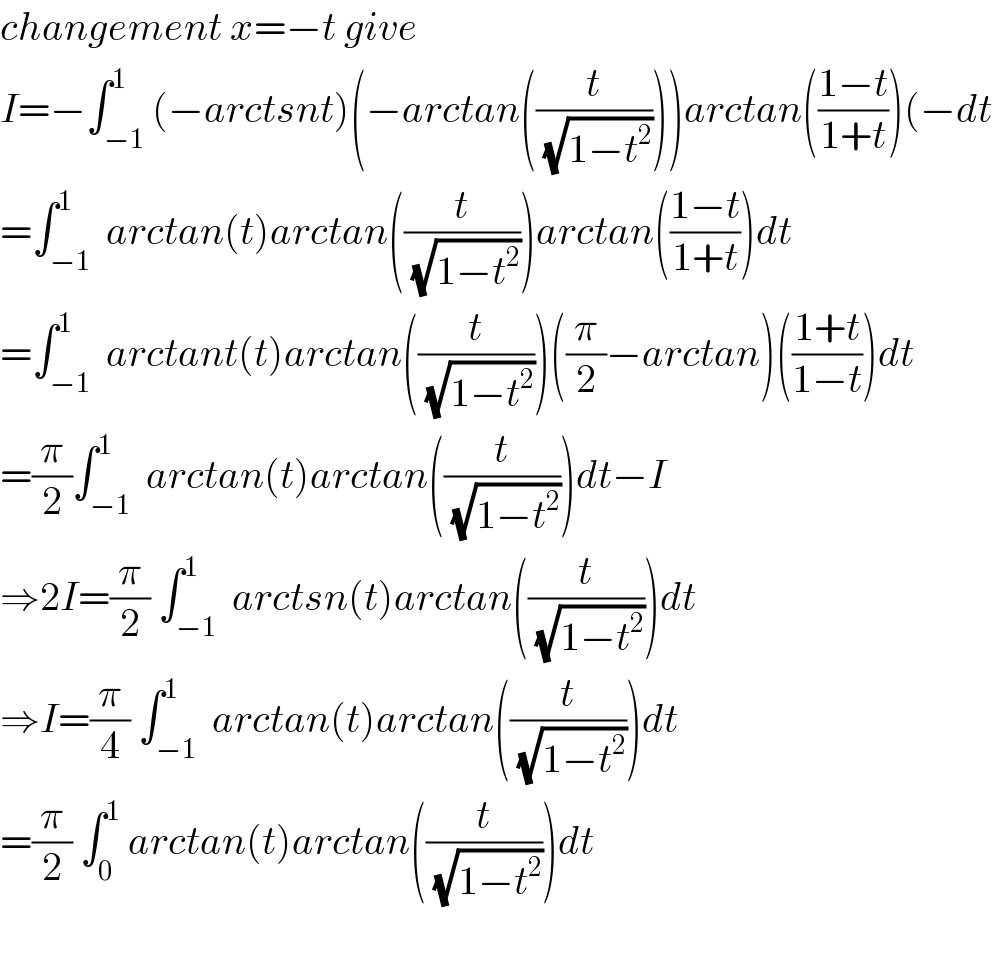
changement x=−t give I=−∫_(−1) ^1 (−arctsnt)(−arctan((t/( (√(1−t^2 ))))))arctan(((1−t)/(1+t)))(−dt =∫_(−1) ^1 arctan(t)arctan((t/( (√(1−t^2 )))))arctan(((1−t)/(1+t)))dt =∫_(−1) ^1 arctant(t)arctan((t/( (√(1−t^2 )))))((π/2)−arctan)(((1+t)/(1−t)))dt =(π/2)∫_(−1) ^1 arctan(t)arctan((t/( (√(1−t^2 )))))dt−I ⇒2I=(π/2) ∫_(−1) ^1 arctsn(t)arctan((t/( (√(1−t^2 )))))dt ⇒I=(π/4) ∫_(−1) ^1 arctan(t)arctan((t/( (√(1−t^2 )))))dt =(π/2) ∫_0 ^1 arctan(t)arctan((t/( (√(1−t^2 )))))dt
$${changement}\:{x}=−{t}\:{give} \\ $$$${I}=−\int_{−\mathrm{1}} ^{\mathrm{1}} \left(−{arctsnt}\right)\left(−{arctan}\left(\frac{{t}}{\:\sqrt{\mathrm{1}−{t}^{\mathrm{2}} }}\right)\right){arctan}\left(\frac{\mathrm{1}−{t}}{\mathrm{1}+{t}}\right)\left(−{dt}\right. \\ $$$$=\int_{−\mathrm{1}} ^{\mathrm{1}} \:{arctan}\left({t}\right){arctan}\left(\frac{{t}}{\:\sqrt{\mathrm{1}−{t}^{\mathrm{2}} }}\right){arctan}\left(\frac{\mathrm{1}−{t}}{\mathrm{1}+{t}}\right){dt} \\ $$$$=\int_{−\mathrm{1}} ^{\mathrm{1}} \:{arctant}\left({t}\right){arctan}\left(\frac{{t}}{\:\sqrt{\mathrm{1}−{t}^{\mathrm{2}} }}\right)\left(\frac{\pi}{\mathrm{2}}−{arctan}\right)\left(\frac{\mathrm{1}+{t}}{\mathrm{1}−{t}}\right){dt} \\ $$$$=\frac{\pi}{\mathrm{2}}\int_{−\mathrm{1}} ^{\mathrm{1}} \:{arctan}\left({t}\right){arctan}\left(\frac{{t}}{\:\sqrt{\mathrm{1}−{t}^{\mathrm{2}} }}\right){dt}−{I} \\ $$$$\Rightarrow\mathrm{2}{I}=\frac{\pi}{\mathrm{2}}\:\int_{−\mathrm{1}} ^{\mathrm{1}} \:{arctsn}\left({t}\right){arctan}\left(\frac{{t}}{\:\sqrt{\mathrm{1}−{t}^{\mathrm{2}} }}\right){dt} \\ $$$$\Rightarrow{I}=\frac{\pi}{\mathrm{4}}\:\int_{−\mathrm{1}} ^{\mathrm{1}} \:{arctan}\left({t}\right){arctan}\left(\frac{{t}}{\:\sqrt{\mathrm{1}−{t}^{\mathrm{2}} }}\right){dt} \\ $$$$=\frac{\pi}{\mathrm{2}}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:{arctan}\left({t}\right){arctan}\left(\frac{{t}}{\:\sqrt{\mathrm{1}−{t}^{\mathrm{2}} }}\right){dt} \\ $$$$ \\ $$
Commented by msup trace by abdo last updated on 11/Feb/20
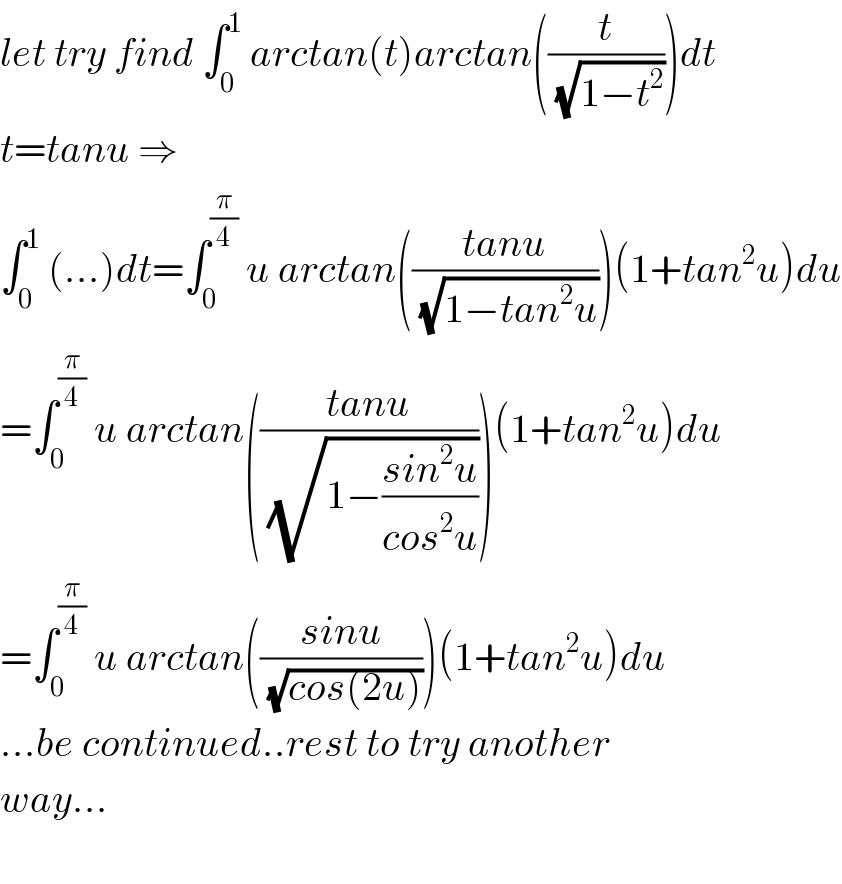
let try find ∫_0 ^1 arctan(t)arctan((t/( (√(1−t^2 )))))dt t=tanu ⇒ ∫_0 ^1 (...)dt=∫_0 ^(π/4) u arctan(((tanu)/( (√(1−tan^2 u)))))(1+tan^2 u)du =∫_0 ^(π/4) u arctan(((tanu)/( (√(1−((sin^2 u)/(cos^2 u)))))))(1+tan^2 u)du =∫_0 ^(π/4) u arctan(((sinu)/( (√(cos(2u))))))(1+tan^2 u)du ...be continued..rest to try another way...
$${let}\:{try}\:{find}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:{arctan}\left({t}\right){arctan}\left(\frac{{t}}{\:\sqrt{\mathrm{1}−{t}^{\mathrm{2}} }}\right){dt} \\ $$$${t}={tanu}\:\Rightarrow \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \:\left(…\right){dt}=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:{u}\:{arctan}\left(\frac{{tanu}}{\:\sqrt{\mathrm{1}−{tan}^{\mathrm{2}} {u}}}\right)\left(\mathrm{1}+{tan}^{\mathrm{2}} {u}\right){du} \\ $$$$=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:{u}\:{arctan}\left(\frac{{tanu}}{\:\sqrt{\mathrm{1}−\frac{{sin}^{\mathrm{2}} {u}}{{cos}^{\mathrm{2}} {u}}}}\right)\left(\mathrm{1}+{tan}^{\mathrm{2}} {u}\right){du} \\ $$$$=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:{u}\:{arctan}\left(\frac{{sinu}}{\:\sqrt{{cos}\left(\mathrm{2}{u}\right)}}\right)\left(\mathrm{1}+{tan}^{\mathrm{2}} {u}\right){du} \\ $$$$…{be}\:{continued}..{rest}\:{to}\:{try}\:{another} \\ $$$${way}… \\ $$$$ \\ $$
Commented by mind is power last updated on 11/Feb/20
try withe t=sin(α)
$${try}\:{withe}\:{t}={sin}\left(\alpha\right) \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *