1-1-cos-i-sin-1-2-i-2-cot-2-prove-

Question Number 129404 by bemath last updated on 15/Jan/21

\frac{1}{1 - \cos θ - i \sin θ} = \frac{1}{2} + \frac{i}{2} . \cot (\frac{θ}{2})

prove .

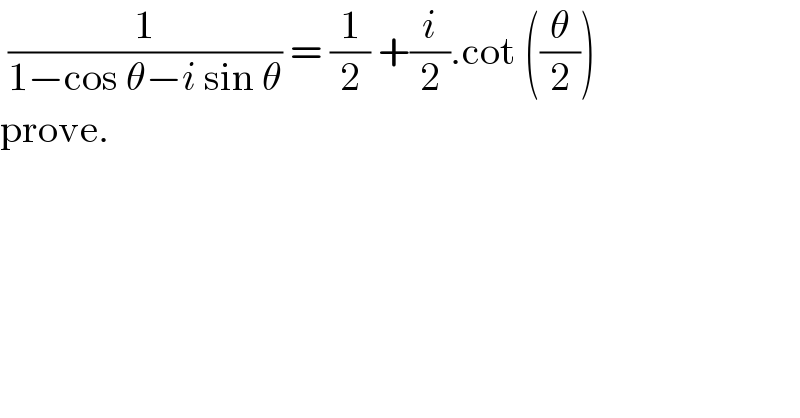
Answered by MJS_new last updated on 15/Jan/21

θ = 2 α

{(1 - \cos 2 α - i \sin 2 α)}^{- 1} =

= {(1 - \frac{1 - \tan^{2} α}{1 + \tan^{2} α} - \frac{2 \tan α}{1 + \tan^{2} α} i)}^{- 1} =

= {(\frac{2 t^{2} - 2 i t}{t^{2} + 1})}^{- 1} = \frac{t^{2} + 1}{2 t^{2} - 2 i t} = \frac{t}{2 t} + \frac{i}{2 t} =

= \frac{1}{2} + \frac{i}{2} \cot α = \frac{1}{2} + \frac{i}{2} \cot \frac{θ}{2}

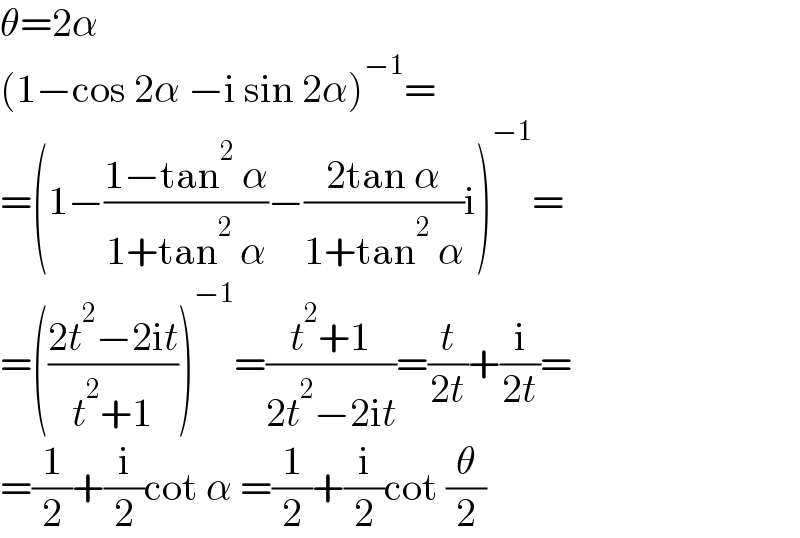
Commented by MJS_new last updated on 16/Jan/21

yes

Commented by liberty last updated on 16/Jan/21

t = \tan α ?

Answered by physicstutes last updated on 15/Jan/21

(\frac{1}{1 - \cos θ - i \sin θ}) (\frac{1 - \cos θ + i \sin θ}{1 - \cos θ + i \sin θ}) = \frac{1 - \cos θ + i \sin θ}{{(1 - \cos θ)}^{2} + {(\sin θ)}^{2}}

= \frac{1 - \cos θ + i \sin θ}{1 - 2 \cos θ + \cos^{2} θ + \sin^{2} θ} = \frac{1 - \cos θ + i \sin θ}{2 - 2 \cos θ} = \frac{1}{2} [\frac{1 - (1 - {2 \sin}^{2} \frac{θ}{2}) + i 2 \sin \frac{θ}{2} \cos \frac{θ}{2}}{1 - (1 - {2 \sin}^{2} \frac{θ}{2})}]

= \frac{1}{2} [1 + i \cot \frac{θ}{2}] = \frac{1}{2} + \frac{i}{2} \cot (\frac{θ}{2})