Question Number 79186 by john santu last updated on 23/Jan/20

$$\underset{−\mathrm{1}} {\overset{\mathrm{1}} {\int}}\:\frac{\mathrm{cos}\:\left({x}\right)}{\mathrm{1}+{e}^{\frac{\mathrm{1}}{{x}}} }\:{dx}\:? \\ $$
Answered by mind is power last updated on 23/Jan/20
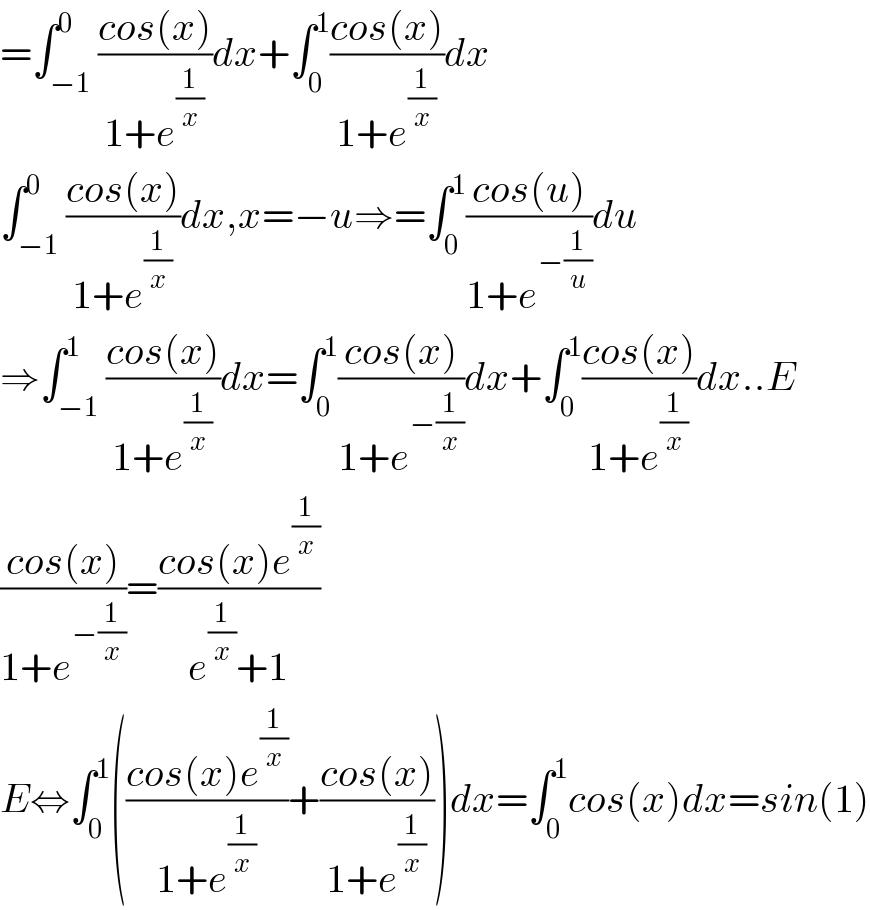
$$=\int_{−\mathrm{1}} ^{\mathrm{0}} \frac{{cos}\left({x}\right)}{\mathrm{1}+{e}^{\frac{\mathrm{1}}{{x}}} }{dx}+\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{cos}\left({x}\right)}{\mathrm{1}+{e}^{\frac{\mathrm{1}}{{x}}} }{dx} \\ $$$$\int_{−\mathrm{1}} ^{\mathrm{0}} \frac{{cos}\left({x}\right)}{\mathrm{1}+{e}^{\frac{\mathrm{1}}{{x}}} }{dx},{x}=−{u}\Rightarrow=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{cos}\left({u}\right)}{\mathrm{1}+{e}^{−\frac{\mathrm{1}}{{u}}} }{du} \\ $$$$\Rightarrow\int_{−\mathrm{1}} ^{\mathrm{1}} \frac{{cos}\left({x}\right)}{\mathrm{1}+{e}^{\frac{\mathrm{1}}{{x}}} }{dx}=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{cos}\left({x}\right)}{\mathrm{1}+{e}^{−\frac{\mathrm{1}}{{x}}} }{dx}+\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{cos}\left({x}\right)}{\mathrm{1}+{e}^{\frac{\mathrm{1}}{{x}}} }{dx}..{E} \\ $$$$\frac{{cos}\left({x}\right)}{\mathrm{1}+{e}^{−\frac{\mathrm{1}}{{x}}} }=\frac{{cos}\left({x}\right){e}^{\frac{\mathrm{1}}{{x}}} }{{e}^{\frac{\mathrm{1}}{{x}}} +\mathrm{1}} \\ $$$${E}\Leftrightarrow\int_{\mathrm{0}} ^{\mathrm{1}} \left(\frac{{cos}\left({x}\right){e}^{\frac{\mathrm{1}}{{x}}} }{\mathrm{1}+{e}^{\frac{\mathrm{1}}{{x}}} }+\frac{{cos}\left({x}\right)}{\mathrm{1}+{e}^{\frac{\mathrm{1}}{{x}}} }\right){dx}=\int_{\mathrm{0}} ^{\mathrm{1}} {cos}\left({x}\right){dx}={sin}\left(\mathrm{1}\right) \\ $$
Commented by john santu last updated on 23/Jan/20

$${thank}\:{you}\:{sir} \\ $$
Commented by mind is power last updated on 23/Jan/20

$${y}'{re}\:{welcom} \\ $$
