Question Number 163408 by mathlove last updated on 06/Jan/22
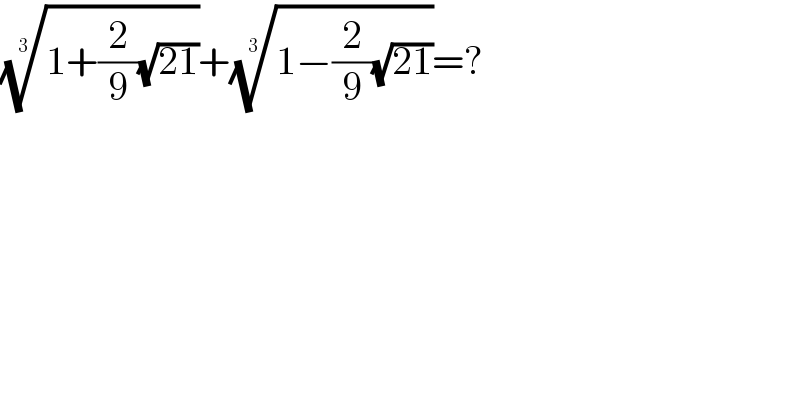
$$\sqrt[{\mathrm{3}}]{\mathrm{1}+\frac{\mathrm{2}}{\mathrm{9}}\sqrt{\mathrm{21}}}+\sqrt[{\mathrm{3}}]{\mathrm{1}−\frac{\mathrm{2}}{\mathrm{9}}\sqrt{\mathrm{21}}}=? \\ $$
Answered by mr W last updated on 06/Jan/22
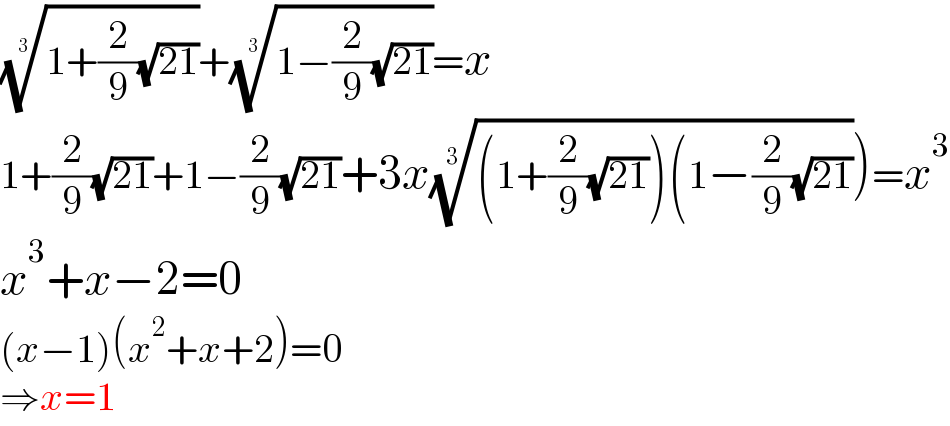
$$\sqrt[{\mathrm{3}}]{\mathrm{1}+\frac{\mathrm{2}}{\mathrm{9}}\sqrt{\mathrm{21}}}+\sqrt[{\mathrm{3}}]{\mathrm{1}−\frac{\mathrm{2}}{\mathrm{9}}\sqrt{\mathrm{21}}}={x} \\ $$$$\left.\mathrm{1}+\frac{\mathrm{2}}{\mathrm{9}}\sqrt{\mathrm{21}}+\mathrm{1}−\frac{\mathrm{2}}{\mathrm{9}}\sqrt{\mathrm{21}}+\mathrm{3}{x}\sqrt[{\mathrm{3}}]{\left(\mathrm{1}+\frac{\mathrm{2}}{\mathrm{9}}\sqrt{\mathrm{21}}\right)\left(\mathrm{1}−\frac{\mathrm{2}}{\mathrm{9}}\sqrt{\mathrm{21}}\right.}\right)={x}^{\mathrm{3}} \\ $$$${x}^{\mathrm{3}} +{x}−\mathrm{2}=\mathrm{0} \\ $$$$\left({x}−\mathrm{1}\right)\left({x}^{\mathrm{2}} +{x}+\mathrm{2}\right)=\mathrm{0} \\ $$$$\Rightarrow{x}=\mathrm{1} \\ $$
