Question Number 130748 by Dwaipayan Shikari last updated on 28/Jan/21
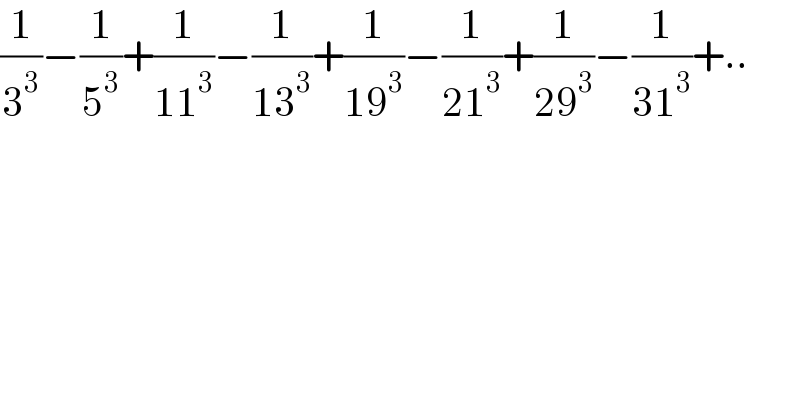
$$\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{3}} }−\frac{\mathrm{1}}{\mathrm{5}^{\mathrm{3}} }+\frac{\mathrm{1}}{\mathrm{11}^{\mathrm{3}} }−\frac{\mathrm{1}}{\mathrm{13}^{\mathrm{3}} }+\frac{\mathrm{1}}{\mathrm{19}^{\mathrm{3}} }−\frac{\mathrm{1}}{\mathrm{21}^{\mathrm{3}} }+\frac{\mathrm{1}}{\mathrm{29}^{\mathrm{3}} }−\frac{\mathrm{1}}{\mathrm{31}^{\mathrm{3}} }+.. \\ $$
Answered by mindispower last updated on 28/Jan/21
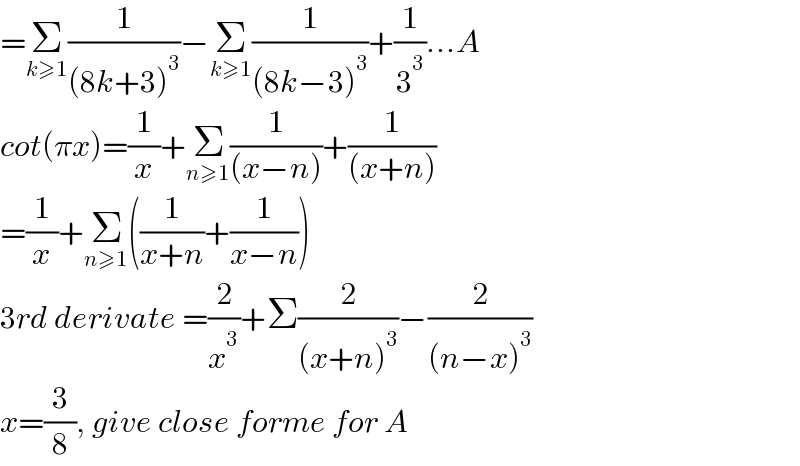
$$=\underset{{k}\geqslant\mathrm{1}} {\sum}\frac{\mathrm{1}}{\left(\mathrm{8}{k}+\mathrm{3}\right)^{\mathrm{3}} }−\underset{{k}\geqslant\mathrm{1}} {\sum}\frac{\mathrm{1}}{\left(\mathrm{8}{k}−\mathrm{3}\right)^{\mathrm{3}} }+\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{3}} }…{A} \\ $$$${cot}\left(\pi{x}\right)=\frac{\mathrm{1}}{{x}}+\underset{{n}\geqslant\mathrm{1}} {\sum}\frac{\mathrm{1}}{\left({x}−{n}\right)}+\frac{\mathrm{1}}{\left({x}+{n}\right)} \\ $$$$=\frac{\mathrm{1}}{{x}}+\underset{{n}\geqslant\mathrm{1}} {\sum}\left(\frac{\mathrm{1}}{{x}+{n}}+\frac{\mathrm{1}}{{x}−{n}}\right) \\ $$$$\mathrm{3}{rd}\:{derivate}\:=\frac{\mathrm{2}}{{x}^{\mathrm{3}} }+\Sigma\frac{\mathrm{2}}{\left({x}+{n}\right)^{\mathrm{3}} }−\frac{\mathrm{2}}{\left({n}−{x}\right)^{\mathrm{3}} } \\ $$$${x}=\frac{\mathrm{3}}{\mathrm{8}},\:{give}\:{close}\:{forme}\:{for}\:{A} \\ $$
