Question Number 155420 by amin96 last updated on 30/Sep/21
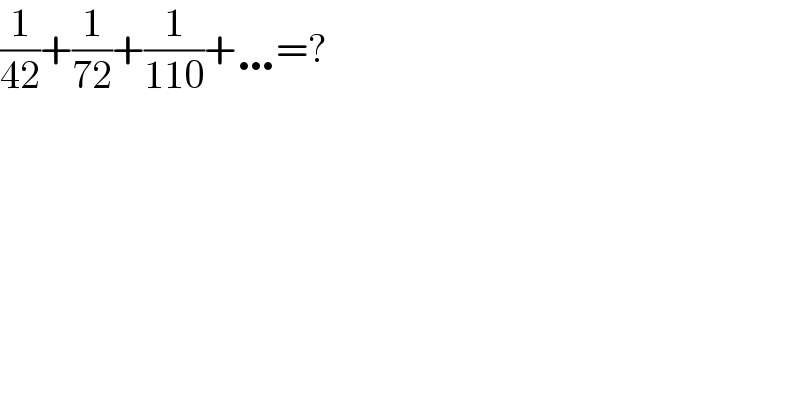
$$\frac{\mathrm{1}}{\mathrm{42}}+\frac{\mathrm{1}}{\mathrm{72}}+\frac{\mathrm{1}}{\mathrm{110}}+\ldots=? \\ $$
Answered by puissant last updated on 01/Oct/21

$${S}=\frac{\mathrm{1}}{\mathrm{42}}+\frac{\mathrm{1}}{\mathrm{72}}+\frac{\mathrm{1}}{\mathrm{110}}+…….=\frac{\mathrm{1}}{\mathrm{6}}−\frac{\mathrm{1}}{\mathrm{7}}+\frac{\mathrm{1}}{\mathrm{8}}−\frac{\mathrm{1}}{\mathrm{9}}+…. \\ $$$$\Rightarrow\:{S}=\underset{{n}=\mathrm{2}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{\left(\mathrm{2}{n}+\mathrm{2}\right)\left(\mathrm{2}{n}+\mathrm{3}\right)}=\frac{\mathrm{1}}{\mathrm{4}}\underset{{n}=\mathrm{2}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{\left({n}+\mathrm{1}\right)\left({n}+\frac{\mathrm{3}}{\mathrm{2}}\right)} \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}}\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{\left({n}+\mathrm{1}\right)\left({n}+\frac{\mathrm{3}}{\mathrm{2}}\right)}−\frac{\mathrm{1}}{\mathrm{20}}−\frac{\mathrm{1}}{\mathrm{6}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}}\bullet\frac{\psi\left(\frac{\mathrm{3}}{\mathrm{2}}\right)−\psi\left(\mathrm{1}\right)}{\frac{\mathrm{3}}{\mathrm{2}}−\mathrm{1}}−\frac{\mathrm{13}}{\mathrm{60}}\:=\:\frac{\mathrm{1}}{\mathrm{2}}\left(\psi\left(\frac{\mathrm{3}}{\mathrm{2}}\right)−\psi\left(\mathrm{1}\right)\right)−\frac{\mathrm{13}}{\mathrm{60}} \\ $$$$\psi\left(\frac{\mathrm{3}}{\mathrm{2}}\right)=\psi\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}}\right)=\psi\left(\frac{\mathrm{1}}{\mathrm{2}}\right)+\mathrm{2}\:; \\ $$$$\:\psi\left(\frac{\mathrm{1}}{\mathrm{2}}\right)=−\mathrm{2}{ln}\mathrm{2}−\gamma\:\:;\:\:\psi\left(\mathrm{1}\right)=−\gamma.. \\ $$$$\Rightarrow\:{S}=\frac{\mathrm{1}}{\mathrm{2}}\left(−\mathrm{2}{ln}\mathrm{2}−\gamma+\mathrm{2}+\gamma\right)−\frac{\mathrm{13}}{\mathrm{60}} \\ $$$$\Rightarrow\:{S}=\:−{ln}\mathrm{2}\:+\mathrm{1}\:−\frac{\mathrm{13}}{\mathrm{60}}.. \\ $$$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\therefore\:\because\:\:\:\:\:\:{S}\:\:=\:\:\frac{\mathrm{47}}{\mathrm{60}}−{ln}\mathrm{2}… \\ $$$$ \\ $$$$\:\:\:\:\:\:\:\:………………..\mathscr{L}{e}\:{puissant}……………… \\ $$
Commented by amin96 last updated on 30/Sep/21

$${great}\:{sir} \\ $$
Commented by Tawa11 last updated on 30/Sep/21

$$\mathrm{Nice}\:\mathrm{sir} \\ $$
Commented by puissant last updated on 30/Sep/21
��������������
Commented by SANOGO last updated on 30/Sep/21

$${le}\:{puissant} \\ $$
