1-a-bi-x-dx-

Question Number 56169 by MJS last updated on 11/Mar/19

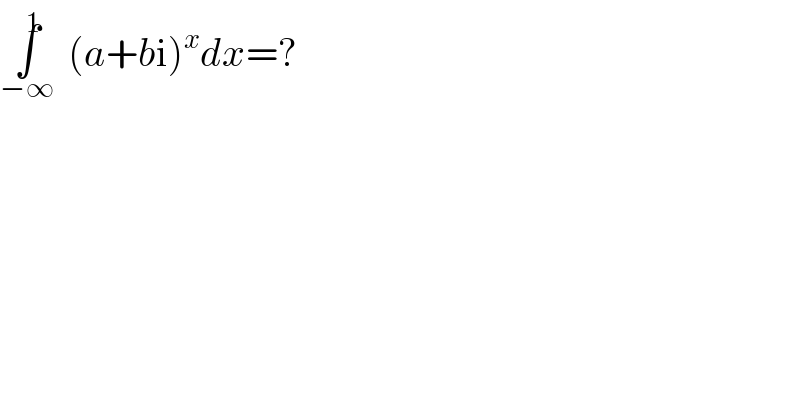
{\int_{- \infty}}^{1} {(a + b i)}^{x} d x = ?

Answered by tanmay.chaudhury50@gmail.com last updated on 11/Mar/19

\int p^{x} d x = \frac{p^{x}}{l n p} + c

b u t {l o g}_{e} (a + i b) = \frac{1}{2} l o g (a^{2} + b^{2}) + i (2 n π + {t a n}^{- 1} \frac{b}{a})

s o

∣ {(a + i b)}^{x} ∣_{- \infty}^{1} / [\frac{1}{2} {l o g}_{e} (a^{2} + b^{2}) + i (2 n π + {t a n}^{- 1} \frac{b}{a})]

(a + i b) / [\frac{1}{2 l} {l o g}_{e} (a^{2} + b^{2}) + i (2 n π + {t a n}^{- 1} \frac{b}{a})

i h a v e r e c t i f i e d \dots

Commented by Smail last updated on 11/Mar/19

\int p^{x} d x = \int e^{x l n p} d x = \frac{1}{l n p} e^{x l n p} + c

= \frac{p^{x}}{l n p} + c

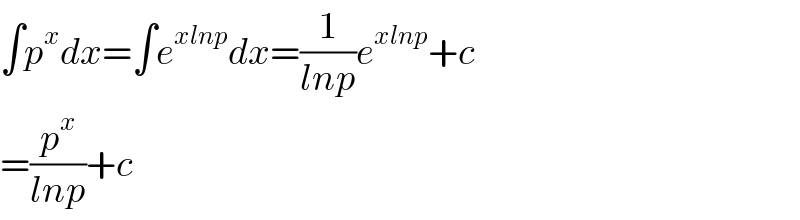
Commented by tanmay.chaudhury50@gmail.com last updated on 11/Mar/19

y e s s i r y o u a r e r i g h t \dots

Commented by MJS last updated on 11/Mar/19

thank you

Facebook Tweet Pin