Menu Close

1-calculate-I-n-0-x-n-e-1-i-x-dx-with-n-integr-natural-and-i-2-1-2-find-0-x-4k-3-xsinx-dx-

Tinku Tara 0 Comments
Pin




Question Number 46612 by maxmathsup by imad last updated on 29/Oct/18
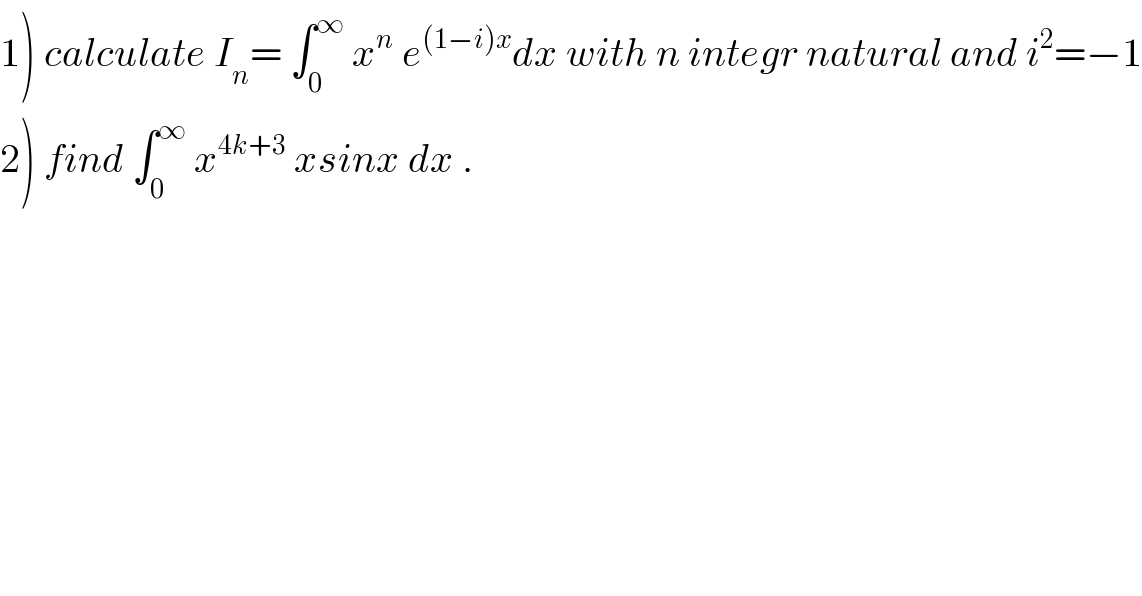
1) calculate I_n = ∫_0 ^∞ x^n e^((1−i)x) dx with n integr natural and i^2 =−1 2) find ∫_0 ^∞ x^(4k+3) xsinx dx .
$$\left.\mathrm{1}\right)\:{calculate}\:{I}_{{n}} =\:\int_{\mathrm{0}} ^{\infty} \:{x}^{{n}} \:{e}^{\left(\mathrm{1}−{i}\right){x}} {dx}\:{with}\:{n}\:{integr}\:{natural}\:{and}\:{i}^{\mathrm{2}} =−\mathrm{1} \\ $$$$\left.\mathrm{2}\right)\:{find}\:\int_{\mathrm{0}} ^{\infty} \:{x}^{\mathrm{4}{k}+\mathrm{3}} \:{xsinx}\:{dx}\:. \\ $$
Commented by maxmathsup by imad last updated on 29/Oct/18
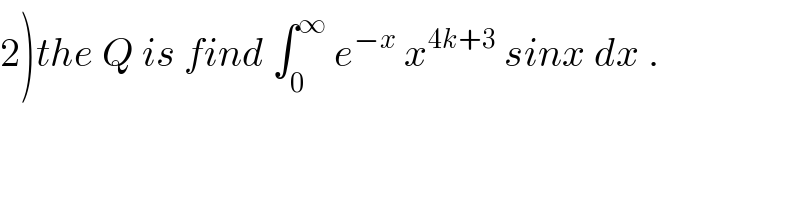
2)the Q is find ∫_0 ^∞ e^(−x) x^(4k+3) sinx dx .
$$\left.\mathrm{2}\right){the}\:{Q}\:{is}\:{find}\:\int_{\mathrm{0}} ^{\infty} \:{e}^{−{x}} \:{x}^{\mathrm{4}{k}+\mathrm{3}} \:{sinx}\:{dx}\:. \\ $$
Commented by maxmathsup by imad last updated on 29/Oct/18
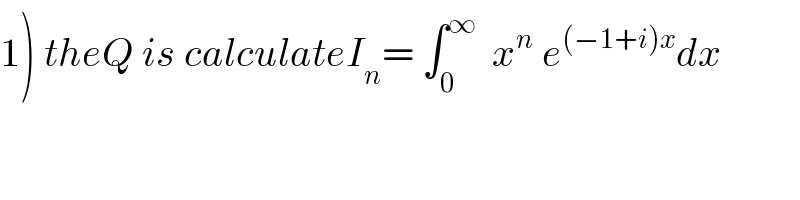
1) theQ is calculateI_n = ∫_0 ^∞ x^n e^((−1+i)x) dx
$$\left.\mathrm{1}\right)\:{theQ}\:{is}\:{calculateI}_{{n}} =\:\int_{\mathrm{0}} ^{\infty} \:\:{x}^{{n}} \:{e}^{\left(−\mathrm{1}+{i}\right){x}} {dx}\: \\ $$
Commented by maxmathsup by imad last updated on 29/Oct/18
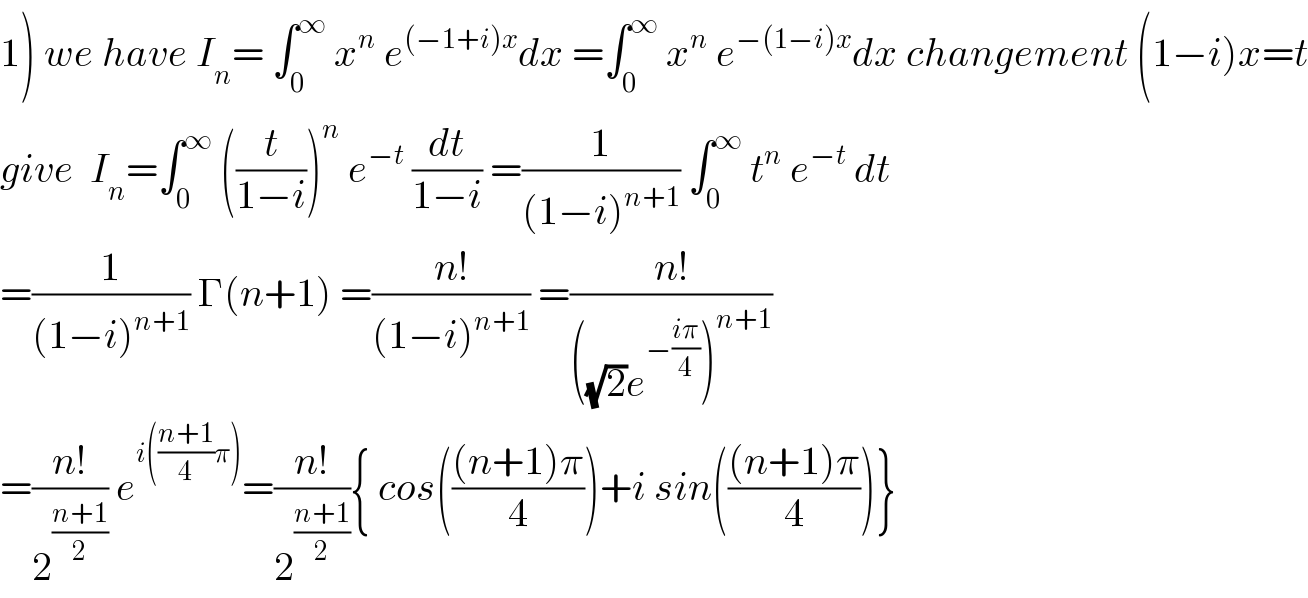
1) we have I_n = ∫_0 ^∞ x^n e^((−1+i)x) dx =∫_0 ^∞ x^n e^(−(1−i)x) dx changement (1−i)x=t give I_n =∫_0 ^∞ ((t/(1−i)))^n e^(−t) (dt/(1−i)) =(1/((1−i)^(n+1) )) ∫_0 ^∞ t^n e^(−t) dt =(1/((1−i)^(n+1) )) Γ(n+1) =((n!)/((1−i)^(n+1) )) =((n!)/(((√2)e^(−((iπ)/4)) )^(n+1) )) =((n!)/2^((n+1)/2) ) e^(i(((n+1)/4)π)) =((n!)/2^((n+1)/2) ){ cos((((n+1)π)/4))+i sin((((n+1)π)/4))}
$$\left.\mathrm{1}\right)\:{we}\:{have}\:{I}_{{n}} =\:\int_{\mathrm{0}} ^{\infty} \:{x}^{{n}} \:{e}^{\left(−\mathrm{1}+{i}\right){x}} {dx}\:=\int_{\mathrm{0}} ^{\infty} \:{x}^{{n}} \:{e}^{−\left(\mathrm{1}−{i}\right){x}} {dx}\:{changement}\:\left(\mathrm{1}−{i}\right){x}={t} \\ $$$${give}\:\:{I}_{{n}} =\int_{\mathrm{0}} ^{\infty} \:\left(\frac{{t}}{\mathrm{1}−{i}}\right)^{{n}} \:{e}^{−{t}} \:\frac{{dt}}{\mathrm{1}−{i}}\:=\frac{\mathrm{1}}{\left(\mathrm{1}−{i}\right)^{{n}+\mathrm{1}} }\:\int_{\mathrm{0}} ^{\infty} \:{t}^{{n}} \:{e}^{−{t}} \:{dt} \\ $$$$=\frac{\mathrm{1}}{\left(\mathrm{1}−{i}\right)^{{n}+\mathrm{1}} }\:\Gamma\left({n}+\mathrm{1}\right)\:=\frac{{n}!}{\left(\mathrm{1}−{i}\right)^{{n}+\mathrm{1}} }\:=\frac{{n}!}{\left(\sqrt{\mathrm{2}}{e}^{−\frac{{i}\pi}{\mathrm{4}}} \right)^{{n}+\mathrm{1}} } \\ $$$$=\frac{{n}!}{\mathrm{2}^{\frac{{n}+\mathrm{1}}{\mathrm{2}}} }\:{e}^{{i}\left(\frac{{n}+\mathrm{1}}{\mathrm{4}}\pi\right)} =\frac{{n}!}{\mathrm{2}^{\frac{{n}+\mathrm{1}}{\mathrm{2}}} }\left\{\:{cos}\left(\frac{\left({n}+\mathrm{1}\right)\pi}{\mathrm{4}}\right)+{i}\:{sin}\left(\frac{\left({n}+\mathrm{1}\right)\pi}{\mathrm{4}}\right)\right\} \\ $$
Commented by maxmathsup by imad last updated on 29/Oct/18
2) we have ∫_0 ^∞ e^(−x) x^(4k+3) sinx dx =Im(∫_0 ^∞ x^(4k+3) e^((−1+i)x) dx) =Im( I_(4k+3) ) =(((4k+3)!)/2^(2k+2) ) sin((((4k+4)π)/4)) =(((4k+3)!)/2^(2k+2) )sin((k+1)π) =0
$$\left.\mathrm{2}\right)\:{we}\:{have}\:\int_{\mathrm{0}} ^{\infty} \:{e}^{−{x}} \:{x}^{\mathrm{4}{k}+\mathrm{3}} \:{sinx}\:{dx}\:={Im}\left(\int_{\mathrm{0}} ^{\infty} \:{x}^{\mathrm{4}{k}+\mathrm{3}} {e}^{\left(−\mathrm{1}+{i}\right){x}} {dx}\right) \\ $$$$={Im}\left(\:{I}_{\mathrm{4}{k}+\mathrm{3}} \:\right)\:=\frac{\left(\mathrm{4}{k}+\mathrm{3}\right)!}{\mathrm{2}^{\mathrm{2}{k}+\mathrm{2}} }\:{sin}\left(\frac{\left(\mathrm{4}{k}+\mathrm{4}\right)\pi}{\mathrm{4}}\right)\:=\frac{\left(\mathrm{4}{k}+\mathrm{3}\right)!}{\mathrm{2}^{\mathrm{2}{k}+\mathrm{2}} }{sin}\left(\left({k}+\mathrm{1}\right)\pi\right)\:=\mathrm{0} \\ $$
Answered by tanmay.chaudhury50@gmail.com last updated on 29/Oct/18
(1−i)x=−t [ t=(i−1)x] dx=((−dt)/((1−i))) ∫_0 ^∞ (t^n /((i−1)^n ))×e^(−t) ×((−dt)/((1−i))) (1/((i−1)^(n+1) ))∫_0 ^∞ e^(−t) t^(n+1−1) dt (1/((i−1)^(n+1) ))×⌈(n+1) (1/((i−1)^(n+1) ))×n! =(((−1)^(n+1) )/((1−i)^(n+1) ))×(((1+i)^(n+1) )/((1+i)^(n+1) ))×n! =(((−1)^(n+1) )/((2)^(n+1) ))×{(√2) ((1/( (√2) ))+i×(1/( (√2))))}^(n+1) ×n! =(((−1)^(n+1) )/2^((n+1)/2) )×{cos(π/4)+isin(π/4)}^(n+1) ×n! =(((−1)^(n+1) )/2^((n+1)/2) )×{cos(2kπ+(π/4))+isin(2kπ+(π/4))}^(n+1) ×n!
$$\left(\mathrm{1}−{i}\right){x}=−{t}\:\:\:\:\:\:\:\:\:\:\:\left[\:\:{t}=\left({i}−\mathrm{1}\right){x}\right] \\ $$$${dx}=\frac{−{dt}}{\left(\mathrm{1}−{i}\right)} \\ $$$$\int_{\mathrm{0}} ^{\infty} \frac{{t}^{{n}} }{\left({i}−\mathrm{1}\right)^{{n}} }×{e}^{−{t}} \:×\frac{−{dt}}{\left(\mathrm{1}−{i}\right)} \\ $$$$\frac{\mathrm{1}}{\left({i}−\mathrm{1}\right)^{{n}+\mathrm{1}} }\int_{\mathrm{0}} ^{\infty} {e}^{−{t}} {t}^{{n}+\mathrm{1}−\mathrm{1}} {dt} \\ $$$$\frac{\mathrm{1}}{\left({i}−\mathrm{1}\right)^{{n}+\mathrm{1}} }×\lceil\left({n}+\mathrm{1}\right) \\ $$$$\frac{\mathrm{1}}{\left({i}−\mathrm{1}\right)^{{n}+\mathrm{1}} }×{n}! \\ $$$$=\frac{\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} }{\left(\mathrm{1}−{i}\right)^{{n}+\mathrm{1}} }×\frac{\left(\mathrm{1}+{i}\right)^{{n}+\mathrm{1}} }{\left(\mathrm{1}+{i}\right)^{{n}+\mathrm{1}} }×{n}! \\ $$$$=\frac{\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} }{\left(\mathrm{2}\right)^{{n}+\mathrm{1}} }×\left\{\sqrt{\mathrm{2}}\:\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}\:}+{i}×\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\right)\right\}^{{n}+\mathrm{1}} ×{n}! \\ $$$$=\frac{\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} }{\mathrm{2}^{\frac{{n}+\mathrm{1}}{\mathrm{2}}} }×\left\{{cos}\frac{\pi}{\mathrm{4}}+{isin}\frac{\pi}{\mathrm{4}}\right\}^{{n}+\mathrm{1}} ×{n}! \\ $$$$=\frac{\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} }{\mathrm{2}^{\frac{{n}+\mathrm{1}}{\mathrm{2}}} }×\left\{{cos}\left(\mathrm{2}{k}\pi+\frac{\pi}{\mathrm{4}}\right)+{isin}\left(\mathrm{2}{k}\pi+\frac{\pi}{\mathrm{4}}\right)\right\}^{{n}+\mathrm{1}} ×{n}! \\ $$$$ \\ $$$$ \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *