Question Number 42994 by MJS last updated on 06/Sep/18
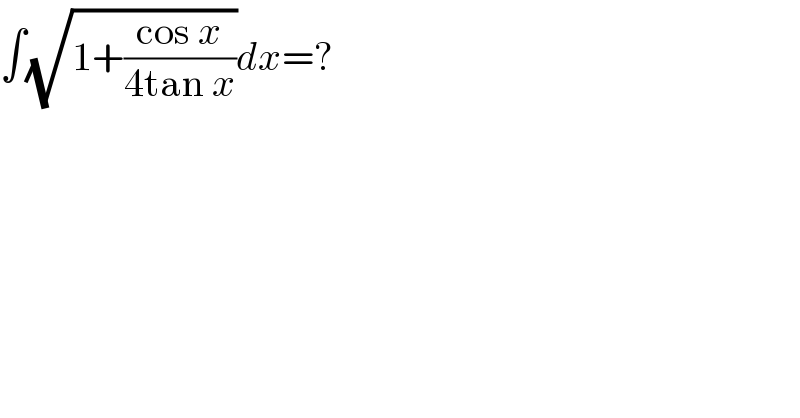
$$\int\sqrt{\mathrm{1}+\frac{\mathrm{cos}\:{x}}{\mathrm{4tan}\:{x}}}{dx}=? \\ $$
Commented by tanmay.chaudhury50@gmail.com last updated on 06/Sep/18
$${this}\:{problem}\:{snatched}\:{the}\:{sleep}\:{and}\:{mind}\:{hovering} \\ $$$${to}\:{get}\:{the}\:{answer}… \\ $$
Commented by mondodotto@gmail.com last updated on 07/Sep/18
$$\mathrm{please}\:\mathrm{someone}\:\mathrm{solve}\:\mathrm{this}\:\mathrm{problem} \\ $$
Commented by rahul 19 last updated on 07/Sep/18

$$\mathrm{If}\:\mathrm{i}'\mathrm{m}\:\mathrm{not}\:\mathrm{wrong}\:,\:\mathrm{here}\:\mathrm{incomplete}\: \\ $$$$\mathrm{elliptic}\:\mathrm{integral}\:\mathrm{of}\:\mathrm{second}\:\mathrm{kind}\:\mathrm{will}\:\mathrm{form}! \\ $$$$\left(\mathrm{i}\:\mathrm{asked}\:\mathrm{someone}!\right) \\ $$
Commented by MJS last updated on 07/Sep/18

$$\mathrm{I}\:\mathrm{think}\:\mathrm{so}\:\mathrm{too} \\ $$
Commented by maxmathsup by imad last updated on 08/Sep/18
$${i}\:{have}\:{tried}\:{this}\:{integral}\:{i}\:{think}\:{no}\:{way}\:{that}\:{lead}\:{to}\:{a}\:{elliptic}\:{intevral}\:{and}\:{if} \\ $$$${some}\:{one}\:{have}\:{something}\:{must}\:{post}…. \\ $$
