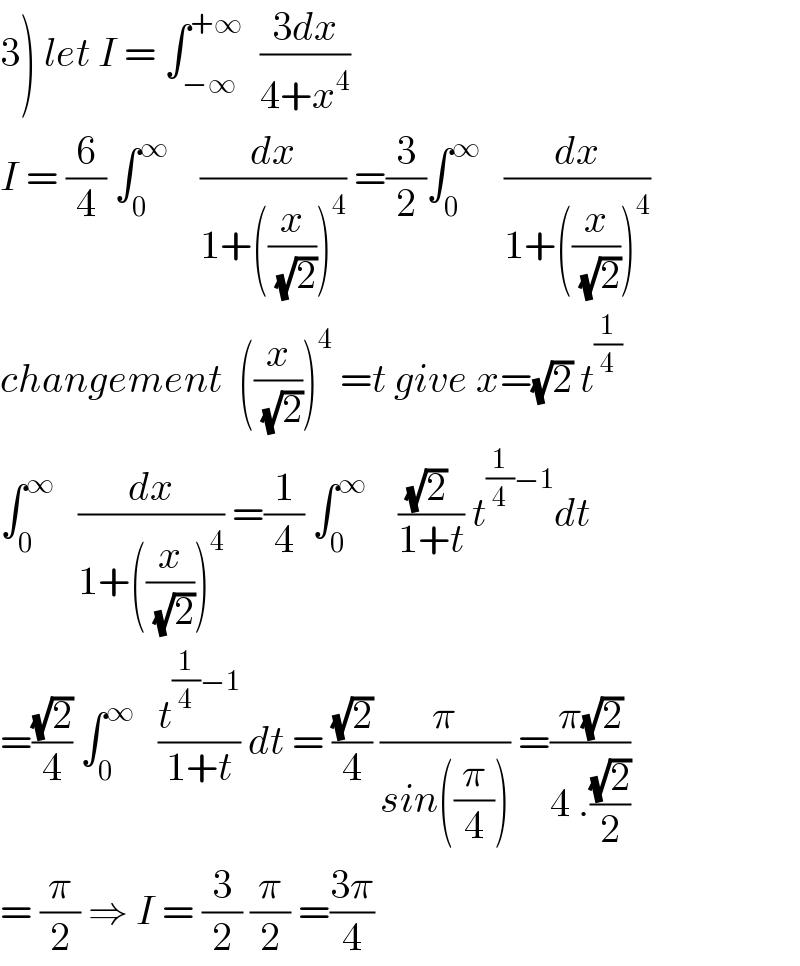Question Number 39967 by math khazana by abdo last updated on 14/Jul/18
$$\left.\mathrm{1}\right)\:{decompose}\:{inside}\:{C}\left({x}\right)\:{the}\:{fraction} \\ $$$${F}\left({x}\right)=\:\frac{\mathrm{3}}{\mathrm{4}+{x}^{\mathrm{4}} } \\ $$$$\left.\mathrm{2}\right)\:{find}\:\int_{−\infty} ^{+\infty} \:\:\:\frac{{dx}}{{x}−{z}}\:\:{with}\:{z}\:{from}\:{C} \\ $$$$\left.\mathrm{3}\right)\:{find}\:{the}\:{value}\:{of}\:\:\int_{−\infty} ^{+\infty} \:\:\:\frac{\mathrm{3}{dx}}{\mathrm{4}+{x}^{\mathrm{4}} }\:. \\ $$
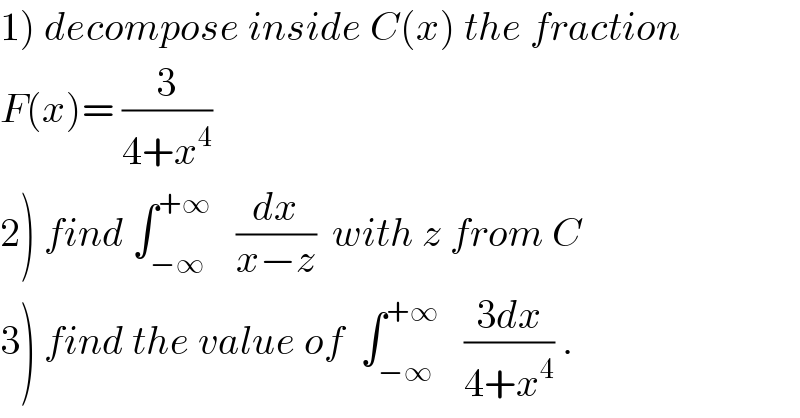
Commented by math khazana by abdo last updated on 15/Jul/18
$$\left.\mathrm{3}\left.\right)\:{for}\:{Q}\:\mathrm{3}\right)\:{i}\:{have}\:{used}\:{the}\:\:{formulae} \\ $$$$\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{t}^{{a}−\mathrm{1}} }{\mathrm{1}+{t}}\:{dt}\:=\:\frac{\pi}{{sin}\left(\pi{a}\right)}\:{if}\:\mathrm{0}<{a}<\mathrm{1}\:. \\ $$$$ \\ $$
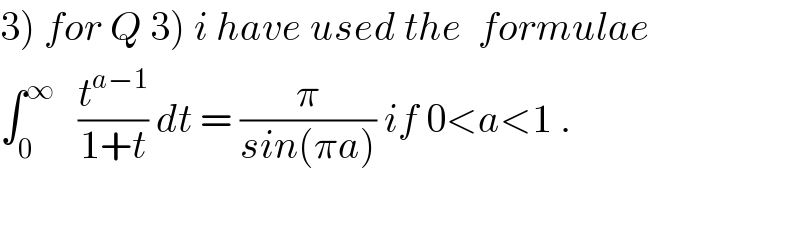
Commented by abdo mathsup 649 cc last updated on 15/Jul/18
$$\left.\mathrm{1}\right)\:{we}\:{have}\:{F}\left({x}\right)=\:\frac{\mathrm{3}}{\left(\sqrt{\mathrm{2}}\right)^{\mathrm{4}} \:+{x}^{\mathrm{4}} }\:=\frac{\mathrm{3}}{\left(\left(\mathrm{2}\right)^{\mathrm{2}} \:+\left({x}^{\mathrm{2}} \right)^{\mathrm{2}} \right.} \\ $$$$=\:\frac{\mathrm{3}}{\left({x}^{\mathrm{2}} \:+\mathrm{2}\right)^{\mathrm{2}} \:−\mathrm{4}{x}^{\mathrm{2}} }\:=\:\frac{\mathrm{3}}{\left({x}^{\mathrm{2}} \:−\mathrm{2}{x}\:+\mathrm{2}\right)\left({x}^{\mathrm{2}} \:+\mathrm{2}{x}\:+\mathrm{2}\right)} \\ $$$${roots}\:{of}\:{x}^{\mathrm{2}} \:−\mathrm{2}{x}\:+\mathrm{2}\: \\ $$$$\Delta^{'} \:=\:\mathrm{1}−\mathrm{2}\:=−\mathrm{1}=\left({i}\right)^{\mathrm{2}} \:\Rightarrow{x}_{\mathrm{0}} =\:\mathrm{1}\:+{i}\:=\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\:{e}^{\frac{{i}\pi}{\mathrm{4}}} \\ $$$${x}_{\mathrm{1}} =\mathrm{1}−{i}\:=\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\:{e}^{−\frac{{i}\pi}{\mathrm{4}}} \\ $$$${roots}\:{of}\:\:{x}^{\mathrm{2}} \:+\mathrm{2}{x}+\mathrm{2}\: \\ $$$$\Delta^{'} \:=\mathrm{1}−\mathrm{2}=−\mathrm{1}\:\Rightarrow\:{x}_{\mathrm{2}} =\:−\mathrm{1}\:+{i}\:=\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\:{e}^{\frac{{i}\mathrm{3}\pi}{\mathrm{4}}} \\ $$$${x}_{\mathrm{3}} =\:−\mathrm{1}−{i}\:=\:\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\:{e}^{−\frac{{i}\mathrm{3}\pi}{\mathrm{4}}} \:\:\Rightarrow \\ $$$${F}\left({x}\right)\:=\:\:\frac{\mathrm{3}}{\left({x}−{x}_{\mathrm{0}} \right)\left({x}−{x}_{\mathrm{1}} \right)\left({x}−{x}_{\mathrm{2}} \right)\left({x}−{x}_{\mathrm{3}} \right)} \\ $$$$=\:\frac{\mathrm{3}}{\left({x}−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}{e}^{\frac{{i}\pi}{\mathrm{4}}} \right)\left({x}−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}{e}^{−\frac{{i}\pi}{\mathrm{4}}} \right)\left({x}−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}{e}^{\frac{{i}\mathrm{3}\pi}{\mathrm{4}}} \right)\left({x}−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\:{e}^{−\frac{{i}\mathrm{3}\pi}{\mathrm{4}}} \right)} \\ $$$$=\:\frac{{a}}{{x}−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}{e}^{\frac{{i}\pi}{\mathrm{4}}} }\:+\:\frac{{b}}{{x}−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}{e}^{−\frac{{i}\pi}{\mathrm{4}}} }\:+\:\frac{{c}}{{x}−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}{e}^{\frac{{i}\mathrm{3}\pi}{\mathrm{4}}} }\:+\:\frac{{d}}{{x}−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}{e}^{−\frac{{i}\mathrm{3}\pi}{\mathrm{4}}} } \\ $$$${we}\:{have}\:\lambda_{{i}} =\:\frac{\mathrm{3}}{\mathrm{4}{x}_{{i}} ^{\mathrm{3}} }\:\Rightarrow \\ $$$${a}\:=\:\frac{\mathrm{3}}{\mathrm{4}\:{x}_{\mathrm{0}} ^{\mathrm{3}} }\:=\:\frac{\mathrm{3}}{\mathrm{4}\:.\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}\:{e}^{\frac{{i}\mathrm{3}\pi}{\mathrm{4}}} }\:=\mathrm{3}\:\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\:\:{e}^{−\frac{{i}\mathrm{3}\pi}{\mathrm{4}}} \\ $$$${b}\:=\:\frac{\mathrm{3}}{\mathrm{4}{x}_{\mathrm{1}} ^{\mathrm{3}} }\:\:=\:\frac{\mathrm{3}}{\mathrm{4}\:.\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}{e}^{−\frac{{i}\mathrm{3}\pi}{\mathrm{4}}} }\:=\frac{\mathrm{3}\sqrt{\mathrm{2}}}{\mathrm{2}}\:{e}^{\frac{{i}\mathrm{3}\pi}{\mathrm{4}}} \\ $$$${c}\:\:\:=\:\frac{\mathrm{3}}{\mathrm{4}\:{x}_{\mathrm{2}} ^{\mathrm{3}} }\:=\:\frac{\mathrm{3}}{\mathrm{4}\:.\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}{e}^{{i}\frac{\mathrm{9}\pi}{\mathrm{4}}} }\:=\:\frac{\mathrm{3}\sqrt{\mathrm{2}}}{\mathrm{2}}\:{e}^{−\frac{{i}\mathrm{9}\pi}{\mathrm{4}}} \:=\frac{\mathrm{3}\sqrt{\mathrm{2}}}{\mathrm{2}}\:{e}^{−\frac{{i}\pi}{\mathrm{4}}} \\ $$$${d}\:=\:\:\frac{\mathrm{3}}{\mathrm{4}\:{x}_{\mathrm{3}} ^{\mathrm{3}} }\:=\:\frac{\mathrm{3}}{\mathrm{4}\:.\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}{e}^{−\frac{{i}\mathrm{9}\pi}{\mathrm{4}}} }\:\:=\:\frac{\mathrm{3}\sqrt{\mathrm{2}}}{\mathrm{2}}\:{e}^{\frac{{i}\mathrm{9}\pi}{\mathrm{4}}} \:\:=\frac{\mathrm{3}\sqrt{\mathrm{2}}}{\mathrm{2}}\:{e}^{\frac{{i}\pi}{\mathrm{4}}} \\ $$$$ \\ $$
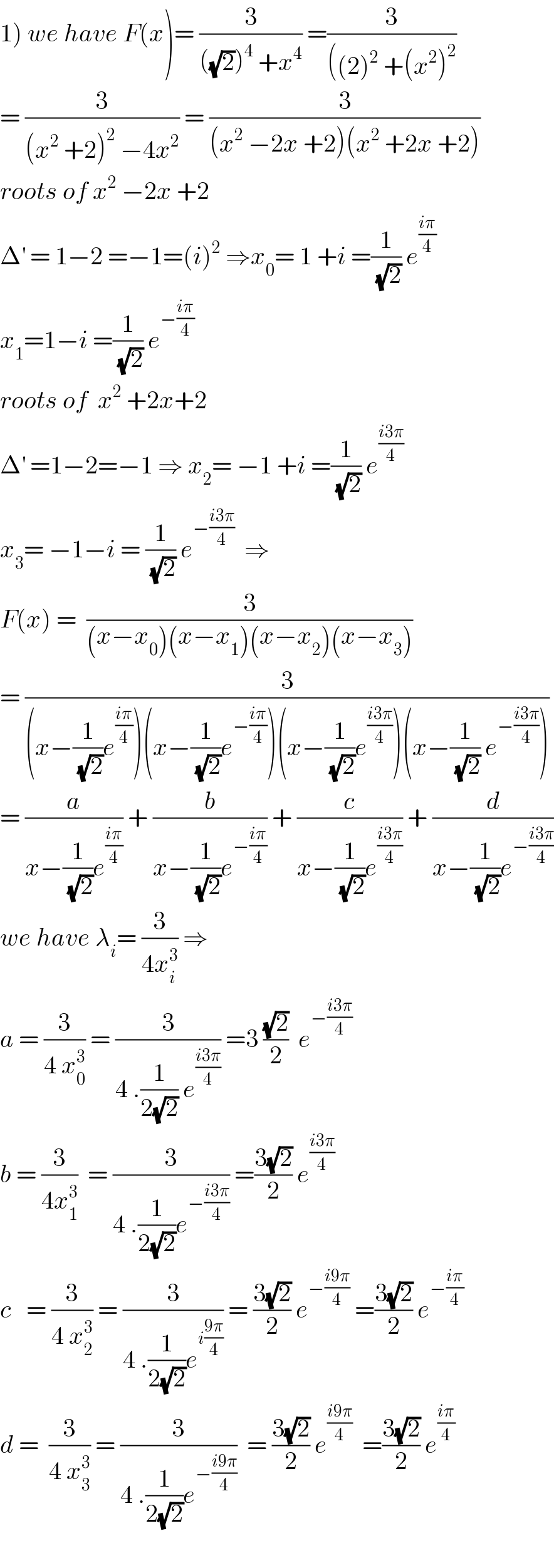
Commented by abdo mathsup 649 cc last updated on 15/Jul/18
![2) let put A(ξ) = ∫_(−ξ) ^(+ξ) (dx/(x−z)) we have lim_(ξ→+∞) A(ξ)= ∫_(−∞) ^(+∞) (dx/(x−z)) let z = α +iβ A(ξ) = ∫_(−ξ) ^ξ (dx/(x−α −iβ)) = ∫_(−ξ) ^(+ξ) ((x−α +iβ)/((x−α)^(2 ) +β^2 )) dx = ∫_(−ξ) ^ξ ((x−α)/((x−α)^2 +β^2 ))dx +iβ ∫_(−ξ) ^ξ (dx/((x−α)^2 +β^2 )) but ∫_(−ξ) ^ξ ((x−α)/((x−α)^2 +β^2 )) dx = (1/2)[ln∣(x−α)^2 +β^2 ∣]_(−ξ) ^(+ξ) = (1/2)ln((((ξ−α)^2 +β^2 )/((ξ+α)^2 +β^2 )))→0 when ξ→+∞ changement x−α = βt give ∫_(−ξ) ^ξ (dx/((x−α)^2 +β^2 )) = ∫_((−ξ−α)/β) ^((ξ−α)/β) (1/(β^2 (1+t^2 ))) β dt = (1/β) [ arctant]_((−ξ−α)/β) ^((ξ−α)/β) =(1/β) { arctan(((ξ−α)/β))+arctan(((ξ+α)/β))} ⇒iβ ∫_(−ξ) ^ξ (dx/((x−α)^2 +β^2 )) =i{ arctan(((ξ−α)/β)) +arctan(((ξ+α)/β))} so if β>0 arctan(((ξ −α)/β)) +arctan(((ξ +α)/β))_(ξ→+∞) →π if β<0 arctan(((ξ−α)/β)) +arctan(((ξ +α)/β))→−π so A(ξ) →iπ if β>0 and A(ξ)→−iπ if β<0 finally ∫_(−∞) ^(+∞) (dx/(x−z)) =iπ if Im(z)>0 and ∫_(−∞) ^(+∞) (dx/(x−z)) =−iπ if Im(z)<0 .](https://www.tinkutara.com/question/Q40000.png)
$$\left.\mathrm{2}\right)\:{let}\:{put}\:\:{A}\left(\xi\right)\:=\:\int_{−\xi} ^{+\xi} \:\:\:\:\frac{{dx}}{{x}−{z}}\:\:{we}\:{have}\: \\ $$$${lim}_{\xi\rightarrow+\infty} \:{A}\left(\xi\right)=\:\int_{−\infty} ^{+\infty} \:\:\frac{{dx}}{{x}−{z}}\:{let}\:{z}\:=\:\alpha\:+{i}\beta \\ $$$${A}\left(\xi\right)\:=\:\int_{−\xi} ^{\xi} \:\:\:\frac{{dx}}{{x}−\alpha\:−{i}\beta} \\ $$$$=\:\int_{−\xi} ^{+\xi} \:\:\:\:\:\frac{{x}−\alpha\:+{i}\beta}{\left({x}−\alpha\right)^{\mathrm{2}\:} \:+\beta^{\mathrm{2}} }\:{dx} \\ $$$$=\:\int_{−\xi} ^{\xi} \:\:\:\:\frac{{x}−\alpha}{\left({x}−\alpha\right)^{\mathrm{2}} \:+\beta^{\mathrm{2}} }{dx}\:\:+{i}\beta\:\int_{−\xi} ^{\xi} \:\:\:\frac{{dx}}{\left({x}−\alpha\right)^{\mathrm{2}} \:+\beta^{\mathrm{2}} }\:{but} \\ $$$$\int_{−\xi} ^{\xi} \:\:\:\frac{{x}−\alpha}{\left({x}−\alpha\right)^{\mathrm{2}} \:+\beta^{\mathrm{2}} }\:{dx}\:=\:\frac{\mathrm{1}}{\mathrm{2}}\left[{ln}\mid\left({x}−\alpha\right)^{\mathrm{2}} \:+\beta^{\mathrm{2}} \mid\right]_{−\xi} ^{+\xi} \\ $$$$=\:\frac{\mathrm{1}}{\mathrm{2}}{ln}\left(\frac{\left(\xi−\alpha\right)^{\mathrm{2}} \:+\beta^{\mathrm{2}} }{\left(\xi+\alpha\right)^{\mathrm{2}} \:+\beta^{\mathrm{2}} }\right)\rightarrow\mathrm{0}\:{when}\:\xi\rightarrow+\infty \\ $$$${changement}\:{x}−\alpha\:=\:\beta{t}\:{give} \\ $$$$\int_{−\xi} ^{\xi} \:\:\:\:\:\frac{{dx}}{\left({x}−\alpha\right)^{\mathrm{2}} \:+\beta^{\mathrm{2}} }\:=\:\int_{\frac{−\xi−\alpha}{\beta}} ^{\frac{\xi−\alpha}{\beta}} \:\:\:\:\frac{\mathrm{1}}{\beta^{\mathrm{2}} \left(\mathrm{1}+{t}^{\mathrm{2}} \right)}\:\beta\:{dt} \\ $$$$=\:\frac{\mathrm{1}}{\beta}\:\left[\:{arctant}\right]_{\frac{−\xi−\alpha}{\beta}} ^{\frac{\xi−\alpha}{\beta}} \:=\frac{\mathrm{1}}{\beta}\:\left\{\:{arctan}\left(\frac{\xi−\alpha}{\beta}\right)+{arctan}\left(\frac{\xi+\alpha}{\beta}\right)\right\} \\ $$$$\Rightarrow{i}\beta\:\int_{−\xi} ^{\xi} \:\:\:\frac{{dx}}{\left({x}−\alpha\right)^{\mathrm{2}} \:+\beta^{\mathrm{2}} }\:={i}\left\{\:{arctan}\left(\frac{\xi−\alpha}{\beta}\right)\:+{arctan}\left(\frac{\xi+\alpha}{\beta}\right)\right\} \\ $$$${so}\:{if}\:\beta>\mathrm{0}\:\:{arctan}\left(\frac{\xi\:−\alpha}{\beta}\right)\:+{arctan}\left(\frac{\xi\:+\alpha}{\beta}\right)_{\xi\rightarrow+\infty} \rightarrow\pi \\ $$$${if}\:\beta<\mathrm{0}\:\:{arctan}\left(\frac{\xi−\alpha}{\beta}\right)\:+{arctan}\left(\frac{\xi\:+\alpha}{\beta}\right)\rightarrow−\pi \\ $$$${so}\:{A}\left(\xi\right)\:\rightarrow{i}\pi\:{if}\:\beta>\mathrm{0}\:{and}\:{A}\left(\xi\right)\rightarrow−{i}\pi\:{if}\:\beta<\mathrm{0} \\ $$$${finally}\:\:\:\int_{−\infty} ^{+\infty} \:\:\:\frac{{dx}}{{x}−{z}}\:={i}\pi\:{if}\:{Im}\left({z}\right)>\mathrm{0}\:{and} \\ $$$$\int_{−\infty} ^{+\infty} \:\:\:\frac{{dx}}{{x}−{z}}\:=−{i}\pi\:{if}\:{Im}\left({z}\right)<\mathrm{0}\:. \\ $$
Commented by math khazana by abdo last updated on 15/Jul/18
$$\left.\mathrm{3}\right)\:{let}\:{I}\:=\:\int_{−\infty} ^{+\infty} \:\:\frac{\mathrm{3}{dx}}{\mathrm{4}+{x}^{\mathrm{4}} } \\ $$$${I}\:=\:\frac{\mathrm{6}}{\mathrm{4}}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\:\frac{{dx}}{\mathrm{1}+\left(\frac{{x}}{\:\sqrt{\mathrm{2}}}\right)^{\mathrm{4}} }\:=\frac{\mathrm{3}}{\mathrm{2}}\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{dx}}{\mathrm{1}+\left(\frac{{x}}{\:\sqrt{\mathrm{2}}}\right)^{\mathrm{4}} } \\ $$$${changement}\:\:\left(\frac{{x}}{\:\sqrt{\mathrm{2}}}\right)^{\mathrm{4}} \:={t}\:{give}\:{x}=\sqrt{\mathrm{2}}\:{t}^{\frac{\mathrm{1}}{\mathrm{4}}} \\ $$$$\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{dx}}{\mathrm{1}+\left(\frac{{x}}{\:\sqrt{\mathrm{2}}}\right)^{\mathrm{4}} }\:=\frac{\mathrm{1}}{\mathrm{4}}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\:\frac{\sqrt{\mathrm{2}}\:}{\mathrm{1}+{t}}\:{t}^{\frac{\mathrm{1}}{\mathrm{4}}−\mathrm{1}} {dt} \\ $$$$=\frac{\sqrt{\mathrm{2}}}{\mathrm{4}}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{t}^{\frac{\mathrm{1}}{\mathrm{4}}−\mathrm{1}} }{\mathrm{1}+{t}}\:{dt}\:=\:\frac{\sqrt{\mathrm{2}}}{\mathrm{4}}\:\frac{\pi}{{sin}\left(\frac{\pi}{\mathrm{4}}\right)}\:=\frac{\pi\sqrt{\mathrm{2}}}{\mathrm{4}\:.\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}} \\ $$$$=\:\frac{\pi}{\mathrm{2}}\:\Rightarrow\:{I}\:=\:\frac{\mathrm{3}}{\mathrm{2}}\:\frac{\pi}{\mathrm{2}}\:=\frac{\mathrm{3}\pi}{\mathrm{4}} \\ $$